MMUS

The initial use of magnetomotive force to induce vibrations in a biological medium and subsequently investigate the induced motion with ultrasound can be traced back to a study conducted by McAleavey et al. [ ] in 2003. This study primarily assessed the feasibility of using this method for the detection of prostate brachytherapy seeds. Nevertheless, the formal introduction of MMUS as a distinct technique is attributed to the work of Oh et al. [ ] in 2006. In this research, the identification of tissue-based macrophages was achieved using MNPs and MMUS. In this method, a sinusoidal magnetic field displaces MNP within tissue or tissue-mimicking phantoms, causing the surrounding tissue to also move. First, this tissue movement was detected using an ultrasound system to measure induced Doppler shifts and image it with power Doppler and M-mode imaging. Later, MMUS was adapted to pulsed magnetic fields [ , , ] and its combination with photoacoustic [ ] and photothermal therapy [ ] was explored. The enhanced pulsed MMUS method used similar cross-correlation techniques as in ultrasound elastography, where the received radiofrequency data under different magnetic force deformations were analyzed. This approach was also used for detecting the intracellular accumulation of MNPs [ , ].

In later works, the phase information of the displacement was included in MMUS [ , ] to enhance its imaging robustness. It was stated in [ , ] that the MMUS algorithm, which uses sinusoidal time-varying magnetic fields and relies entirely on the magnitude of displacement, has problems distinguishing natural tissue displacement outside the MNP area from displacement due to magnetomotive force. Evertsson et al. [ ] introduced a high-frequency phase-sensitive MMUS approach that analyzes the phase of the displacement. This phase-sensitive approach has become widely implemented in MMUS and will be used in this section to explain the working principle of MMUS. The other implementations of MMUS follow a similar principle.

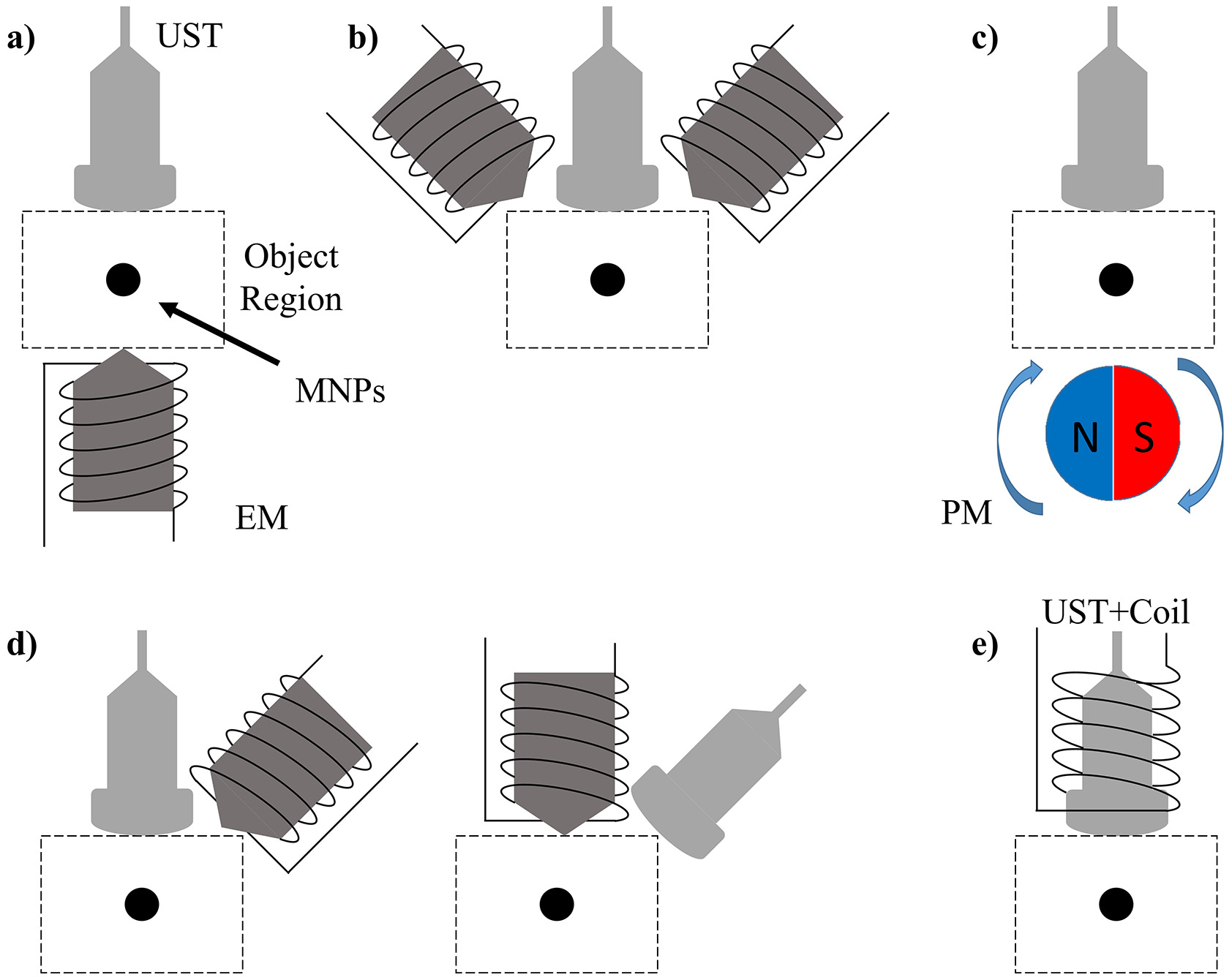
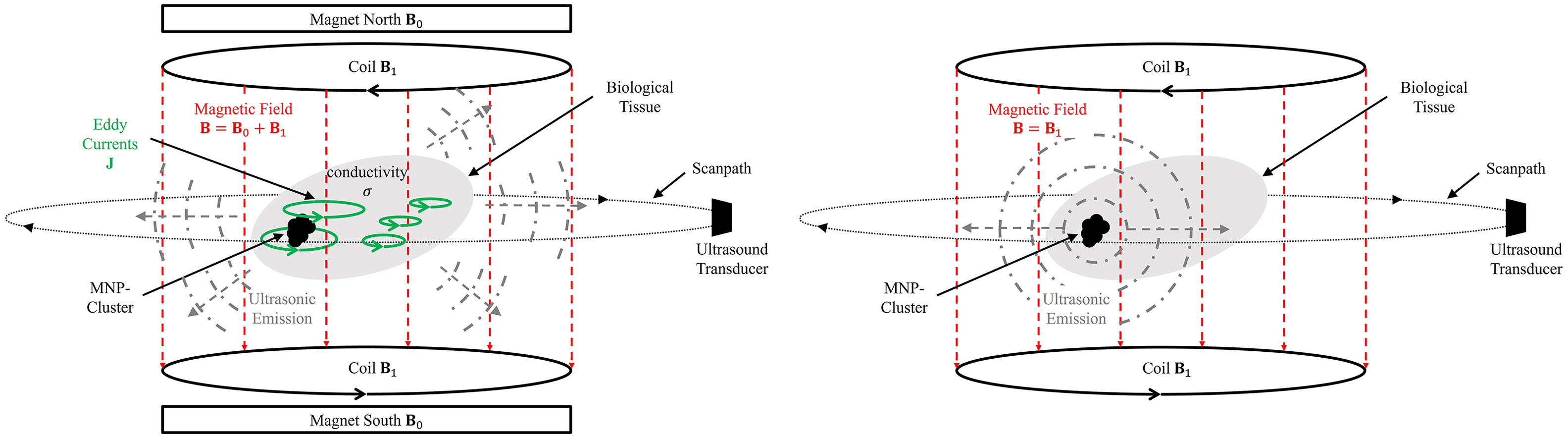
Evertsson et al. [ ] used an MMUS configuration, where the electromagnet and the ultrasound transducer were positioned on opposing sides (refer to Figure 1 a). The electromagnet emits a time-varying sinusoidal magnetic field B ( r , t ), that has the same frequency as the applied current of the electromagnet. As this magnetic field is applied to the imaging area, the magnetomotive force [ ]

Various magnetomotive ultrasound configurations used for positioning ultrasound transducer (UST), electromagnet (EM) and permanent magnet (PM). (a), (b) and (d) illustrate the electromagnet as coil with iron core, while (e) shows it without an iron core. The corresponding publications are listed in Table 4 .

This equation provides interesting properties of the acting magnetomotive force, for example, the oscillation frequency of the MNPs is twice that of the applied magnetic field. Moreover, the larger the magnetic field, the magnetic field gradient, or the magnetic susceptibility of the MNPs, the larger the magnetomotive force. In classical MMUS approaches, the next step would involve measuring the magnitude of the induced displacement with ultrasound-based methods and identifying areas where the displacement frequency corresponds to twice the applied frequency. However, it has been demonstrated by Evertsson et al. [ ] that relying solely on displacement measurement does not exclude displacement in unwanted areas. Instead, they showed that the region containing MNPs oscillates not only twice the excitation frequency (2· f ₀ ) but also with a phase shift of π compared with the surrounding tissue. Therefore, combining measurements of displacement and phase enhances the imaging of MNPs. Figure 2 from Evertsson et al. [ ] illustrates the results of this phase-sensitive MMUS approach ( Figure 2 d) compared with the classical approach ( Figure 2 b). Figure 2 a shows the total displacement and Figure 2 c the phase of the displacement.

Phase sensitive magnetomotive ultrasound [ ] (MMUS) results for different magnetic nanoparticle (MNP) concentrations within a polyvinyl alcohol (PVA) ultrasound phantom. In the B-mode image, three different regions are shown: I: MNPs with 0.6 mg/mL; II: ultrasound phantom; and III: MNPs with 0.45 mg/mL. The ultrasound transducer was placed above and the magnet below the phantom. A sinusoidal signal ( f = 4 Hz) was applied to the magnet. (a) Total induced displacement magnitude for all frequencies. (b) Displacement magnitude only for the 4 Hz excitation signal. (c) Corresponding phase. (d) Phase-sensitive MMUS result (used with the permission of the copyright holder; Copyright 2013 IEEE).

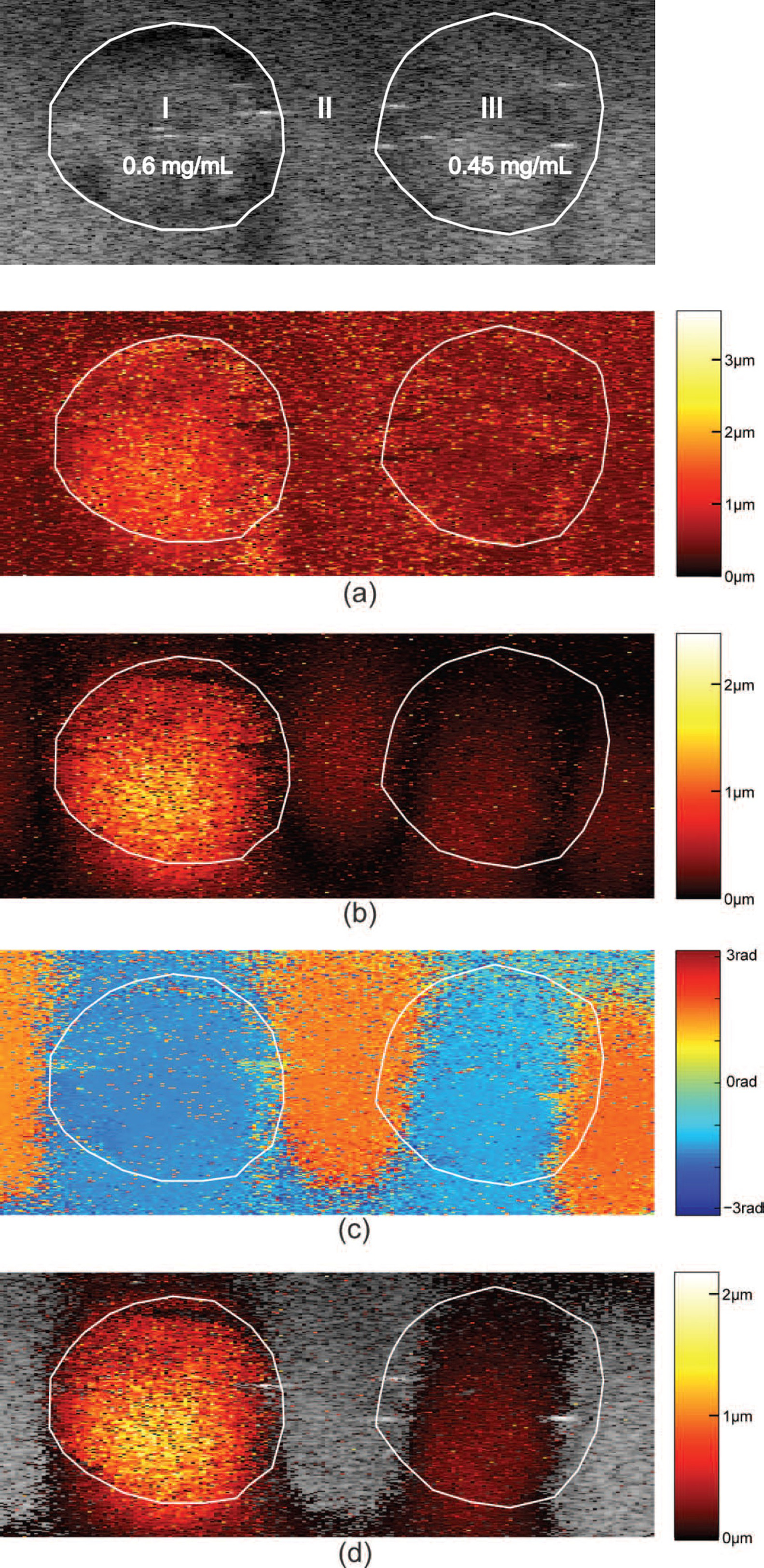
To evaluate the effectiveness of the phase-sensitive MMUS approach [ ], a polyvinyl alcohol ultrasound phantom was prepared with three distinct regions ( Figure 2 ). Region I contained an MNP inclusion with an iron content of 0.6 mg/mL, and region III had an iron content of 0.45 mg/mL. Region II represented the normal polyvinyl alcohol ultrasound phantom without MNPs. The ultrasound transducer was placed above the phantom, coupled with ultrasound gel, and the excitation electromagnet was positioned below the phantom.

Figure 2 a displays the magnitude of the total induced displacement for all frequencies, where no clear distinction between the MNPs area and the normal ultrasound phantom area is visible. When examining only the magnitude of displacement corresponding to the excitation frequency ( Figure 2 b), region I becomes visible prominently. However, it is important to note that all other regions in the phantom also exhibit displacement at this frequency. This lack of specificity makes it impossible to distinguish between MNPs with lower iron content (region III) and artifacts. In Figure 2 c, the phase of displacement for all frequencies is shown. Areas with and without MNPs have a phase difference of π. Evertsson et al. [ ] attributed the phase difference of π to the rigid boundaries of the phantom mold. Due to these rigid boundaries, the displacement of MNPs must be counteracted with displacement in the opposite direction, resulting in the opposite phase. By using the areas with a phase difference of π from Figure 2 c as a mask for the displacement in Figure 2 b, a phase-sensitive MMUS image is generated ( Figure 2 d). This image primarily contains displacement information from regions I and III, where the MNPs inclusions are located. This highlights the improvement over the original MMUS method, which solely relied on the magnitude of the displacement. A finite element simulation of a comparable setup validated the effectiveness of the proposed MMUS method and provided valuable insights into MMUS movement patterns [ ]. The simulated MMUS image closely matched the measured displacement, with differences primarily in the noise pattern and the magnitude of the actual displacement.

It is interesting to note that the measured displacements of different publications differ in some magnitude. In the case of [ , ], the displacement magnitude is typically around 3 μm or lower. In contrast, earlier publications [ ] demonstrated displacements exceeding 100 μm, while subsequent works [ ] reported results in the tens of μm range. The discrepancy in displacement magnitude can be attributed to the different ultrasound phantoms used, the strength of the magnetic field applied, the MNP iron content, and the physical properties of the MNPs. For example, Levy et al. [ ] demonstrated the influence of the elastic parameters of the ultrasound phantom on MMUS displacement, where higher elastic moduli results in lower displacements. Additional approaches to enhance induced displacement include modifying the properties of the MNPs, such as using tailored MNPs [ ] or pre-magnetizing the MNPs [ ]. Furthermore, improvements can be achieved through coded excitation [ , ] to enhance the signal-to-noise ratio and robustness against interference [ ], as well as using more accurate displacement estimators [ , ]. It is worth mentioning that MMUS can also be combined with thermal strain imaging (see the Ultrasound-guided MH section) for imaging MH [ ].

Furthermore, it has been demonstrated that MMUS can be performed in near real-time, achieving a frame rate of 3.46 Hz [ ]. This represents a significant advancement compared with other MMUS implementations that require several seconds to minutes to compute the displacement map. There are additional approaches to further enhance the real-time capabilities of MMUS, such as using recursive estimators [ ] or implementing it online on an ultrasound system [ ].

The configuration used for MMUS, particularly the positioning of the magnet and ultrasound transducer, is crucial for its clinical applications. The original configuration ( Figure 1 a) with the magnet and ultrasound transducer on opposite sides of the imaging region presents practical challenges, such as large distances between them and limited space in operation rooms. Alternative configurations have been explored, as depicted in Figure 1 . These configurations have been extensively tested in ex vivo and in vivo experiments. The first MMUS imaging on living rats, focusing on tumor detection, was conducted by Mehrmohammadi et al. [ ]. In this study, a pulsed MMUS scheme was used to perform 3-D imaging of rat tumors, marking also the first instance of 3-D MMUS imaging. Furthermore, the development of a hybrid transducer (resembling the configuration shown in Figure 1 e) aimed at overcoming the spatial resolution limitations associated with alternating current biosusceptometry for MNP detection. This transducer has the capability to concurrently collect susceptometric and MMUS data [ ]. In vivo testing of this hybrid transducer demonstrated its potential for evaluating gastric function. Notably, the 3-D MMUS images produced by this transducer provided detailed anatomical information about the rat stomach [ ]. Ex vivo testing on rat lymph nodes was carried out [ , ], also in combination with other imaging modalities [ , ]. In vivo experiments were conducted on rats [ , ] and rabbits [ ]. Significantly, the study by Fink et al. [ ] marked the first instance of imaging the accumulation process of MNPs during MDT and using a setup in vivo where both the magnet and ultrasound transducer were positioned on the same side ( Figure 1 d), demonstrating the effectiveness of MMUS for MDT. However, the most advanced and nearly clinic-ready MMUS configuration involves a rectal probe with a revolving permanent magnet inside, surrounded by an ultrasound transducer array [ ] (patent pending [ ]). The rectal MMUS probe has been evaluated successfully on excised human tissue from a patient with rectal cancer, marking the first-ever human tissue trial of MMUS. A patient with advanced low rectal cancer was scheduled for rectal removal as part of tumor treatment. Before the surgery, 2 mL of MNPs solution (Magtrace, Endomag) was injected at three different sites: one directly into the tumor and two on either side of the tumor using an injection needle designed for hemorrhoids. After removal, the MMUS probe was able to image these three regions successfully. Furthermore, patents for MMUS were also granted or are pending [ , ].

Quantitative MMUS

Accurate quantification of the concentration of chemotherapeutic agents and MNPs at the tumor site is crucial for effective dose control and optimal treatment outcomes in MDT and MH. It is also important for molecular imaging to quantify where the particles accumulate. This information enables precise modulation of therapeutic interventions and ensures adequate heating in hyperthermia applications. The quantification of MNP distribution using MMUS was first explored in a simulation study [ ] in 2010. The study examined the design of magnetic coils to generate uniform magnetic fields for MMUS. They suggested that a uniform magnetic field would result in a displacement map proportional to the MNPs density if used in MMUS schemes.

Subsequently, research was conducted to quantify the effects of non-uniform magnetic fields and excitation parameters on magnetomotive displacement [ ]. Furthermore, Hossain et al. [ ] and Thapa et al. [ ] computed the normalized MNP density map by using the displacement excited by MNPs and the magnetic force in an inverse scheme. They successfully computed normalized MNPs density maps which are proportional to the MNP quantity, but only normalized and with halo-like artifacts.

In recent studies by Fink et al. [ , , ], a method was developed to quantify the absolute distribution of MNPs, rather than normalized maps. For this purpose, MMUS displacement maps were generated and measured using an ultrasound MNP phantom, in which displacements were induced by an external magnetic field. The displacement was measured using the frequency and phase sensitive approach from Evertsson et al. [ ], which results in u _{z ,meas} ( x, y ).

Fink et al. [ ] assumes a linear mechanical model, as the tissue displacement is in the low micrometer range. Navier’s equation is used to formulate the differential equation

An example of this result is depicted in Figure 3 . In this example, an MNPs concentration of 4 mg/mL, corresponding with 6.0·10 ¹⁴ particles per mL, was used. As shown, the result matches the actual MNPs distribution. With this approach, only a single simulation of the displacement map needs to be performed and compared with the real data. Thus, the method’s speed is limited solely by the simulation time and does not encounter convergence issues. These findings demonstrate the potential of MMUS for quantitative imaging of MNPs.

Result of inverse magnetomotive ultrasound to quantify the distribution of magnetic nanoparticles (MNP) within a polyvinyl-alcohol ultrasound phantom from [ ]. An MNP iron content of 4 mg/mL ≈ 6 × 10 ¹⁴ particles per mL was homogeneously distributed within the white dashed rectangle with a size of 20 mm × 20 mm. The inversion result closely matches the actual distribution (used with the permission of the copyright holder; Copyright 2021 IEEE).

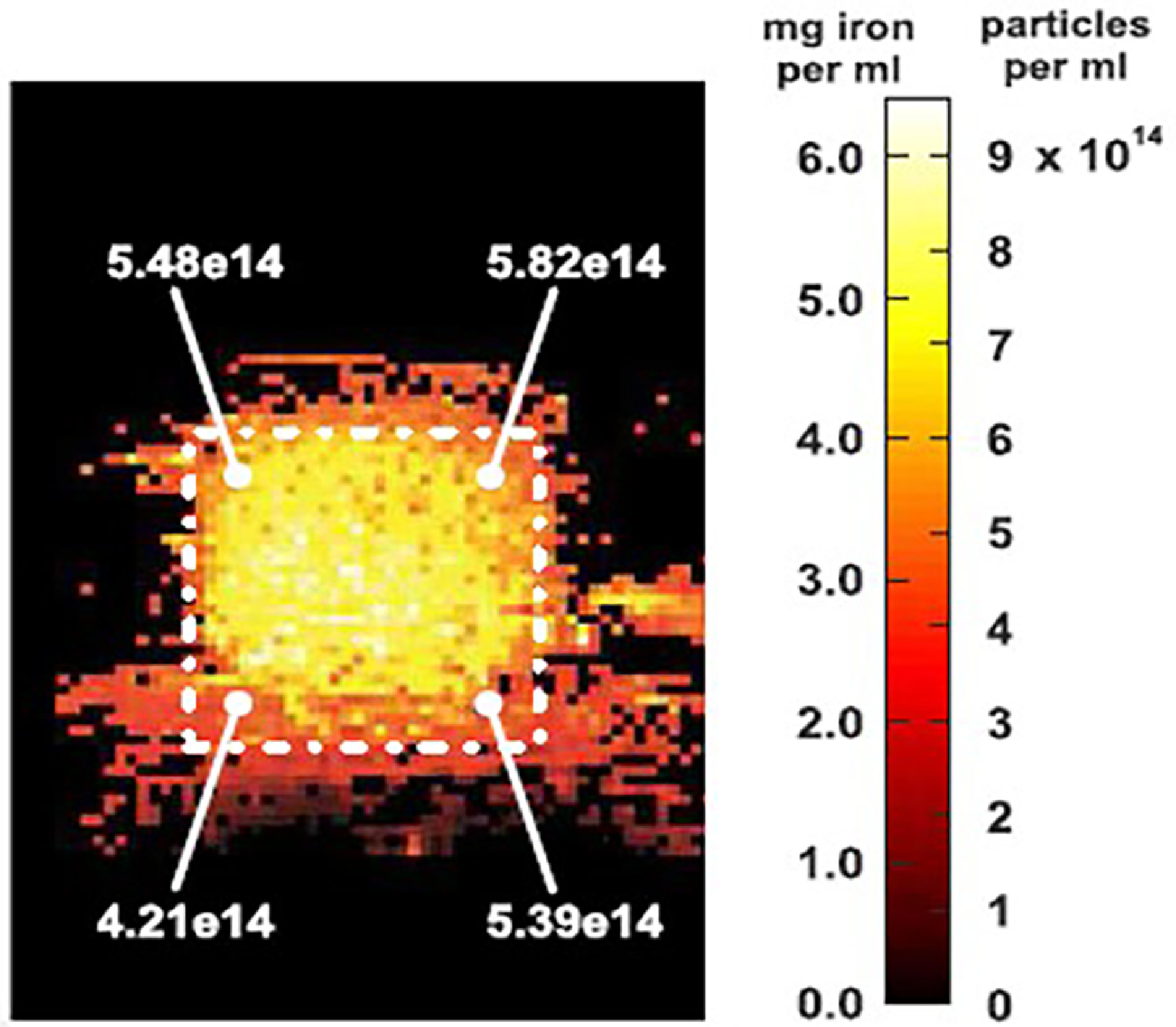
For practical implementation, precise mapping of the elastic parameters of surrounding tissue remains an area of ongoing research. Fink et al. [ ] demonstrated that inaccuracies in assessing mechanical parameters for simulations can significantly impact the results negatively. This can hinder practical implementation, as accurately quantifying the elastic parameters of biological tissue is complex. The linear and elastic assumptions used in mechanical simulations can pose problems in real scenarios, such as near blood vessels. It is also important to note that Sjöstrand et al. [ ] evaluated finite element simulations for MMUS displacement estimation, highlighting the significance of mechanical coupling.

Furthermore, the method requires additional evaluation, as it assumes a linear relationship between measured and simulated displacements with a single scaling parameter for the entire distribution. Although the presented method claims to be an inversion technique, it lacks a defined inversion problem and a feedback loop. Additionally, the 3-D distribution of MNPs is assumed to be uniform outside the imaging plane or that no particles exist outside this plane. These assumptions are inaccurate and can affect the results. For a detailed discussion on the assumptions, refer to the conclusion of Fink et al. [ ]. The method proposed by Fink et al. [ ] shows promise and warrants further evaluation, but its practicality in real-world applications needs to be demonstrated.

Magnetomotive elastography

Obtaining information about the structure and function of tissue through the assessment of their mechanical parameters is an essential part of medical diagnosis and therapy. Ultrasound elastographic imaging offers a non-invasive means of measuring these mechanical parameters. Two commonly used methods within this field are ultrasound strain elastography [ , ] and ultrasound shear wave elastography (SWE) [ , ]. These techniques induce tissue displacement to assess the stiffness or elasticity of tissue, with Young’s modulus ( E ) and shear modulus ( G ) representing the resistance to deformation under compression (longitudinal stress) and shear stress (transversal stress) respectively. Both methods are clinically available, and some studies have reported using these techniques to image the distribution of MNPs [ ]. However, the existing literature is limited, and the experiments have primarily been restricted to simple phantom studies.

A promising approach involves using the magnetically induced displacement of MNPs to image mechanical parameters. This technique primarily aims to image tissue properties, but can also map the distribution of MNPs. Various methods exist within this approach. MMUS elastography, which is similar to traditional ultrasound elastography, has not yet been tested on MNPs [ ]. Another variant is MMUS-based resonant acoustic spectroscopy, which estimates Young’s modulus by comparing the simulated and measured mechanical spectral responses [ ]. However, MMUS-SWE emerges as the most promising approach, having undergone more extensive evaluation in the literature compared with other methods [ , ].

In standard ultrasound SWE, a radiation force impulse is emitted from an ultrasound transducer to mechanically displace the tissue and induce shear wave propagation. As the speed of the longitudinal wave is higher than the speed of the shear wave (e.g., c _L = 1430…1579 m/s and c _S = 2…6 m/s) [ ], the shear wave propagation can be tracked with ultrafast plane wave imaging and used to reconstruct a shear wave speed map. The shear wave speed can then be used to estimate the shear modulus and Young’s modulus.

In MMUS-SWE, MNPs and the magnetic gradient force ( eqn [1 ]) are used. Under the influence of this magnetic gradient force, induced by a time-limited harmonic [ , ] or a pulsed signal [ ], shear waves are excited and propagate. This propagation can be tracked using the pulse-echo method from Deng et al. [ ] or similar techniques to compute shear wave speed ( c _S ), which is linked to shear modulus G

Results of a phantom experiment to excite and track shear waves using magnetic excitation from Lin et al. [ ]. (a) Scanning electron microscope image of the used MNPs. (b) Their magnetization curve. (c) B-mode image of the phantom, where the MNPs inclusion is marked. (d) Shear wave propagation over time (vibration speed). (e) A localization image of the MNPs distribution using MMUS-SWE. (f) Shear wave speed estimation using linear regression. (g) Overlay of the MNP localization, the B-mode image and the 2-D shear wave speed map around the MNPs (used with the permission of the copyright holder; Copyright 2022 IEEE).

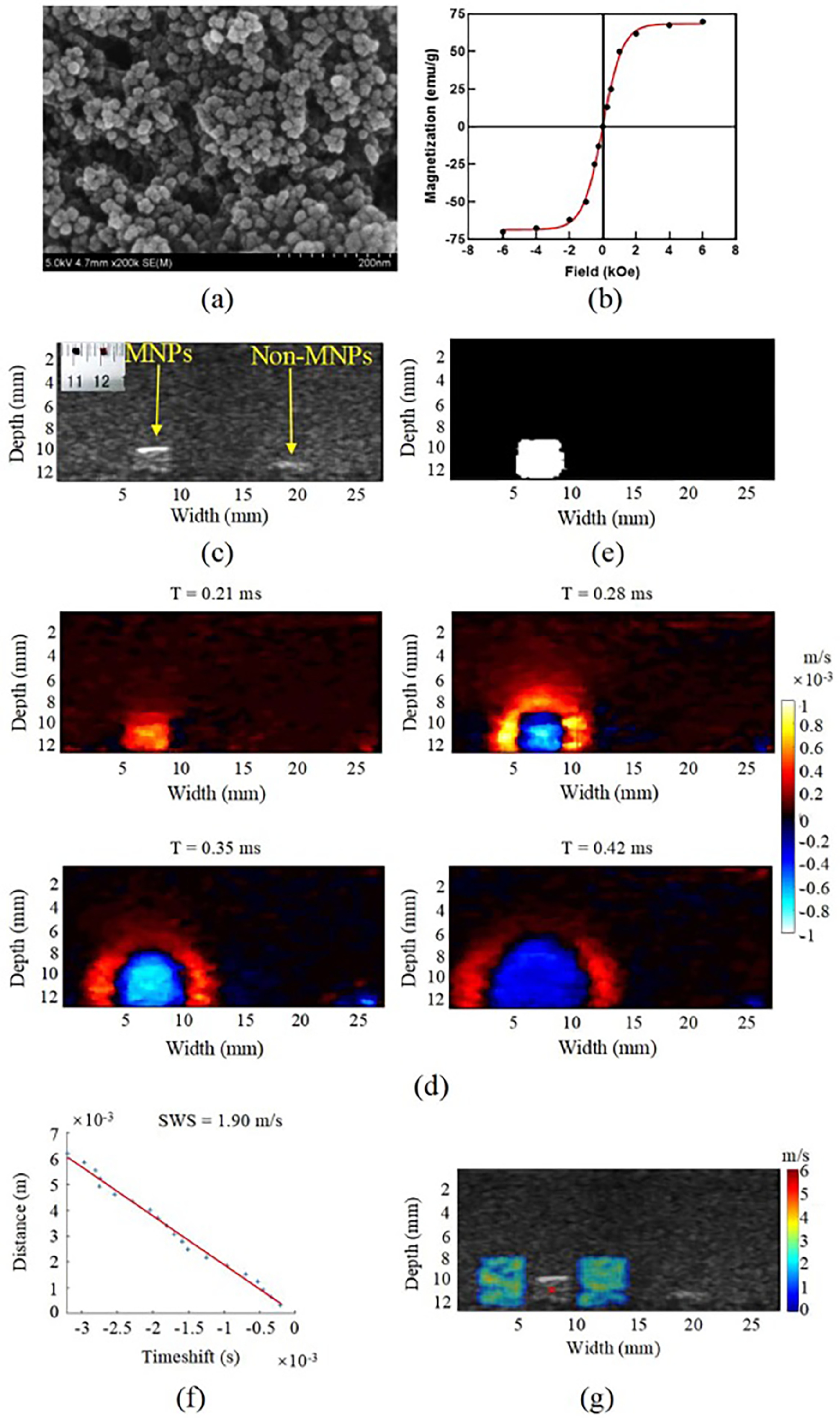
The presented phantom experiment in Figure 4 used MNPs (10-30 nm, MACKLIN, China) in a gelatin mixture. A phantom with an MNPs inclusion and a non-MNPs inclusion ( α -Fe ₂ O ₃ , 10-30 nm) (Aladdin Scientific, Shanghai, China) was prepared. A magnetic field with a maximum magnetic flux density of 1.4 T and a pulse width of 380 μs was applied. The corresponding shear wave propagation of the phantom experiment is presented in Figure 4 , showing a B-mode image of the phantom with MNPs ( Figure 4 c) and the corresponding shear wave propagation ( Figure 4 d). The authors of [ ] also computed an MNP localization image using MMUS-SWE, where the maximum displacement of the first 20 frames and an 80% threshold is used to generate a binary mask, with the center representing the MNPs position. This localization image is presented in Figure 4 e.

The phantom experiments of [ ] and the in vitro experiments of [ ] demonstrated the potential to simultaneously image the MNPs distribution and the shear wave speed. Using the shear wave speed allows for estimations of the elastic parameters. However, in vivo experiments are needed to show the practicability of this method.

MNPs in magnetomotive imaging

Magnetomotive imaging represents one of the most advanced and mature techniques for imaging MNPs in ultrasound-based methods. MMUS, quantitative MMUS, and magnetomotive SWE are based on the magnetic displacement of MNPs as described by eqn (1 ). Beyond the magnetic gradient force, the viscous drag force and the elastic restoring force are also significant, influenced by the tissue environment and the mechanical coupling of MNPs to the tissue [ ]. Table 7 lists some MNPs used in magnetomotive imaging, categorized by the corresponding imaging method. The listed studies have used various MNPs with differing iron core materials, coatings, sizes and magnetic properties.

The experimental environment (phantom, ex vivo or in vivo ) significantly influences the resulting displacement, which must be considered when using magnetomotive imaging methods. It has been shown that MMUS can effectively image MNP distributions in phantoms with iron concentrations as low as 0.04 mg Fe/mL [ ]. However, this strongly depends on the experimental setup. For instance, Evertsson et al. [ ] reported that an iron concentration of 0.3 mg Fe/mL was insufficient for MMUS, attributing this to diamagnetic effects and mechanical parameters of the phantom which can result in a threshold phenomena. These variations highlight the importance of MNP type, elastic environment, and magnetic field used.

MMUS has been used successfully for imaging sentinel lymph nodes in rats with iron concentrations of 0.1, 0.2 and 0.3 mg Fe/mL after the subcutaneous injection of 0.1 mL MNP solution. Additionally, an experiment in human tissue was conducted using clinically available MNPs (Magtrace, Endomag) with a concentration of 26 mg/mL [ ].

QMMUS has been tested with MNP concentrations of 1 to 4 mg/mL, and MMUS-SWE with concentrations of 20 to 60 mg/mL, primarily in phantom studies. Among these methods, MMUS has shown the most promising results, while QMMUS and MMUS-SWE also demonstrated potential for diverse MNPs and practical iron concentrations. QMMUS and MMUS-SWE have to be tested and evaluated with lower iron concentration levels and in animal experiments to demonstrate their potential in clinically relevant scenarios.

MAT-MI

The first method is MAT-MI, introduced by Xu and He [ ] in 2005. In this section, a rough idea of MAT-MI will be sketched. For more in-depth details, refer to the publications listed in Table 5 .

A schematic illustration of the setup for MAT-MI is shown in Figure 5 . As shown in Figure 5 , a conductive sample (biological tissue with MNPs inclusion) is placed in a magnetic field. This field consists of a static part B ₀ ( r ) from permanent magnets and an alternating part B ₁ ( r , t ) from magnetic coils. Due to the alternating part of the magnetic field, a spatially varying electric field

(a) Schematic setup for Magnetoacoustic Tomography with Magnetic Induction (MAT-MI). (b) Schematic setup for both magnetoacoustic tomography/detection (MAT/MAD) and magnetoacoustic concentration tomography with magnetic induction (MACT-MI). The difference between these two setups is the missing magnet for the static magnetic field. In MAT-MI, acoustic signals are generated due to induced eddy currents in conductive objects and the resulting Lorentz force. MAT and MACT-MI are made specifically for MNP imaging. The magnetic force of the pulsed magnetic field oscillates the MNPs in ultrasonic frequency range, which generates ultrasound waves.

The Lorentz force density is defined as the cross product between externally applied magnetic fields B and the action current density J . Using this, the magnetic Lorentz force density

Related posts:

Assessing Intensive Care Unit Acquired Weakness: An Observational Study Using Quantitative Ultrasound Shear Wave Elastography of the Rectus Femoris and Vastus Intermedius Transcranial MRI-guided Histotripsy Targeting Using MR-thermometry and MR-ARFI Sonopermeation With Size-sorted Microbubbles Synergistically Increases Survival and Enhances Tumor Apoptosis With L-DOX by Increasing Vascular Permeability and Perfusion in Neuroblastoma Xenografts Application of B+M-Mode Ultrasound in Evaluating Dysphagia in Elderly Stroke Patients Acoustic Droplet Vaporization Efficiency and Oxygen Scavenging in Whole Blood Ultrasound Strain Imaging for Characterizing Atherosclerotic Plaque in the Carotid Arteries of Asymptomatic Subjects

Stay updated, free articles. Join our Telegram channel

Join