Effects of Different Gas Cores on the Ambient Pressure Sensitivity of the Subharmonic Response of SonoVue

Abstract

Objective

Subharmonic Aided Pressure Estimation (SHAPE) is a noninvasive technique for estimating organ-level blood pressure using the strong correlation between the subharmonic signal and ambient pressure. The compressible gas core of microbubbles enables them to generate linear and nonlinear acoustic responses when exposed to ultrasound. Here, the sulfur hexafluoride (SF ₆ ) gas core of SonoVue (known as Lumason in the United States), a clinical contrast agent, was exchanged with a perfluorobutane (PFB) core to investigate its effect on the SHAPE response.

Methods

Excitations of 25–700 kPa peak negative pressure (PNP) and 3 MHz transmission frequency were used to study in vitro the effects of overpressure changes ranging from 5 to 25 kPa (37–186 mm Hg).

Results

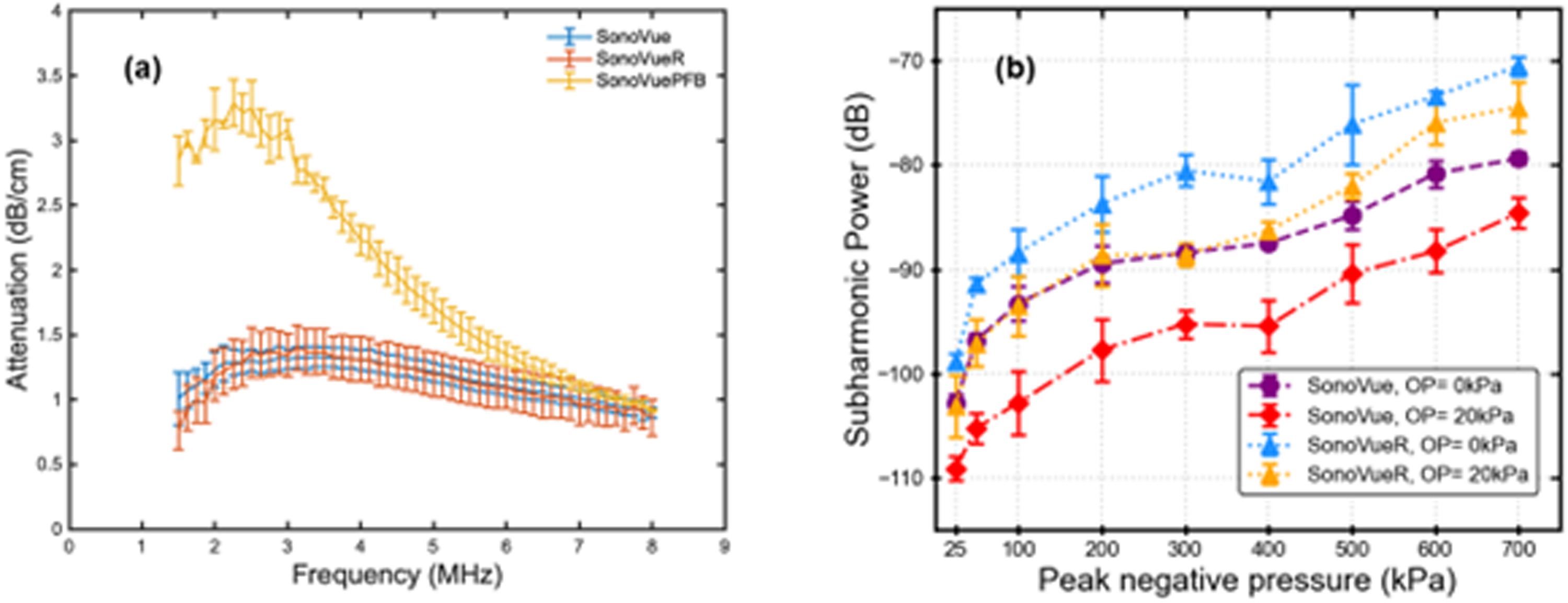
Unlike SonoVue with SF ₆ , at low PNPs (<400 kPa), SonoVue with a PFB gas core exhibited no subharmonic at the atmospheric pressure, but during pressurization, a stable subharmonic response (maximum of 25 dB at 100 kPa PNP and 20 kPa Overpressure) appeared. SonoVue with a PFB gas core showed an increase in subharmonics with overpressure at high PNPs (>400 kPa), which was not observed before in normal SonoVue or other lipid microbubbles. With negligible size distribution difference between these two microbubbles, these effects on subharmonic generation are likely due to the gas core, casting new light on the mechanism by which ambient overpressure affects subharmonic.

Conclusion

This study may inform future SHAPE technique developments.

Introduction

Microbubbles with a diameter ranging between 1 and 8 µm comprising a nanometer-thick shell of lipid, protein, or polymer encapsulating a gas core serve as contrast agents in ultrasound imaging [ ]. Thanks to their compressible gas core, these microspheres undergo volume oscillations when exposed to ultrasound. At large amplitude ultrasound excitations, bubbles scatter various nonlinear acoustic waves in addition to the linear fundamental oscillation at the same frequency of the excitation [ ]. The nonlinear response of bubbles has been utilized in various advanced therapeutic and diagnostic applications [ ]. Among those is the subharmonic aided pressure estimation (SHAPE) technique, which utilizes the strong correlation between ambient pressure and the subharmonic response of bubbles, happening at half the excitation frequency, to estimate the local fluid pressure inside blood vessels and organs noninvasively [ ]. In this study, we investigate the role of the gas core of a contrast agent on the subharmonic response and ambient pressure sensitivity of SonoVue (a.k.a. Lumason in the United States; Bracco Diagnostics, Princeton, NJ, USA) by replacing the SF ₆ gas core with perfluorobutane (PFB).

Acoustic excitation amplitudes larger than a threshold value are needed for bubbles to generate a subharmonic response. While typically subharmonic generation shows three stages of occurrence (at threshold), growth, and saturation, with the latter two happening beyond an approximately 200–500 kPa peak negative pressure threshold [ ], some studies reported significantly lower thresholds at about 50 kPa [ ]. Most of them are for SonoVue agent with its SF ₆ gas core instead of bubbles with more common perfluorocarbon indicating a critical role of the gas core. Our previous study [ ] showed that SonoVue bubbles produce subharmonic at PNPs as low as 25 kPa followed by two separate phases of growth and saturation with increasing PNP. A similar subharmonic profile was reported by Xu et al. [ ] as well, whereas a tri-phasic behavior has been reported in other studies [ , ]. The subharmonic generated at low (≤400 kPa) and high (>400 kPa) excitations for SonoVue (named Type I and II, respectively) behaved differently. Note that the transition excitation (∼400 kPa PNP) observed for a 32-cycle, 3 MHz excitation might change for different excitation parameters.

The sensitivity of subharmonic signals to ambient pressure was shown to differ depending on the excitation parameters ( Table 1 in Azami et al. [ ]). To address contradicting reports on subharmonic correlation—both positive and negative—with ambient pressure, in our previous study we studied the effect of an increase in hydrostatic pressure on the subharmonic amplitude over a wide range of PNPs covering all stages of subharmonic production [ ]. For a lipid bubble with a C ₄ F ₁₀ gas core with its typical three stages of subharmonic (occurrence, growth, saturation), adding overpressure resulted in a subharmonic decrease at the growth and saturation stage. The linear decrease in subharmonic with overpressure in the growth stage is the current standard method used in SHAPE because of both strong sensitivity and the high subharmonic amplitude, which is detectable with a clinical scanner [ ]. On the other hand, increasing overpressure triggered and increased subharmonic signal levels for PNP values for which the baseline subharmonic at atmospheric pressure is close to the noise level. This observation, which was in accordance with Frinking et al. [ ] partially addressed the contradictory trends in ambient pressure sensitivity measurements in the literature. Forcing bubbles into a buckling stage by elevated hydrostatic pressure rather than the acoustic pressure was responsible for the subharmonic increase at low excitation amplitudes. It is worth noting however that Mayer et al. [ ] did not detect any subharmonic activities with overpressure below threshold (at atmospheric pressure) strength with a clinical scanner, highlighting the need for further investigation. Note that previously we have shown through numerical investigation that subharmonic response can theoretically increase or decrease with overpressure depending on the ratio of the natural frequency of the microbubble, which is a function of the ambient pressure, to the excitation frequency [ ]. We showed that the subharmonic response attains a maximum when this ratio between frequencies is a critical value close to ∼0.5. Increasing overpressure increases the natural frequency and, thereby, its ratio to the excitation frequency, moving it either toward the critical value (increasing the subharmonic) or away from it (decreasing the subharmonic).

Table 1

Subharmonic Sensitivity Coefficients and R ²Values for SonoVuePFB and SonoVue in 0–20 kPa (0–150 mm Hg) Overpressure Interval

	SonoVuePFB		SonoVue
PNP ( kPa )	Sensitivity Coefficients (dB/ mm Hg)	R ²	Sensitivity Coefficients (dB/ mm Hg)	R ²
25	0.100	0.858	-0.048	0.719
50	0.146	0.964	-0.047	0.764
100	0.158	0.924	-0.070	0.901
200	0.149	0.930	-0.055	0.728
300	0.142	0.925	-0.049	0.617
400	0.111	0.865	-0.041	0.617
500	0.041	0.784	-0.055	0.711
600	0.033	0.615	-0.040	0.537
700	0.031	0.544	-0.029	0.654

For SonoVue with its Type I and Type II subharmonics, we found the SHAPE sensitivity trend was different from other bubbles with perfluorocarbon gas core [ ]. Specifically, while the Type II subharmonic response of SonoVue bubbles (i.e., at very high acoustic pressures) was typical of the one seen in the growth-saturation stage of other bubbles, the Type I subharmonic, at low PNPs, was present at atmospheric pressure only for SonoVue bubbles. During pressurization-depressurization cycles, it was present in earlier cycles with its amplitude decreasing with overpressure. For later cycles, it disappeared at atmospheric pressure to appear under overpressure, this behavior being identical to our previous in-house PFB bubbles [ ].

Kanbar et al. [ ] showed that bubbles with cores of air or SF ₆ with higher diffusivity than perfluorocarbons (PFC) exhibit subharmonic signals at 10 MHz acoustic excitations of 450 kPa, whereas bubbles with a PFC gas core produced subharmonic signals only after a 20–40 min delay. This observation was related to the quick outward diffusion of air or SF ₆ that creates a buckled shell and compression-only behavior that was associated with subharmonic generation [ ]. Similarly, Shekhar et al. [ ] showed that altering the diffusion rate by adjusting the air saturation level of the surrounding medium affects the subharmonic production, where bubbles in media with higher air saturation levels hence, lower diffusion rates, showed a delayed onset of subharmonic signal generation.

We think that the Type I subharmonic of SonoVue observed at unusually low excitation amplitudes is due to bubble shrinkage and buckling caused by outward SF ₆ diffusion. Here, we investigate the role of the gas core on the subharmonic response of SonoVue and its sensitivity to ambient pressure, by switching the SF ₆ core with a lower diffusivity C ₄ F ₁₀ (perfluorobutane [PFB]) gas. For the rest of the paper, we refer to SonoVue with PFB as SonoVuePFB, and the control case, reformulating by taking SF ₆ out and putting it back, as SonoVueR. We studied the acoustic response and subharmonic production at the atmospheric pressure and overpressures ranging from 5 to 25 kPa (37–186 mm Hg) over a PNP range of 25–700 kPa. Due to the large number of parameters, we restricted here to a single frequency which is representative of the resonance frequency of the polydisperse SonoVue agent as reported in the literature [ , , ]. The pressure sensitivity of subharmonic response at different frequencies was studied previously [ ] resulting in qualitatively similar behaviors.

Methods

SonoVue with PFB core

Before activating SonoVue bubbles as instructed in the product manual, the headspace of the vial containing SF ₆ was removed using a needle connected to the vacuum outlet. After vacuuming the headspace for two minutes (which created a -20 kPa pressure inside the vial), it was connected to a pressure-regulated PFB cylinder (FlouroMed L.P., Round Rock, TX, USA) and filled up until 5 kPa pressure was reached inside the vial. The next steps were based on the original activation protocol for SonoVue with SF ₆ in the head space. Briefly, 5 mL saline water was injected and the vial was shaken by hand for 20 seconds. The resultant solution containing SonoVuePFB was used within two hours of activation. To investigate the effects of SF ₆ removal, we also reformulated a control case of SonoVueR by drawing 5 mL SF ₆ out of the headspace of a SonoVue vial using an injection syringe and then immediately replacing it with more SF ₆ (making sure to reach 5 kPa pressure in the vial as in the case of SonoVuePFB).

Microbubble size measurement

To compare the size distribution of SonoVue, SonoVueR, and SonovuePFB, optical microscopy was used to measure the size distributions. In a similar setup and procedure to Azami et al. , a 10 µL aliquot of 11 times diluted bubble suspension was injected into a hemocytometer counting chamber. An in-house MATLAB (Mathworks, Natick, MA, USA) image processing program was used to count bubbles over 12 optical microscopy images taken at 40x magnification from random locations in the counting chamber. This procedure was replicated three times to calculate the average size distribution from a population of more than 4000 bubbles ( n = 3). The polydispersity of the size distributions was quantified by calculating the polydispersity index (PDI), which is the ratio of the standard deviation of the size distribution to the mean size.

Pressure-dependent scattering setup

The acoustic responses of various bubbles under varying static ambient pressures were measured using the pressurizing chamber and the acoustic measurement tank described in detail in our previous papers [ ]. For each experiment, 140 mL of 1000 (2000) times diluted SonoVue (SonoVuePFB) suspension was transferred into the pressurizing chamber before placing it inside an acoustic measurement tank filled with deionized water as shown in Figure 1 a. The difference in concentration was in response to higher attenuation by SonoVuePFB (See Fig. 2 a below) and based on preliminary experimental observations to obtain sufficient sensitivity for the SonoVuePFB scattering experiments. A calibrated 5 MHz focused transducer (V309, Olympus, Waltham, MA, USA) was driven by arbitrary function generator (DG1022, RIGOL, Portland, OR, USA) through a 55 dB linear power amplifier (A150, E&I, Rochester, NY, USA) to transmit acoustic excitations at 3 MHz frequency and 25–700 kPa peak negative pressure (PNP) into the pressurizing chamber. The sinusoidal ultrasound pulse consisted of 32 cycles and the repetition frequency was 100 Hz. The scattered acoustic response from the bubble suspension inside the pressure chamber was received by a broadband focused transducer (Y-102, Sonic Concepts, Bothell, WA, USA; -6 dB bandwidth of 10 kHz–15 MHz, 19 mm active diameter, and 50 mm geometric focal length). The received signal was filtered and amplified 20 dB by an ultrasonic pulser/receiver (Model 5800, Panametrics, Olympus, Waltham, MA, USA) and fed into an oscilloscope (MDO 3024, Tektronix, Beaverton, OR, USA). A MATLAB program was used to record twenty voltage time signals from the oscilloscope in sample mode. The averaged power spectrum was calculated offline using a Fast Fourier Transform with 1 V amplitude taken as the reference signal amplitude for calculations in dB.

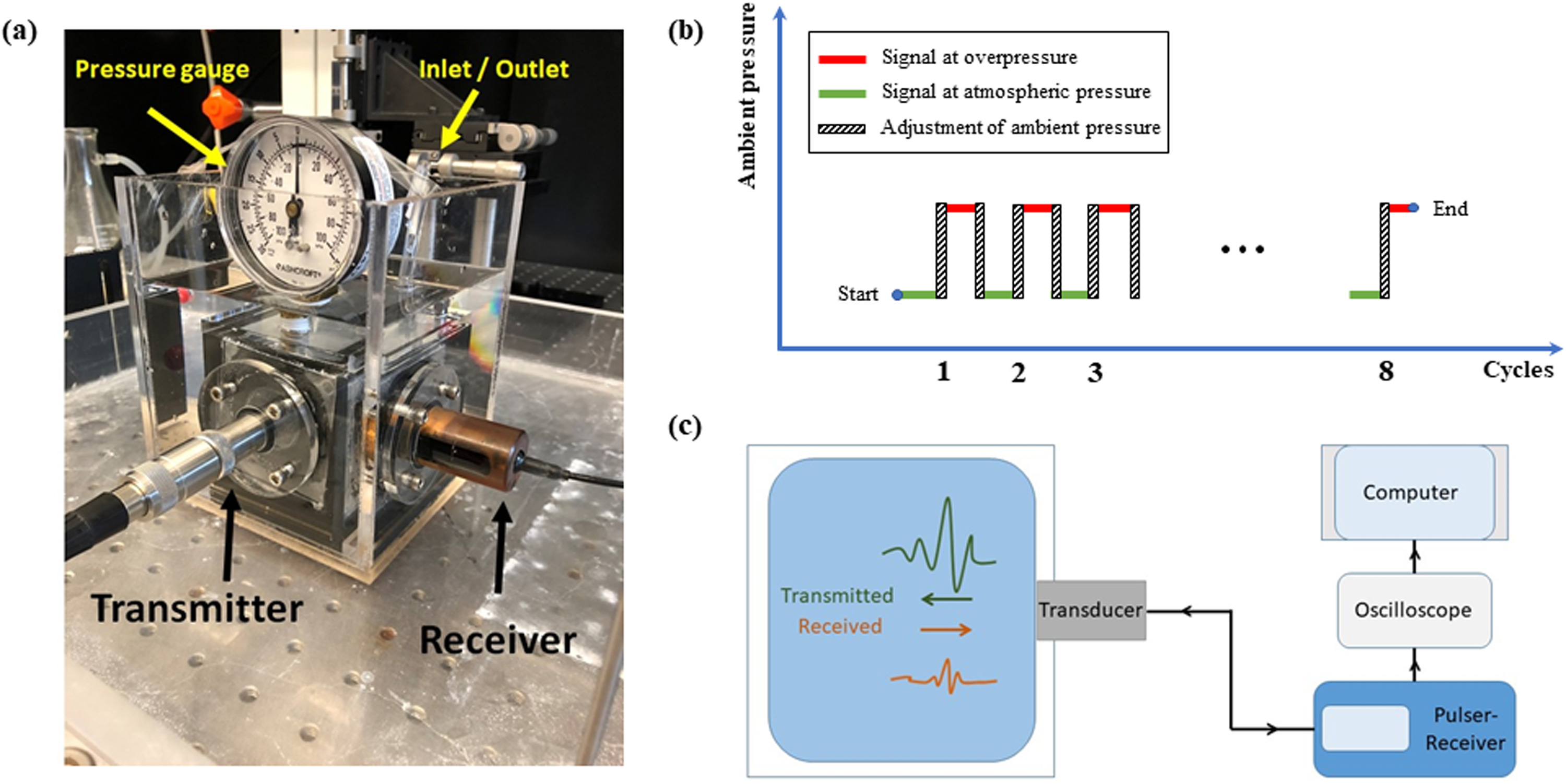
Experimental procedure for pressure-dependent scattering measurement

The acoustic response of the bubbles was measured under static pressurization-depressurization cycles as described previously [ ]. During each experiment, the pressure inside the chamber was increased to the same value and released eight times keeping the acoustic excitation constant. In each of the eight pressurization-depressurization cycles, the acoustic response was measured once immediately before pressurization (i.e., at atmospheric pressure) and once at the fixed overpressure, as described in Figure 1 b. For each parameter combination of 9 different PNPs (25–700 kPa) and 5 different ambient overpressures (5, 10, 15, 20, or 25 kPa), a fresh suspension of bubbles was used. Each measurement lasted for 210 ± 10 seconds. The experiments were replicated three times.

Attenuation setup

Attenuation was used to assess the effects of gas removal from the SonoVue vial during the gas exchange by comparing attenuation through SonoVue and SonoVueR following methods described in our previous publications [ , , ]. Briefly, 1 ml of microbubble suspension was diluted with 1950 ml of deionized (DI) water in a polycarbonate chamber. A magnetic stirrer was used to ensure the microbubbles were uniformly distributed during the measurements. The same pulser-receiver as in the scattering setup was used in a pulse-echo mode to transmit and receive pulses through a 5 MHz single element (-6 dB bandwidth: 2.8–6.4 MHz) unfocused transducer (V309, Olympus, Waltham, MA, USA) with a 100 Hz pulse repetition frequency (PRF). An external attenuator with an 11 dB setting was turned on to ensure negligible nonlinear dynamics. The oscilloscope received the signal with a 73 µs delay and a 1.5 GHz sampling rate in 64 sequence average mode. Signals were recorded as described in the previous section. Each measurement was repeated at least four times. Signals were recorded before and after the microbubble injection as shown in Figure 1 c. The fast Fourier transform (FFT) of the recorded signals was taken to calculate attenuation coefficients (dB/cm) as:

<SPAN role=presentation tabIndex=0 id=MathJax-Element-1-Frame class=MathJax style="POSITION: relative" data-mathml='α(ω)=10log(V2c(ω)/V2MB(ω))/d’>𝛼(𝜔)=10log(𝑉2𝑐(𝜔)/𝑉2𝑀𝐵(𝜔))/𝑑α(ω)=10log(V2c(ω)/V2MB(ω))/d
α(ω)=10log(V2c(ω)/V2MB(ω))/d

Only gold members can continue reading. Log In or Register to continue