Empirical Model of Focused Ultrasound-Mediated Treatment for Chemotherapy Delivery to Brain Tumors

Abstract

Focused ultrasound (FUS) has emerged as a transformative technique for enhancing drug delivery to brain tumors by temporarily and locally disrupting the blood-brain barrier (BBB). Despite significant progress in both pre-clinical and clinical research, a major challenge remains: the absence of a model that connects the properties of drug particles and FUS sonication parameters to therapeutic effectiveness. In this study, we introduce a novel empirical model that integrates key factors, including drug pharmacodynamics, microbubble kinetics for BBB disruption, intrabrain ultrasound signal propagation, and skull-thickness variations. The model defines a new sonication parameter that encapsulates ultrasound signal characteristics and predicts the concentration of therapeutic agents internalized or bound to DNA with an accuracy exceeding 82%. By employing data from previous pre-clinical studies, this model facilitates the development of precise sonication protocols tailored for clinical applications. These advancements represent a significant step toward personalized FUS-mediated treatments, bridging the gap between experimental research and patient-centered therapies.

Introduction

Central nervous system (CNS) diseases, including Alzheimer’s, Parkinson’s, multiple sclerosis, brain tumors, stroke and epilepsy, pose a significant global health burden affecting millions of people worldwide [ ]. For example, around 700,000 people are living with primary brain tumors in the US alone. These conditions are leading causes of death and disability, emphasizing the urgent need for innovative treatments and improved health care strategies.

One of the primary challenges in treating CNS diseases is the blood-brain barrier (BBB). The BBB is formed by endothelial cells in the brain’s capillaries and protects the brain from potentially harmful substances in the bloodstream while allowing essential nutrients to pass through. It effectively limits the delivery of chemotherapeutic agents to the tumor site. As a promising strategy to overcome this obstacle, microbubble-mediated focused ultrasound (MB-FUS) has emerged in the last two decades [ ]. Ultrasound-mediated therapies utilize the unique properties of ultrasound, such as its non-invasive tissue penetration and capacity to interact with biological structures. Ultrasound signals are produced by ultrasound transducers, and implantable transducers in single- and multi-element devices are also used as the ultrasound source [ ]. The backscattering of ultrasound waves from MBs generates mechanical disturbances that can temporarily disrupt the BBB, facilitating the passage of administered therapeutic agents through the vascular network to reach target tissues. This effect is enhanced when MBs oscillate in resonance with the ultrasound signal, referred to as cavitation, which can be classified in terms of linearity. In linear cavitation mode, the backscattered signal retains the same frequency as the original ultrasound signal emitted by the transducer. Linear cavitation typically occurs at a low mechanical index (MI <0.1) where the ultrasound pressure is relatively mild, causing the microbubbles (MBs) to oscillate without significant deformation. When the ultrasound pressure increases, the MBs oscillate non-linearly, producing backscattered signals that contain multiple harmonics (higher frequencies) of the original ultrasound signal. This indicates a more complex oscillation pattern of the MBs . At very high mechanical indexes the MBs can undergo inertial cavitation, characterized by a violent collapse that generates a broadband signal. While this collapse can induce strong mechanical effects and enhance drug delivery, it may also pose a risk of damaging surrounding tissues due to the intense mechanical forces involved.

Studies have demonstrated that ultrasound exposure, in combination with chemotherapeutic agents, can significantly improve treatment efficacy. For example, for gliomas, the most common primary tumors of the brain, an increase in therapeutic effectiveness of up to 3.5-fold and potential survival rate improvements by as much as 30% have been reported [ ]. However, the effective clinical translation of MB-FUS is hampered by several challenges, including determining the optimal sonication parameters (SPs), biological variability and overcoming translational hurdles [ ]. The BBB opening intensity and the pharmacokinetics of therapeutic agents are influenced by specific ultrasound signal characteristics, including acoustic pressure, burst length, and pulse repetition frequency and exposure time [ ]. Additionally, drug dosage and timing of administration are influential in the success of an MB-FUS-based treatment. Furthermore, safety concerns are paramount—excessive mechanical stress from MBs could result in adverse outcomes such as hemorrhage, edema or apoptosis in healthy tissues [ ].

To date, over 100 pre-clinical studies have examined BBB disruption and the safety of ultrasound exposure. These studies have assessed BBB opening by evaluating the ability of particles, such as magnetic resonance imaging (MRI) contrast agents gadolinium diethylene triamine penta-acetic acid (Gd-DTPA) or Evans blue, to cross the vessel walls. However, these approaches often lack comprehensive pharmacokinetic-pharmacodynamic (PK-PD) analyses. Additionally, ultrasound parametric studies conducted in some of these experiments have not yielded a standardized predictive model for the therapeutic response of focused ultrasound (FUS)-mediated treatments. Moreover, permeability measurements derived from MRI using Gd-DTPA as a contrast agent require adaptation for therapeutic agents of different sizes, which may affect their ability to traverse the BBB. In parallel to these pre-clinical investigations, several clinical trials (phase I/II) are currently evaluating FUS-mediated treatments for CNS disorders [ ]. However, these studies often lack treatment protocols tailored to individual patients and do not fully capture the potential therapeutic improvements resulting from BBB disruption followed by chemotherapy.

This study aims to develop a comprehensive mathematical framework that correlates SPs with drug pharmacodynamics. We define a novel SP in the linear cavitation regimen addressing various features of ultrasound exposure. It integrates all ultrasound factors into a unified factor considering the impact of each factor on BBB disruption and drug delivery. Our model predicts drug transport through the vessel wall following BBB disruption and its subsequent uptake by the tumor. This is done by employing ordinary differential equations (ODEs) to describe the dynamics of drug transport through the tumor vasculature and transmembrane transport, including DNA intercalation. Our approach is based on data collected from 22 previous pre-clinical experiments performing ultrasound parametric studies. Hence, our model could reduce the need for costly and challenging in vivo studies, paving a road toward improving current and future clinical trials by enabling more precise targeting of drug delivery and sparing patients ineffective treatments. As illustrated in Figure 1 , the proposed approach bridges the gap between pre-clinical and clinical studies by developing a comprehensive model that simultaneously incorporates drug characteristics and skull anatomy. This integrated platform enables personalized optimization of ultrasound application and drug administration, tailored to individual patient needs.

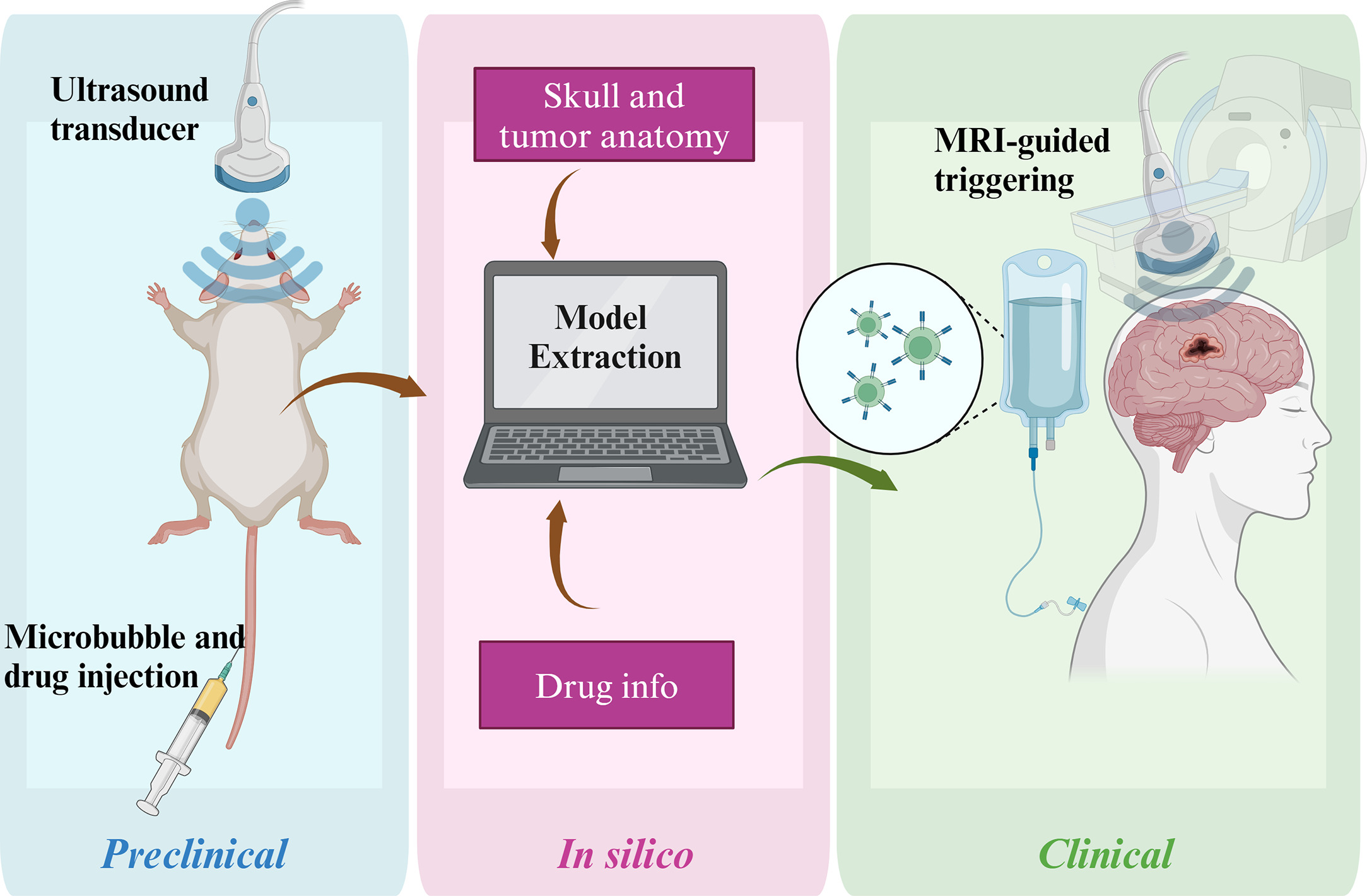
System model

As previously discussed, FUS-based treatment for CNS diseases depends on ultrasound exposure to facilitate BBB opening and the PK-PD of the administered drug. Key factors in modeling BBB opening include the behavior of the ultrasound signal as it propagates through the skull and brain tissue, along with the dosage and timing of MBs injection. BBB opening typically occurs within minutes, followed by a closure phase that can last for several hours. Regions with higher vascular density may respond faster due to increased MB concentration. The ultrasound signal’s characteristics influence these dynamics, the target locations in the brain and the kinetics of the MBs.

Impact of skull anatomy and tumor location

The effects of skull thickness and the ultrasound path from transducer to tumor mainly manifest in clinical studies and should be incorporated into the computational model. To tailor the model for individual patients, it is necessary to scale the SPs based on the estimated ultrasonic loss to the target. This propagation loss is characterized by the skull attenuation co-efficient 𝛼skl
α skl
and the intracranial path attenuation co-efficient 𝛼incl
α incl
. Consequently, the loss factor LF is given by eqn (1) :

𝐿𝐹𝑆𝐾=𝑒𝛼sklzskl+𝛼inclzincl,LFSK=eαsklzskl+αinclzincl,
LFSK=eαsklzskl+αinclzincl,

where z _skl denotes the skull thickness and z _incl represents the intracranial path length from the transducer to the tumor location. LF _SKcan be interpreted as the inverse of transcranial efficiency. Please note that using an implantable transducer eliminates the intraskull propagation of ultrasound and significantly reduces the signal attenuation.

Effect of MB administration

As previously discussed, MB administration is combined with FUS to enhance the effect of ultrasound signals on BBB disruption. The level of MB concentration at the treatment site during the sonication period is a critical factor in determining effective SPs. Here, we focused on the kinetics of MBs and their degradation after administration. Different types of MBs interact with ultrasound in unique ways depending on their size, oscillatory behavior and the pressure required to induce effects, which are influenced by shell thickness. However, these specific interactions were not addressed in our model.

Generally, MBs persist for a few minutes, and their concentration can be approximated by an exponential decay described by a first-order ODE. Therefore, we can define MB loss factor as eqn (2) :

𝐿𝐹MB=𝑒𝑡In(2)𝜏𝑀𝐵,LFMB=etIn(2)τMB,
LFMB=etIn(2)τMB,

where t represents the time after MB injection, and

𝜏𝑀𝐵τMB
τ M B
denotes the half-life of the MBs in the bloodstream, both in seconds. The validity of this proposed model will be discussed in the results section.

Proposed SP

To develop a practical model linking ultrasound signaling to the BBB transvascular rate function, we introduced a novel SP, given by eqn (3) :

𝑆𝑃=𝐸𝑇×𝑃𝑅𝐹×𝑃𝐷×𝑁𝑃𝑃𝑓√=𝐸𝑇×𝑃𝑅𝐹×𝑃𝐷×𝑀𝐼,SP=ET×PRF×PD×NPPf=ET×PRF×PD×MI,
SP=ET×PRF×PD×NPPf=ET×PRF×PD×MI,

where ET is the exposure time in seconds, PRF is the pulse repetition frequency in Hz, PD is the pulse duration in ms, NPP is the negative peak pressure in MPa and f is the ultrasound frequency in MHz. MI denotes the mechanical index measuring the instantaneous cavitation potential. SP represents the cumulative mechanical impact of ultrasound over the total exposure time. It combines the instantaneous mechanical cavitation potential with the temporal characteristics of the ultrasound signal (exposure time, pulse repetition frequency and pulse duration). Higher values of SP indicate a greater total mechanical bio-effect of ultrasound in our application.

In order to incorporate the loss effects as discussed in previous sub-sections, the SP given by the model should be scaled by loss factors LF _SKand LF _MBgiven by eqns (1) and ( 2 ), respectively, as eqn (4) :

𝑆𝑆𝑃=𝑆𝑃×𝐿𝐹𝑆𝐾×𝐿𝐹𝑀𝐵,SSP=SP×LFSK×LFMB,
SSP=SP×LFSK×LFMB,

where SSP is the scaled SP. Given the limited data set and the inherent variability due to differing experimental conditions, we aimed to establish a linear relationship between SP and the therapeutic response. This approach was chosen to mitigate the risk of overfitting. Additionally, a linear model facilitates a matrix-based formulation of the problem, which is advantageous for multi-target scenarios represented as a multiple-input and multiple-output (MIMO) system. Such a framework could be extended in future work to design optimized drug-delivery strategies across multiple targets.

In order to relate the therapeutic response to the SP, we first need to discuss the PK-PD aspects of the therapeutic agent.

Pharmacokinetics and pharmacodynamics of drug delivery through the BBB

Significant research has been conducted and mathematically implemented on the pharmacodynamics of the BBB and therapeutic agents [ ]. In this study, we focused on a single mechanism as a proof of concept to demonstrate the ability to predict drug delivery mediated by nuclear translocation using the transvascular rate function and interaction model in FUS-based treatment. Nevertheless, eqns (7) and ( 8 ) can be adapted or replaced with formulations describing the dynamics of other therapeutic agents, such as small-molecule inhibitors or monoclonal antibodies targeting specific cell surface receptors or antigens. We used sets of ODEs to describe the drug’s transport from the bloodstream to the cell nucleus, often assuming a single-target pathway, as shown below in eqns (5 – 8 ):

𝑑𝐶𝑣(𝑡)𝑑𝑡=𝐾tv(𝑡)(𝐶𝑣(𝑡)−𝐶𝑒(𝑡))−𝐾𝑣𝑑𝐶𝑣(𝑡)+𝐼(𝑡),dCv(t)dt=Ktv(t)(Cv(t)−Ce(t))−KdvCv(t)+I(t),
dCv(t)dt=Ktv(t)(Cv(t)−Ce(t))−KdvCv(t)+I(t),

𝑑𝐶𝑒(𝑡)𝑑𝑡=𝐾tv(𝑡)(𝐶𝑣(𝑡)−𝐶𝑒(𝑡))−𝑉𝐶𝑒(𝑡)𝑑𝑐𝐶𝑒(𝑡)+𝐾𝑒𝛼+𝑉𝐶𝑖(𝑡)𝑑𝑐𝐶𝑖(𝑡)+𝐾𝑖(1−𝛼)−𝐾𝑒𝑑𝐶𝑒(𝑡),dCe(t)dt=Ktv(t)(Cv(t)−Ce(t))−VCe(t)dcCe(t)+Keα+VCi(t)dcCi(t)+Ki(1−α)−KdeCe(t),
dCe(t)dt=Ktv(t)(Cv(t)−Ce(t))−VCe(t)dcCe(t)+Keα+VCi(t)dcCi(t)+Ki(1−α)−KdeCe(t),

𝑑𝐶𝑖(𝑡)𝑑𝑡=𝑉𝐶𝑒(𝑡)𝐶𝑒(𝑡)+𝐾𝑒𝛼−𝑉𝐶𝑖(𝑡)𝐶𝑖(𝑡)+𝐾𝑖(1−𝛼)−𝑉𝑏𝐶𝑖(𝑡),dCi(t)dt=VCe(t)Ce(t)+Keα−VCi(t)Ci(t)+Ki(1−α)−VbCi(t),
dCi(t)dt=VCe(t)Ce(t)+Keα−VCi(t)Ci(t)+Ki(1−α)−VbCi(t),

Only gold members can continue reading. Log In or Register to continue