Abstract
This chapter includes nuclear medicine instrumentation and quality control. The underlying principles, as well as the specifics of survey meters, well counters, single crystal probes, gamma cameras, SPECT/CT and PET/CT, are discussed. Recent advances of solid state and semiconductor detectors are also included. There are detailed quality control sections for both radiopharmaceuticals and equipment.
Keywords
survey meter, well counter, gamma camera, SPECT, PET/CT scanner, quality control, artifacts
Chapter Outline
Single-Photon Emission Computed Tomography (SPECT)
Positron Emission Tomography (PET)
Instrumentation Quality Control
Geiger-Mueller Counter
Geiger-Mueller (GM) counters are handheld, very sensitive, inexpensive survey instruments used primarily to detect small amounts of radioactive contamination. The detector is usually pancake shaped, although it may be cylindrical ( Fig. 2.1 ). The detector is gas filled and has a high applied voltage from the anode to the cathode. This causes one ionization to result in an “avalanche” of other electrons, allowing high efficiency for detection of even a single gamma ray. The avalanche of electrons takes some time to dissipate; as a result, “dead time” must occur before the next ionization can be detected. This precludes use of GM counters in high radiation fields. They are usually limited to exposure rates of up to about 100 mR (2.5 × 10 -5 C/kg)/hr. Most GM counters are equipped with a thin window that also allows detection of most beta rays. Very weak beta rays (such as those from tritium) cannot be detected.
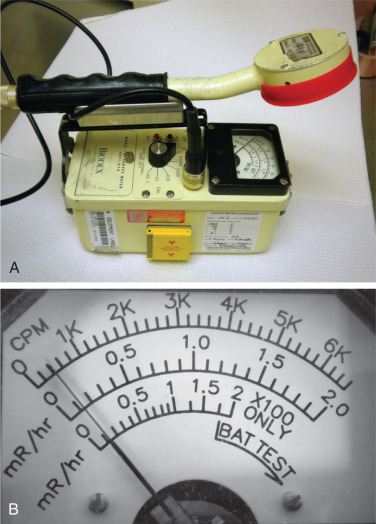
Advantages of GM counters include their durability, portability, and ability to detect many types of radiation with high sensitivity. Limitations include that they can neither differentiate which type of radiation is being detected nor determine the exact energy of the detected radiation. Thus their primary use is simply to survey for the presence of radiation and radioactivity.
Ionization Chamber
Ionization chambers are handheld survey instruments used to measure low or high exposure rates ( Fig. 2.2 ). They have an air- or gas-filled chamber but a low efficiency for detection of gamma rays. These instruments have a relatively low applied voltage from anode to cathode; as a result, there is no avalanche effect and no dead time problem. Ionization chambers typically are useful over a wide range of exposure rates from 0.1 mR (2.5 × 10 -8 C/kg)/hr to 100 R (2.5 × 10 -2 C/kg)/hr. A dose calibrator is a special form of an ionization chamber.

Sodium Iodide Well Counter
Well counters are gasless devices common in nuclear medicine laboratories for performing in vitro studies as well as quality control and assurance procedures. Generally, there is a solid cylindrical sodium iodide crystal with a cylindrical well cut into the crystal, into which a radioactive sample is placed, often within a test tube ( Fig. 2.3 ). A photomultiplier tube (PMT) is optically coupled to the crystal base. Radiation from the sample interacts with the crystal emitting low-level light, which is detected and amplified by the PMT feeding into a scalar. The scalar readout directly reflects the amount of radioactivity in the sample and is usually recorded in counts for the period during which the sample is measured.
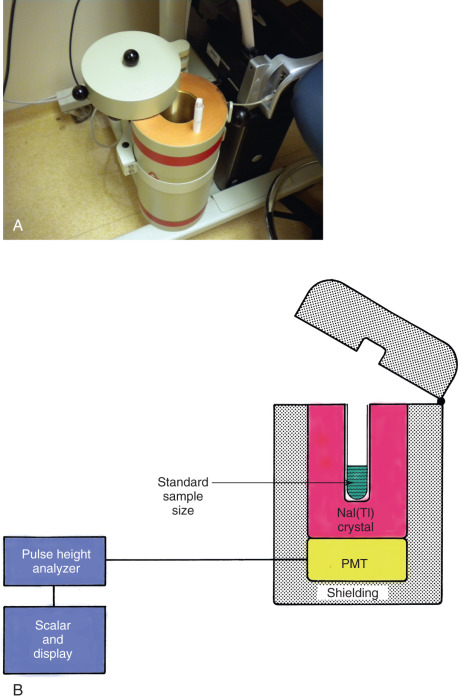
Reflected light and scattering inside the well surface and the thickness of the crystal limit the energy resolution of the standard well counter. Because the sample is essentially surrounded by the crystal, the geometric efficiency for detection of gamma rays is high. Geometric efficiency is defined as the fraction of emitted radioactivity that is incident on the detection portion of the counter, in this case, the crystal. Because the crystal is relatively thick, most low-energy photons undergo interaction, and few pass through undetected. As a result, in the energy ranges below 200 keV, the overall crystal detection efficiency is usually better than 95%.
Because the top of the well in the crystal is open, it is important to keep the sample volume in the test tube small. If varying sample volumes are placed in the well counter, different amounts of radiation escape near the top of the crystal, resulting in unequal geometric efficiencies. Absorption of gamma rays within the wall of the test tube is a factor when lower energy sources, such as iodine-125 ( 125 I), are counted; therefore the sample tubes should also be identical.
Because sodium iodide well counters have such a high detection efficiency, there is a serious problem with electronic dead time. If high levels of activity are used, much of the radiation is not detected. In general, well counters can typically count activity only up to about 1 µCi (37 kBq). Attempts to measure amounts of activity greater than this in a well counter can lead to serious underestimates attributable to dead-time counting errors.
Single Probe Counting Systems
Single probe counting systems using only one crystalline detector are primarily used for measuring thyroid uptake of radioactive iodine. The probe used for thyroid counting is actually similar to the standard well counter in concept ( Fig. 2.4 ), although it does not have the central hole in the sodium iodide crystal. The typical crystal is 5 cm in diameter and 5 cm in thickness, with a cone-shaped (flat field) collimator. As with the well counter, a PMT is situated at the crystal base. When these probes are used, it is important for quantitative consistency to maintain a fixed distance from the face of the crystal to the object being measured and to eliminate all extraneous sources of background radiation.
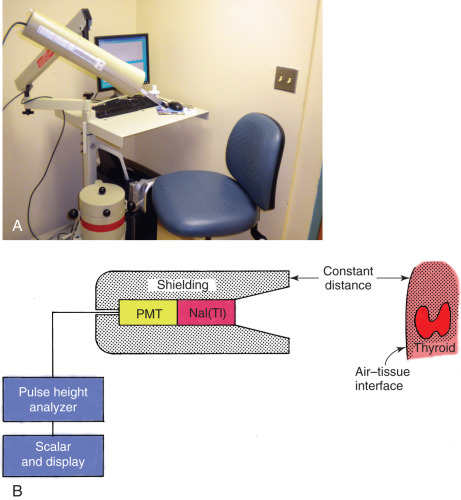
In addition to the larger type probes, there are also handheld probes (and even very tiny gamma cameras) used intraoperatively to identify and localize sentinel lymph nodes and parathyroid adenomas. These need to have excellent spatial resolution and are highly collimated counting devices with solid-state scintillation or semiconductor detectors. Some of the devices have interchangeable probes and can detect gamma, beta, or positron emissions and thus can be used for a variety of radionuclides, including 99m Tc, 111 In, and 18 F.
Dose Calibrator
Because it is extremely important to measure a dose (activity) of a radiopharmaceutical before administration to a patient, the dose calibrator is an essential piece of equipment in any nuclear medicine laboratory. A standard sodium iodide well counter is not useful because the upper limit of sample activity that can be measured accurately is in the microcurie (37 kBq) range. A dose calibrator is essentially a well-type, cylindrical ionization chamber filled with a defined volume of pressurized gas (usually argon) capable of measuring quantities in the millicurie (37 MBq) range. Within the chamber is a collecting electrode ( Fig. 2.5 ). As radiation emanates from the radiopharmaceutical in the syringe, it enters the chamber and interacts with the gas, causing ionization. An electrical differential applied between the chamber and the collecting electrode causes the ions to be captured and measured. This measurement is used to calculate the activity of a dose contained in a syringe or capsule placed in the well. Limits for maximum activity that can be accurately measured by dose calibrators are usually specified for 99m Tc.
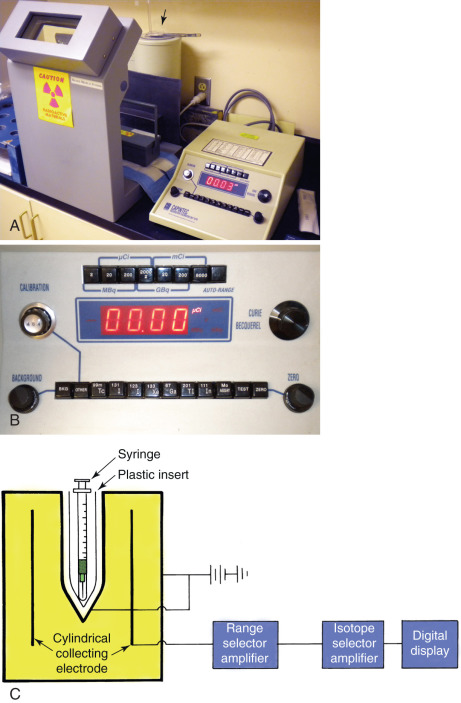
As with other radiopharmaceuticals, the activity of positron emitters may be measured in a typical dose calibrator before administration to the patient. Although a dose calibrator (ionization chamber) cannot determine the energy of emitted photons, the amount of electrical current in the chamber produced by the photons varies directly with photon energy. Because the 511 keV annihilation photons are substantially more energetic than are 99m Tc photons, the current produced is about three times greater. Therefore the maximum activity limit for 18 F is about one-third that specified for 99m Tc. Consequently, a dose calibrator with relatively high specified maximum activity is preferred. In addition, more lead shielding around the dose calibrator is required for measurement of 18 F. It should be at least 5 cm or greater compared with the 4- to 6-mm thickness usually supplied with a standard dose calibrator.
Gamma Scintillation Camera
The most widely used imaging devices in nuclear medicine are the simple gamma scintillation camera and the single-photon emission computed tomography (SPECT) capable gamma camera. A scintillation gamma camera converts photons emitted by the radionuclide in the patient into a light pulse and subsequently into a voltage signal. This signal is used to form an image of the distribution of the radionuclide. The four basic components of a gamma camera system ( Fig. 2.6 ) are the collimator, the scintillation crystal, an array of PMTs, and a computer to convert the output signals into an image display. A free-standing display monitor with an image processing computer (workstation) to further refine, format, and extract quantitative information from the image data, and picture archiving and communication systems are also integral parts of the system. All of the newer cameras incorporate some digital features. Even the most advanced digital scintillation cameras, however, start with the analog signal in the scintillation crystal.
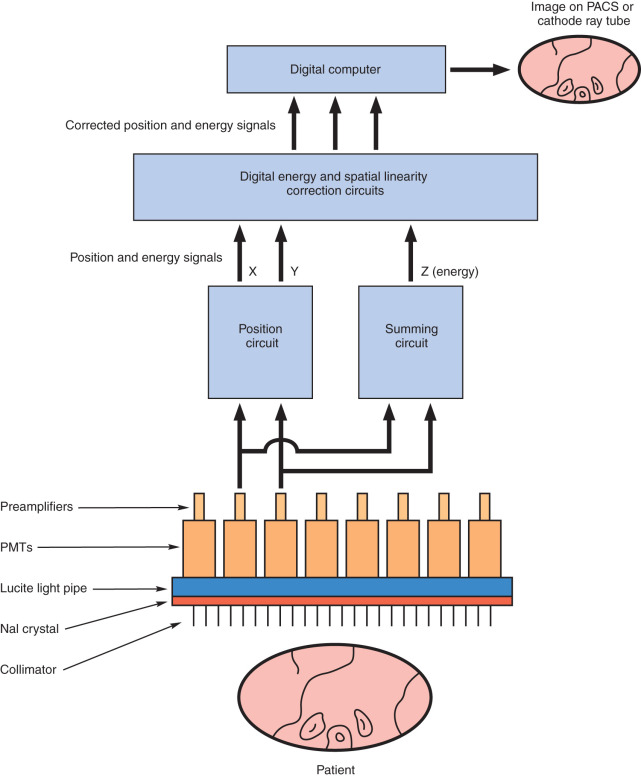
Collimator
The collimator is made of perforated or folded lead approximately 1 to 2 inches (2.5–5 cm) thick and is interposed between the patient and the scintillation crystal. It allows the gamma camera to localize accurately the radionuclide in the patient’s body. Collimators perform this function by absorbing and stopping most radiation except that arriving almost perpendicular to the detector face. Most radiation striking the collimator at oblique angles is not included in the final image. Of all the photons emitted by an administered radiopharmaceutical, more than 99% are “wasted” and not recorded by the gamma camera; less than 1% are used to generate the desired image. Thus the collimator is the “rate limiting” step in the imaging chain of gamma camera technology.
The two basic types of collimators are pinhole and multihole. A pinhole collimator operates in a manner similar to that of a box camera ( Fig. 2.7 ). Radiation must pass through the pinhole aperture to be imaged, and the image is always inverted on the scintillation crystal. Because little radiation coming from the object of interest is allowed to pass through the pinhole over a given period, the pinhole collimator has very poor sensitivity. Collimator sensitivity refers to the percentage of incident photons that pass through the collimator. The poor sensitivity of a pinhole collimator makes placement near the organ of interest critical, and bringing the object of interest close to the pinhole magnifies the image. Because magnification is a function of distance, the image may be distorted if the object of interest is not relatively flat or thin. Pinhole collimators are routinely used for very high resolution images of small organs, such as the thyroid, and for certain skeletal regions, such as hips or wrists, especially in pediatric patients.
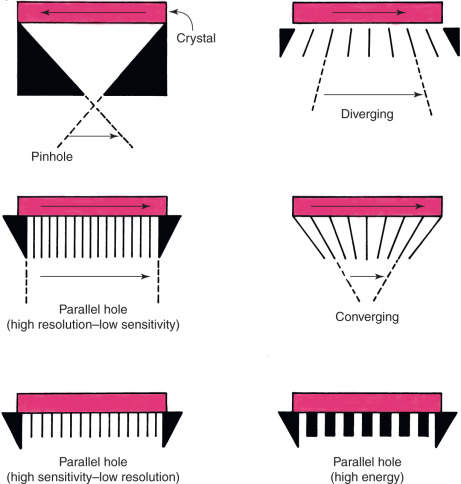
The holes in a multihole collimator may be aligned in a parallel, diverging, or converging manner. The parallel hole collimator is the most widely used multihole collimator in nuclear medicine laboratories. It consists of parallel holes with a long axis perpendicular to the plane of the scintillation crystal. The lead walls between the holes are referred to as septa. The septa absorb most gamma rays that do not emanate from the direction of interest; therefore a collimator for high-energy gamma rays has much thicker septa than does a collimator for low-energy rays. The septa are generally designed so that septal penetration by unwanted gamma rays does not exceed 10% to 25%.
A parallel hole collimator should be chosen to correspond to the energy of the isotope being imaged. Low-energy collimators generally refer to a maximum energy of 150 keV, whereas medium-energy collimators have a maximum suggested energy of about 400 keV. Collimators are available with different lengths and different widths of septa. In general, the longer the septa, the better the resolution but the lower the count rate (sensitivity) for a given amount of radionuclide. The count rate is inversely proportional to the square of the collimator hole length. If the length of the septa is decreased, the detected count rate increases, and resolution decreases ( Fig. 2.8 ).
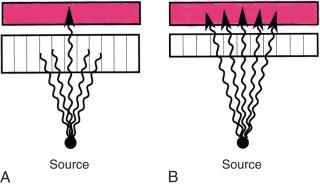
The difference between typical low-energy, general-purpose collimators and low-energy, high-sensitivity collimators is that high-sensitivity collimators may allow about twice as many counts to be imaged, although the spatial resolution is usually degraded by about 50%. A high-resolution, low-energy collimator has about three times the resolving ability of a high-sensitivity, low-energy collimator.
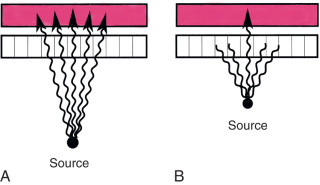
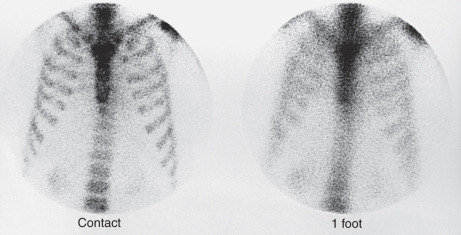
With a parallel-hole collimator, neither the size of the image nor the count rate changes significantly with the distance of the object of interest from the collimator. This is because as the object is moved small distances away from the crystal, the inverse square law reduces the number of counts. However, this is compensated for by the increased viewing area of the collimator. On the other hand, resolution is best when the object of interest is as close to the collimator face as possible ( Figs. 2.9 and 2.10 ), and scans with multihole collimators are usually obtained with the collimator in contact with or as close as possible to the patient. With a parallel-hole collimator, scattered photons emitted from the patient perpendicular to the crystal face may be imaged ( Fig. 2.11 ). These photons and those that penetrate the septa degrade spatial resolution.
Crystal and Other Photon Detector Devices
Radiation emerging from the patient and passing through the collimator typically interacts with a thallium-activated sodium iodide crystal. Crystals also can be made with thallium or sodium-activated cesium iodide or even lanthanum bromide, but these are uncommon. Interaction of the gamma ray with the crystal may result in ejection of an orbital electron (photoelectric absorption), producing a pulse of fluorescent light (scintillation event) proportional in intensity to the energy of the gamma ray. PMTs situated along the posterior crystal face detect this light and amplify it. About 30% of the light from each event reaches the PMTs. Because sodium iodide crystals are fragile and hygroscopic, they must be encased in a sealed aluminum housing to protect them from moisture, extraneous light, and minor physical damage.
The crystals are typically 10 to 25 inches in diameter and may be circular, square, or rectangular. For most cameras, a 6- to 12-mm thick crystal is used. A larger-diameter crystal has a larger field of view (FOV) and is more expensive, but has the same inherent resolution as does a smaller-diameter crystal. The thicker the crystal becomes, the worse the spatial resolution but the more efficient the detection of gamma rays. In general, with a 12-mm thick crystal, the efficiency for detection of gamma rays from xenon-133 ( 133 Xe) (81 keV) and technetium-99m ( 99m Tc) (140 keV) is almost 100%; that is, few of the photons pass through the crystal without causing a light pulse. As the gamma energy of the isotope is increased, the efficiency of the crystal is markedly reduced. For example, with iodine-131 ( 131 I) (364 keV), efficiency is reduced to about 20% to 30%. With a thinner crystal, the overall sensitivity (count rate) decreases by about 10% because more photons pass through, but there is about a 30% increase in spatial resolution because the PMTs are closer to the event and thus can localize it more accurately, and because there is an increase in light collection. Some newer cameras have pixilated detectors in which the FOV is covered by an array of detectors with a face size of 2 to 3 mm instead of a single large crystal.
Photon Transducers
A PMT converts a light pulse into an electrical signal of measurable magnitude. An array of these tubes is situated behind the sodium iodide crystal and may be placed directly on the crystal, connected to the crystal by light pipes, or optically coupled to the crystal with a silicone-like material. A scintillation event occurring in the crystal is recorded by one or more PMTs. Localization of the event in the final image depends on the amount of light sensed by each PMT and thus on the pattern of PMT voltage output. The summation signal for each scintillation event is then formed by weighing the output of each tube. This signal has three components: spatial coordinates on x- and y-axes as well as a signal ( z ) related to intensity (energy). The x- and y-coordinates may go directly for real-time display on a cathode ray tube (CRT) or may be recorded in the computer. The signal intensity is processed by the pulse height analyzer (PHA).
The light interaction caused by a gamma ray generally occurs near the collimator face of the crystal. Thus, although a thicker crystal is theoretically more efficient, the PMT is farther away from the scintillation point with a thick crystal and is unable to determine the coordinates as accurately. Therefore spatial resolution is degraded. The number of PMTs is also important for the accurate localization of scintillation events; thus for spatial resolution, the greater the number of PMTs, the greater the resolution. Most gamma cameras use about 40 to 100 hexagonal, square, or round PMTs.
Some newer scintillation imaging systems have used position-sensitive PMTs (PS-PMTs) and avalanche photodiodes (APD). PS-PMTs are usually used with small FOV devices that have pixilated detectors, rather than a large single crystal. APDs are solid-state photon converters that can be thought of as a light-sensitive diode and are being used in positron emission tomography/magnetic resonance imaging (PET/MRI) applications because they are less sensitive to magnetic fields.
Solid-State Photon Detectors
The majority of gamma cameras currently in use are based on crystal scintillation light detection systems invented more than 50 years ago. Over the last several decades there has been progress in development of solid-state radiation detection and imaging devices that can operate at room temperatures, do not have vacuum tubes nor hygroscopic crystals, and are much less bulky.
The sodium iodide crystal gamma camera can be thought of as an indirect detection system because the photons must be absorbed in the scintillator, converted to light, which is subsequently converted to an electrical signal, and amplified by a bulky PMT before energy and position evaluation occurs. There are also unavoidable light signal losses in the scintillator.
Solid-state imaging relative to gamma cameras means the absence of both vacuum tubes and PMTs; they instead use segmented crystals and semiconductors. Solid-state gamma cameras use a cesium iodide (CsI) scintillator or a semiconductor cadmium zinc telluride (CZT) matrix detector. CsI(Tl) crystalline detectors can be made of many tiny columnar crystals and have “indirect” conversion of light to electricity with a photodiode attached to each tiny individual pixel crystal.
In semiconductor direct detection systems there is no need to go through the optical phase. A semiconductor is made of a material that normally does not conduct electricity, but when various impurities are included, there is either an excess of electrons (N material [think of negative]) or a deficit of electrons (P material [think of positive]), which allows a current to flow through the material ( Fig. 2.12 ). When an external current is applied to a block of joined N and P semiconductor material, the electrons and electron “holes” are pulled toward the anode and cathode, respectively, leaving a depletion zone in the middle. When photons interact in this zone, by Compton or photoelectric interaction, they convert the photon energy into high-kinetic-energy electrons and holes (e-h pairs) which in turn create secondary e-h pairs until the energy is so low that the e-h pairs recombine. The detectors collect the electrons and holes to produce a current detected in the readout circuit ( Fig. 2.13 ) Semiconductor CZT has higher absorption efficiency than scintillation detectors of the same thickness. Semiconductor detector systems typically have two times better energy resolution, more than two times better spatial resolution (2.5 mm versus 5–6 mm), and up to ten times more count sensitivity than scintillator sodium iodine crystal systems.

The CZT system uses a collimator and an array of crystal units (about 4 cm each) that are pixelated (2.5 mm) and formed as tiles, which are placed together to form the detector. Usually the irradiated side of the detectors is the cathode side and the detector array with pixels is on the anode or readout side. The pixel readout is used to obtain spatial information. For each pixel to be read out individually requires thousands of electronic channels, making discrete electronics impractical. The detector is coupled electrically by bump bonds to a microelectronic circuit connected pixel by pixel to an application specific integrated circuit (ASIC) for signal readout ( Fig. 2.14 ), position, counting, and energy analysis.
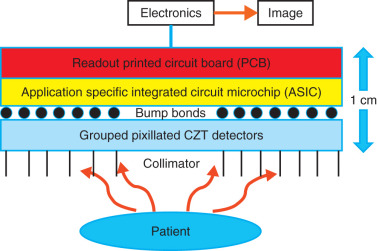
The usable energy range is 40 to 250 keV and the FOV can be as large as 50 × 40 cm. A number of these systems are currently available combined with CT, and research is underway to develop hybrid semiconductor gamma camera MRI units (because PMTs cannot function in a high magnetic field).
Pulse Height Analyzer
The basic principle of the PHA in a sodium iodide scintillator system is to discard signals from background and scattered radiation and/or radiation from interfering isotopes, so that only primary photons known to come from the photopeak of the isotope being imaged are recorded. The PHA discriminates between events occurring in the crystal that will be displayed or stored in the computer and events that will be rejected. The PHA can make this discrimination because the energy deposited by a photon bears a linear relation to the voltage signal emerging from the PMTs.
A typical energy spectrum from a PHA is shown in Fig. 2.15 . The photopeak is the result of total absorption of the major gamma ray from the radionuclide. If the characteristic K-shell x-ray of iodine (28 keV) escapes from the crystal after the gamma ray has undergone photoelectric absorption, the measured gamma-ray energy for 99m Tc would be only 112 keV (140 minus 28 keV). This will cause an iodine escape peak.

A backscatter peak may result when primary gamma rays undergo 180-degree scatter and then enter the detector and are totally absorbed. This can occur when gamma rays strike material behind the source and scatter back into the detector. It may also occur when gamma rays pass through the crystal without interaction and Compton scatter from the shield or PMTs back into the crystal.
The lead x-ray peak is caused by primary gamma rays undergoing photoelectric absorption in the lead of shielding or the collimator; as a result, characteristic x-rays (75 to 90 keV) are detected. The effect of Compton scattering in the detector gives a peak from 0 to 50 keV. The sharp edge at 50 keV is called the Compton edge. If the source of radiation is within a patient, Compton scattering occurs within the patient’s tissue, and some of these scattered gamma rays travel toward the detector with an energy from 90 to 140 keV. These scattered photons from within the patient cause imaging difficulties, because the Compton scatter overlaps with the photopeak distribution.
Signal intensity information is matched in the PHA against an appropriate window, which is really a voltage discriminator. To allow energy related to the desired isotope photopeak to be recorded, the window has upper and lower voltage limits that define the window width. Thus a 20% symmetric window for 140 keV photopeak means that the electronics will accept 140 ± 14 keV (i.e., 140 keV ± 10%) gamma rays. Any signals higher or lower than this, particularly those from scattered radiation, are rejected. Most cameras have multiple PHAs, which allow several photopeaks to be used at once. This is particularly useful for radionuclides with multiple gamma emissions of different energies, such as indium-111 ( 111 In) and gallium-67 ( 67 Ga). On newer cameras, the signal processing circuitry, such as preamplifiers and PHAs, is located on the base of each PMT so that there is little signal distortion between the camera head and the console. In solid-state semiconductor systems the readout ASIC has photon energy discrimination and there is no need for a separate PHA.
Console Controls
Most gamma cameras allow for a fine adjustment known as automatic peaking of the isotope. This essentially divides the photopeak window in half and calculates the number of counts in each half. If the machine is correctly peaked, each half of the window has the same number of counts from the upper and lower portions of the photopeak. Occasionally, an asymmetric window is used to improve resolution by eliminating some of the Compton scatter ( Fig. 2.16 )
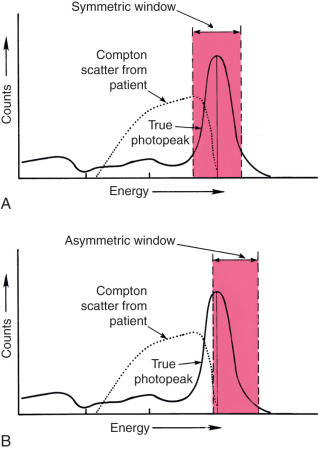
Image exposure time is selected by console control and is usually a preset count, a preset time, or preset information density for the image accumulation. Information density refers to the number of counts per square centimeter of the gamma camera crystal face. Other console controls are present for orientation and allow the image to be reversed on the x- and y-axes.
In addition, the CRT image may be manipulated by an intensity control, which simply affects the brightness of the image, or by a persistence control, which regulates the length of time the light dots composing the image remain on the screen. Hard-copy images on film may be obtained directly from the computer, although most institutions now display digital images on monitors and store the images in a picture archiving system.
Resolution
Resolution is one of the common performance parameters for gamma cameras. Resolution usually refers to either spatial or energy resolution. Energy resolution is the ability to discriminate between light pulses caused by gamma rays of differing energies. Spatial resolution refers to the ability to display discrete but contiguous sources of radioactivity. The spatial resolution of various gamma camera systems is usually given in terms of either inherent or overall resolution. Inherent spatial resolution is the ability of the crystal PMT detector and accompanying electronics to record the exact location of the light pulse on the sodium iodide crystal. Gamma cameras have an inherent resolution of about 3 mm.
Statistical variability is particularly important in resolution. An event occurring exactly between two PMTs does not always give the same number of photons to each tube; thus for any single event, the distribution of photons is statistically variable. Statistical variation is relatively greater when fewer light photons are available. In other words, the inherent resolution of a system or its ability to localize an event is directly related to the energy of the isotope being imaged. When radioisotopes with low-energy gamma rays or characteristic x-rays are used, the camera has less inherent spatial resolution.
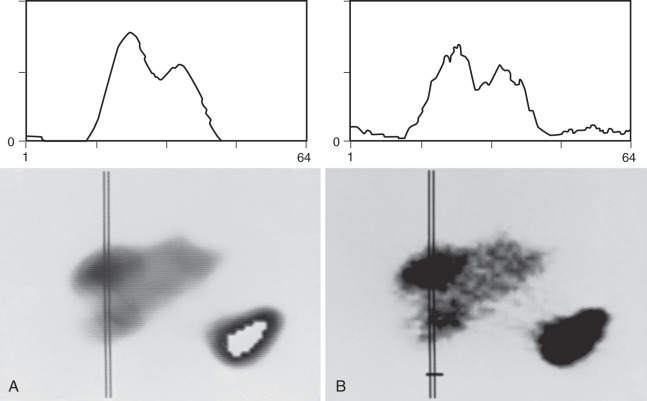
Overall spatial resolution is the resolution capacity of the entire camera system, including such factors as the collimator resolution, septal penetration, and scattered radiation. The simplest method of examining overall spatial resolution is to determine the full width at half maximum (FWHM) of the line spread function. This refers to the profile response of the gamma camera to a single point source of radioactivity, and reflects the number of counts seen by the crystal at different lateral distances from the source ( Fig. 2.17A ). The source is often placed 10 cm from the crystal for these measurements. The FWHM is expressed as the width in centimeters at 50% of the height of the line spread peak. The narrower the peak, the better the resolution. When state-of-the-art cameras and 99m Tc are used, the position of scintillation events can be determined to within 3 to 5 mm. A typical high-resolution collimator has three times better resolution than does a representative high-sensitivity collimator but allows only one-tenth as many counts per minute for a given activity.
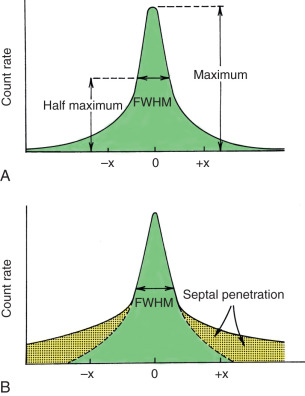
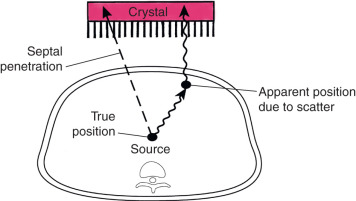
Although spatial FWHM is useful for comparing collimators, it often does not give other desirable information and does not necessarily relate to the overall clinical performance of the collimator. More difficult, but perhaps more encompassing, measurements of collimator performance are modulation transfer functions, which take other factors for optimizing collimator design, such as the presence of scattering material and septal penetration, into account. The value of this can be seen in Fig. 2.17B , which illustrates that the septal penetration occurring in the collimator may be completely undetected by the measurement of FWHM alone.
When the overall spatial resolution of the system with high-energy isotopes is considered, the limiting resolution is that of the collimator. When low-energy isotopes are imaged, the inherent resolution becomes more important than the collimator resolution. As the energy of the incident gamma ray decreases, the inherent resolution of the crystal decreases markedly because the lower-energy gamma rays provide less light for the PMTs to record; thus there is more statistical uncertainty regarding the origin of the gamma ray. Although the inherent resolution of cameras is often championed by salespeople, the overall resolution determines the quality of the image because it is a combination of the resolutions of each of the components in the imaging chain, including the collimator, the inherent resolution, septal penetration, and scatter. The overall system resolution ( R s ) is
R s = square root of the sum of R 2 i + R 2 c
Another category of resolution is energy resolution, or the ability of the imaging system to separate and distinguish between the photopeaks of different radionuclides. If the energy resolution is good, the photopeaks are tall and narrow; if energy resolution is poor, the photopeaks appear as broad bumps in the energy spectrum. The FWHM concept is also used to examine energy resolution and is usually quoted for the relatively high-energy (662 keV) photon of cesium-137 ( 137 Cs). With lower-energy photons, the energy resolution is worse. Most gamma cameras have an energy resolution of 10% to 15%, allowing use of 15% to 20% energy windows to encompass all the photons of interest.
Count Rate and Dead Time
As with any detection system, it is important that scintillation events do not occur so fast that the electronic system is unable to count each as a separate event. If two equal light pulses occur too close together in time, the system may perceive this as one event with twice the energy actually present. Such an occurrence of primary photons would be eliminated by the energy window of the PHA, and none of the information from the two events would be imaged; thus the sensitivity of the system would be diminished. A more significant problem is loss of spatial resolution when several scattered (low-energy) photons strike the crystal at the same time, so that their light production is summed and mimics a primary photon of interest. The time after an event during which the system is unable to respond to another event is referred to as dead time . Dead time can be important in high count rate dynamic studies (in the range of 50,000 counts/sec), particularly with single crystal cameras. An example is a first-pass cardiac study.
Field Uniformity
Despite the efforts of manufacturers to produce high-quality collimators, crystals, PMTs, and electronics, nonuniformity inevitably occurs. Acceptable field nonuniformity is on the order of 2% to 5%. Much of this can be corrected by the computer system. Analysis of field uniformity is discussed later in the chapter.
Image Acquisition: Memory and Matrix Size
Data may be acquired either by frame mode or by list mode. In the frame mode, incoming data are placed in a spatial matrix in the memory that is used to generate an image. In the list mode, all data are put in the memory as a time sequence list of events. At regular intervals, a special code word is inserted into the list. This list is flexible and can be sorted or divided into images at a later time. The list mode has the disadvantage of a low acquisition rate and a large memory requirement. Frame mode uses much less memory than does the list mode and is more commonly used, except for gated cardiac studies. All data for images that are collected in the frame mode are acquired in a matrix. The usual image matrix sizes are 64 × 64 and 128 × 128, although 32 × 32 and 256 × 256 matrix sizes are occasionally used. The main disadvantage of frame mode is that the identity of individual events within a time frame is lost.
Matrix size refers to the number of picture elements along each side of the matrix. These elements may be either bytes or words. In an 8-bit computer, both a byte and a word are composed of 8 bits. In a 16-bit computer, a byte is 8 bits and a word is 16 bits. The maximum number of counts that can be represented by an 8-bit picture element (pixel) is 2 8 , or 0 through 255 (256 different values). Ordinarily, 16-bit collections are used; the maximum size is 2 16 , or 0 through 65,535 (65,536 different values) per pixel.
The matrix size determines the image resolution. Although the matrix size and the number of counts desired have a significant impact on memory required, the ultimate memory requirements depend on what the computer system is being used for and how many cameras it is interfaced with simultaneously. The matrix size has nothing to do with the final size of the displayed image. A 32 × 32 matrix has relatively few pixels; therefore the final image is coarse. An image obtained in a 256 × 256 acquisition matrix is much more detailed. Remember that an image resolution of 256 × 256 may refer to either the memory acquisition matrix or the CRT display matrix. Some manufacturers take a 64 × 64 matrix image from the memory and display it on the CRT in a 256 × 256 or 1024 × 1024 matrix, using interpolation methods.
The 32 × 32 matrix occupies less memory and therefore less disk space. In addition, it can be acquired faster than can a finer matrix. Thus there is a trade-off between spatial and temporal resolution. In a 32 × 32 matrix, the spatial resolution is poor but, because it can be acquired rapidly, the temporal resolution is excellent. For a given computer system, the matrix size desired for acquisition and the read-write speed of the hard disk dictate the maximum framing rate that is possible.
The amount of memory determines the number of frames that can be collected in the electrocardiographic R-R interval on electrocardiogram gated cardiac studies. For optimum measurement of ejection fraction, at least 25 frames/sec are needed. If peak ejection or peak filling rate is to be measured, 50 frames/sec are needed.
Image Display and Processing
Image display and processing is necessary in all nuclear medicine computer systems. The computer plays an extremely important role in lesion detectability, and can perform this function in a number of ways, including reduction of noise, background subtraction, construction of cine loops, and production of tomographic images. Data are normally collected in 64 × 64 byte images. Although a 32 × 32 byte mode can be used, the decrease in spatial resolution is usually intolerable. Even in 64 × 64 pixel images, there is a noticeable saw-toothed appearance to the image edges. Because the pixel matrix achieved on a display video is 1024 × 1024 with 256 levels of gray, the data are usually processed to use all the pixels. The simplest method to fill in the extra pixels is linear interpolation.
To reduce the effects of statistical variation, particularly in low-count images, the image can be smoothed. Smoothing is accomplished through the use of filters, which may be either spatial or temporal. Temporal filters are used for dynamic acquisition, and spatial filters are used on static images. Spatial filters attempt to remove statistical fluctuations of the image by modifying values of data points within various pixels.
Spatial Filters
The processing performed by spatial filters is done according to the spatial frequencies of the information. By attenuating or augmenting parts of the spatial frequency spectrum, an image should be obtained that is easier to interpret or that has more diagnostic value. The simplest smoothing method is nine-point smoothing. This takes 9 pixels of information and, by taking weighted averages of the 8 pixels on the edge of a central pixel, changes the value of that central pixel.
Other kinds of filters that are commonly used are low-pass, high-pass, and band-pass filters. A low-pass filter selectively attenuates high frequencies and smoothes the image by removing high-frequency noise. This filtering improves the statistical quality of an image but degrades the sharpness and spatial resolution. Fig. 2.18A shows an example of low-pass filtering applied to data from a SPECT liver scan. High-pass filtering enhances edges to some extent but also augments the noise ( Fig. 2.18B ). This type of filtering is important in cardiac nuclear medicine for locating the edges of the myocardium. A band-pass filter is a combination of low-pass and high-pass filters that effectively suppresses high-frequency and low-frequency signals and transmits only signals that are in a given spatial frequency window.