Abstract
This chapter contains the fundamentals of nuclear physics related to radionuclides, methods of production, and discussion of the types of radiopharmaceuticals, as well as mechanisms of localization. Also included are issues of quality control and unsealed radionuclides used for radiation therapy.
Keywords
radiopharmaceuticals, radionuclides, radioisotope production, radiopharmacy, radioactive Decay, nuclear medicine quality control, radiopharmaceutical adverse reactions
Basic Isotope Notation
The atom may be thought of as a collection of protons, neutrons, and electrons. The protons and neutrons are found in the nucleus, and shells of electrons orbit the nucleus with discrete energy levels. The number of neutrons is usually designated by N. The number of protons is represented by Z (also called the atomic number ). The atomic mass number, or the total number of nuclear particles, is represented by A and is simply the sum of N and Z. The symbolism used to designate atoms of a certain element having the chemical symbol X is given by 
. For example, the notation 
refers to a certain isotope of iodine. In this instance, 131 refers to the total number of protons and neutrons in the nucleus. By definition, all isotopes of a given element have the same number of protons and differ only in the number of neutrons. For example, all isotopes of iodine have 53 protons.
Nuclear Stability and Decay
A given element may have many isotopes, and some of these isotopes have unstable nuclear configurations of protons and neutrons. These isotopes often seek greater stability by decay or disintegration of the nucleus to a more stable form. Of the known stable nuclides, most have even numbers of neutrons and protons. Nuclides with odd numbers of neutrons and protons are usually unstable. Nuclear instability may result from either neutron or proton excess. Nuclear decay may involve a simple release of energy from the nucleus or may actually cause a change in the number of protons or neutrons within the nucleus. When decay involves a change in the number of protons, there is a change of element. This is termed a transmutation. Isotopes attempting to reach stability by emitting radiation are radionuclides.
Several mechanisms of decay achieve stability. One of these is alpha-particle emission. In this case, an alpha (α) particle, consisting of two protons and two neutrons, is released from the nucleus, with a resulting decrease in the atomic mass number ( A ) by four and reduction of both Z and N by two. The mass of the released alpha particles is so great that they travel only a few centimeters in air and are unable to penetrate even thin paper. These properties cause alpha-particle emitters to be essentially useless for imaging purposes.
Beta-particle emission is another process for achieving stability and is found primarily in nuclides with a neutron excess. In this case, a beta (β−) particle (electron) is emitted from the nucleus accompanied by an antineutrino; as a result, one of the neutrons may be thought of as being transformed into a proton, which remains in the nucleus. Thus, beta-particle emission decreases the number of neutrons ( N ) by one and increases the number of protons ( Z ) by one, so that A remains unchanged ( Fig. 1.1 ). When Z is increased, the arrow in the decay scheme shown in Fig. 1.1 points toward the right, and the downward direction indicates a more stable state. The energy spectrum of beta-particle emission ranges from a certain maximum down to zero; the mean energy of the spectrum is about one-third of the maximum. A 2-MeV beta particle has a range of about 1 cm in soft tissue and is therefore not useful for imaging purposes.
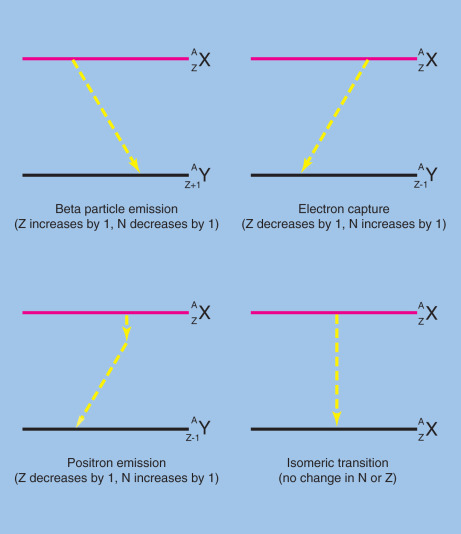
Electron capture occurs in a neutron-deficient nuclide when one of the inner orbital electrons is captured by a proton in the nucleus, forming a neutron and a neutrino. This can occur when not enough energy is available for positron emission, and electron capture is therefore an alternative to positron decay. Because a nuclear proton is essentially changed to a neutron, N increases by one, and Z decreases by one; therefore, A remains unchanged (see Fig. 1.1 ). Electron capture may be accompanied by gamma emission and is always accompanied by characteristic radiation, either of which may be used in imaging.
If, in any of these attempts at stabilization, the nucleus still has excess energy, it may be emitted as nonparticulate radiation, with Z and N remaining the same. Any process in which energy is given off as gamma rays and in which the numbers of protons and neutrons are not changed is called isomeric transition (see Fig. 1.1 ). An alternative to isomeric transition is internal conversion. In internal conversion, the excess energy of the nucleus is transmitted to one of the orbital electrons; this electron may be ejected from the atom, which is followed by characteristic radiation when the electron is replaced. This process usually competes with gamma-ray emission and can occur only if the amount of energy given to the orbital electron exceeds the binding energy of that electron in its orbit.
The ratio of internal conversion electrons to gamma-ray emissions for a particular radioisotope is designated by the symbol α. (This should not be confused with the symbol for an alpha particle.) For an isotope such as technetium-99m ( 99m Tc), α is low, indicating that most emissions occur as gamma rays with little internal conversion. A low conversion ratio is preferable for in vivo usage because it implies a greater number of gamma emissions for imaging and a reduced number of conversion electrons, which are absorbed by the body and thus add to the patient’s radiation dose.
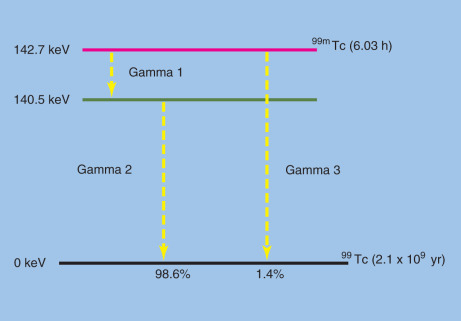
In many instances, a gamma-ray photon is emitted almost instantaneously after particulate decay. If there is a measurable delay in the emission of the gamma-ray photon and the resulting decay process is an isomeric transition, this intermediate excited state of the isotope is referred to as metastable. The most well-known metastable isotope is 99m Tc (the m refers to metastable). This isotope decays by isomeric transition to a more stable state, as indicated in Fig. 1.2 . In the decay scheme, the arrows point straight down, showing that there is no change in Z. Also, 99m Tc may decay by one of several routes of gamma-ray emission.
In cases in which there are too many protons in the nucleus (a neutron-deficient nuclide), decay may proceed in such a manner that a proton may be thought of as being converted into a neutron. This results in positron (β + ) emission , which is always accompanied by a neutrino. This obviously increases N by one and decreases Z by one, again leaving A unchanged (see Fig. 1.1 ). The downward arrow in the decay scheme again indicates a more stable state, and its leftward direction indicates that Z is decreased. Positron emission cannot occur unless at least 1.02 MeV of energy is available to the nucleus.
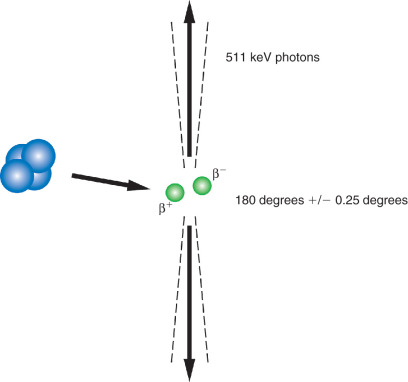
When a positron is emitted, it travels for a short distance from its site of origin, gradually losing energy to the tissue through which it moves. When most of its kinetic energy has been lost, the positron reacts with a resident electron in an annihilation reaction. This reaction generates two 511-keV gamma photons, which are emitted in opposite directions at about (but not exactly) 180 degrees from each other ( Fig. 1.3 ).
Radionuclide Production
Most radioactive material that does not occur naturally can be produced by particulate bombardment or nuclear fission. Both methods alter the neutron-to-proton ratio in the nucleus to produce an unstable isotope. Bombardment essentially consists of the irradiation of the nuclei of selected target elements with neutrons in a nuclear reactor or with charged particles (alpha particles, protons, or deuterons) from a cyclotron. Bombardment reactions may be summarized by equations in which the target element and bombarding particle are listed on the left side of the equation and the product and any accompanying particulate or gamma emissions are indicated on the right. For example,
Z A X + n ( neutron ) → Z A + 1 X + γ or more specifically
42 98 Mo + n ( neutron ) → 42 99 Mo + γ
These equations may be further abbreviated using parenthetical notation. The molybdenum reaction presented previously is thus represented as 98 Mo ( n, γ) 99 Mo. The target and product are noted on the outside of the parentheses, which contain the bombarding particle on the left and any subsequent emissions on the right.
Once bombardment is completed, the daughter isotope must be physically separated from any remaining and unchanged target nuclei, as well as from any target contaminants. Thus, it is obvious that the completeness of this final separation process and the initial elemental purity of the target are vital factors in obtaining a product of high specific activity. Because cyclotron isotope production almost always involves a transmutation (change of Z ) from one element to another, this process aids greatly in the separation of the radionuclides to obtain carrier-free isotopes (i.e., isotopes that have none of the stable element accompanying them). Radionuclides made by neutron bombardment, which does not result in a change of elemental species (e.g., 98 Mo [ n, γ] 99 Mo), are not carrier free because the chemical properties of the products are identical, and thus radionuclides are not as easily separated.
Fission isotopes are simply the daughter products of nuclear fission of uranium-235 ( 235 U) or plutonium-239 ( 239 Pu) in a reactor and represent a multitude of radioactive materials, with atomic numbers in the range of roughly half that of 235 U. These include iodine-131 ( 131 I), xenon-133 ( 133 Xe), strontium-90 ( 90 Sr), molybdenum-99 ( 99 Mo), and cesium-137 ( 137 Cs), among others. Because many of these isotopes are present together in the fission products, the desired isotope must be carefully isolated to exclude as many contaminants as possible. Although this is sometimes difficult, many carrier-free isotopes are produced in this manner.
Neutron bombardment and nuclear fission almost always produce isotopes with neutron excess, which decay by beta emission. Some isotopes, such as 99 Mo, may be produced by either method. Cyclotron-produced isotopes are usually neutron deficient and decay by electron capture or positron emission. Some common examples of cyclotron-produced isotopes include iodine-123 ( 123 I), fluorine-18 ( 18 F), gallium-67 ( 67 Ga), indium-111 ( 111 In), and thallium-201 ( 201 Tl). In general, cyclotron-generated radionuclides are more expensive than are those produced by neutron bombardment or fission.
Positron-emitting radionuclides are most commonly produced in cyclotrons by charged-particle bombardment of a stable element with protons, deuterons, or helium nuclei. The produced radionuclides have an excess of protons and decay by the emission of positrons.
Radioactive Decay
The amount of radioactivity present (the number of disintegrations per second) is referred to as activity. In the past, the unit of radioactivity has been the curie (Ci), which is 3.7 × 10 10 disintegrations per second. Because the curie is an inconvenient unit, it has been largely replaced by an international unit called a becquerel (Bq), which is 1 disintegration per second. Conversion tables are found in Appendixes B.1 and B.2 . Specific activity refers to the activity per unit mass of material (mCi/g or Bq/g). For a carrier-free isotope, the longer the half-life of the isotope, the lower is its specific activity.
Radionuclides decay in an exponential fashion, and the term half-life is often used casually to characterize decay. Half-life usually refers to the physical half-life, which is the amount of time necessary for a radionuclide to be reduced to half of its existing activity. The physical half-life ( T p ) is equal to 0.693/λ, where λ is the decay constant. Thus, λ and the physical half-life have characteristic values for each radioactive nuclide. Decay tables for various radionuclides are presented in Appendix C .
A formula that the nuclear medicine physician should be familiar with is the following:
A = A 0 e − 0.693 / T p ( t )
This formula can be used to find the activity ( A ) of a particular radioisotope present at a given time ( t ) and having started with activity ( A 0 ) at time 0. For instance, if you had 5 mCi (185 MBq) of 99m Tc at 9:00 a.m. today, how much would remain at 9:00 a.m. tomorrow? In this case, T p of 99m Tc is 6 hours, t is 24 hours, and e is a mathematical constant. Thus,
A = A 0 e − 0.693 T p ( t )
A = A 0 e − 0.693 6 hours ( 24 hours )
Related posts:
Stay updated, free articles. Join our Telegram channel
Full access? Get Clinical Tree
