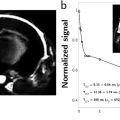
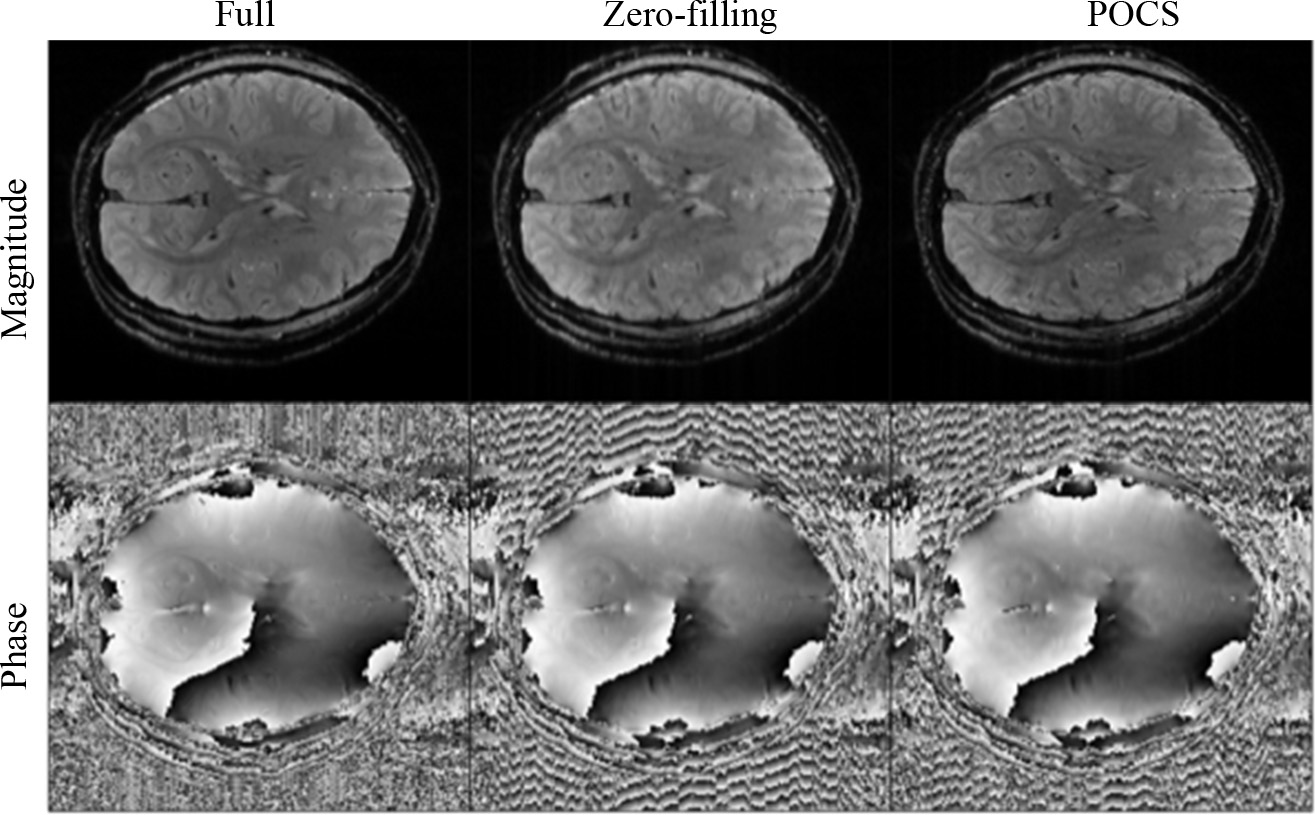
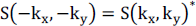Nadège CORBIN, Sylvain MIRAUX, Valéry OZENNE, Émeline RIBOT and Aurélien TROTIER CRMSB, CNRS, Université de Bordeaux, France MRI has become an indispensable clinical technology for patient diagnosis or therapy monitoring. It is also a powerful tool for studying and performing longitudinal follow-up of healthy and pathological models in small animals. Given the large increase in the usage of this technology, the need for faster image acquisition has emerged. Other constraints, including physiological limitations and patient movement during the scan, as well as the need for dynamic image acquisitions and multi-contrast protocols, have spurred the development of acceleration methods. The numerous advances in the domain of acquisition sequences and image reconstruction have thus a goal of providing images with excellent quality while maintaining or reducing the acquisition time. In this chapter, we will consider the notion of fast imaging by describing the most commonly used sequences and acceleration techniques. Finally, the last section will be dedicated to applications requiring rapid MRI acquisitions. Fast imaging is associated with the notion of temporal resolution. In MRI, this concept concerns two aspects. The most intuitive one is the time required to acquire a full image. To visualize a dynamic phenomenon, such as a contrast agent entering inside a tissue, the image acquisition time has to be much shorter than the duration of the observed phenomenon. As an example, in functional brain imaging, current techniques acquire about 30 slices of the brain in less than 1 second (s). This temporal resolution permits, for example, following the activation states of cerebral structures during the execution of a task. Advances in MRI have even provided this technology with “real-time” capabilities for some applications. However, the real-time notion is not only related to the acquisition time, but also to the time necessary for reconstructing and displaying an image. Nowadays, it is possible to perform surgical interventions with real-time MRI guidance (see Chapter 11). Another way of considering the temporal resolution is related to the periodicity of an observed phenomenon and to the ways of decomposing such a behavior. For instance, let us take the example of cardiac imaging. Under normal physiological conditions, heart movement is regular and periodic. In humans, heartbeats occur approximately every second. Using MRI, it is possible to decompose this cardiac rhythm into several phases by acquiring images with a very high temporal resolution (of the order of 5 ms in mice). For this kind of acquisition, the sequence repetition time is going to define the temporal resolution. However, to acquire these images (i.e. to obtain full k-spaces), the acquisition will be repeated over several cardiac periods and will thus last several minutes. If MR image acquisitions have to be performed rapidly, the first step consists of exploiting the available time during an MR sequence in the most efficient way possible. Basic MRI sequences include many dead times useful for magnetization recovery that can be leveraged to “gain some time”. Hence, in a 2D gradient-echo MRI sequence with T1 weighting (TE/TR = 5/400 ms), the dead time can be used to: Multi-echo sequences were already introduced in the early days of MRI with the idea of gathering several k-space lines during the sequence dead times. The two main types of such sequences are rapid imaging with refocused echoes (RARE) (Hennig et al. 1986) and echo-planar imaging (EPI) (Ordidge et al. 1988). Figure 3.1. (a) Chronogram of a RARE sequence with the first three echoes of the echo train. (b) Chronogram of an EPI sequence with the first six echoes of the echo train A RARE sequence uses an RF excitation pulse followed by a train of refocusing pulses to produce multiple spin echoes. Each echo is spatially encoded such that several k-space lines can be sampled for each excitation pulse. As a result, acquisition times can be greatly reduced compared to a standard spin echo sequence. RARE sequences are among the most used MRI sequences, especially for acquiring T2-weighted scans. They are extremely versatile: their chronograms can be easily modified to acquire images in two or three dimensions, they can be adapted to non-Cartesian methods (PROPELLER, BLADE) and several modules (fat suppression, FLAIR) can be added. Moreover, image reconstruction is relatively simple as it requires very few corrections compared to EPI sequences. The principle of an EPI sequence is comparable to that of a RARE sequence. However, the different echoes are generated from a gradient echo and not a spin echo. EPI sequences are therefore faster than RARE sequences. The former are usually used for functional brain imaging, for quickly encoding the signal after a diffusion module, or for first-pass imaging of a contrast agent during perfusion MRI. EPI sequences are T2*-weighted. Echo train length (ETL) is an important parameter in RARE (and EPI) sequences. It defines the reduction of the acquisition time compared to a spin echo sequence. The longer the echo train is, the more the acquisition time is reduced. The maximum ETL is constrained by two factors: tissue relaxation time T2 (i.e. the time during which the transverse magnetization decreases) and the elapsed time between the centers of two consecutive spin echoes. The latter is also called inter-echo time. In practice, for a T2-weighted RARE sequence with TE = 80 ms, ETL is 32 with an inter-echo time of 5 ms. Image contrast can be modified depending on the way in which the RARE sequence encodes the k-space. In simple terms, if the first echo of the echo train is used to encode the central line of the k-space, then the sequence’s TEeffective (time between the RF pulse and the echo of the k-space center) is short and the image will have a T1 weighting. On the other hand, if the last echo is used to encode the center of the k-space, TEeffective is long and the sequence becomes T2-weighted. The most classical trajectory for sampling signals in the k-space is the Cartesian one. In this case, each signal is recorded along a horizontal line of the k-space. This approach is still the most used today as it provides high spatial resolutions in two and three dimensions. It is also very robust to hardware drifts, and in particular to errors in trajectories related to the execution of gradients. Nevertheless, other ways of sampling the k-space have been developed for accelerated acquisitions. Today, the most common non-Cartesian imaging methods are the radial approaches (Lauterbur 1973). The signal is sampled along radial spokes or diameters of a disk, which provides ultra-short echo time sequences and projection-reconstruction (PR) sequences, respectively. In the case of a 2D sequence, these trajectories cover a disk, while in 3D sequences, they cover a sphere (Figure 3.2). Figure 3.2. Trajectories in the k-space for radial sequences and UTE, in 2D, and 3D. (UTE: ultra-short echo time; PR: projection-reconstruction). Radial imaging techniques have several advantages such as the possibility of using high undersampling factors. In fact, since each radial spoke or diameter acquires the center of the k-space, it is possible to reconstruct an image even with a low number of projections. These sequences are also known to be less sensitive to flow or motion artifacts. On the downside, compared to Cartesian methods, radial sequences often produce images with a lower spatial resolution because the edges of the k-space are undersampled (Figure 3.3). Figure 3.3. k-Space trajectories and the corresponding images. (a) Radial sequences with number of projections corresponding to Nyquist criteria and a weak number of projections (b). The projections have to be uniformly distributed to obtain an image without artifacts (c) Among other non-Cartesian trajectories, the spiral acquisition developed by Ahn et al. (1986) consists of sampling the signal using sinusoidal gradients. Although seldom used in clinical and preclinical routines, this approach presents some advantages that make it robust and very versatile: (1) oversampling of the k-space center makes this method robust to flow and movement artifacts; (2) this type of trajectory acquires the full k-space in a single acquisition (single-shot approach), providing 2D or 3D images very quickly; (3) in the case of a so-called spiral-out trajectory, that is, a spiral trajectory starting from the center of the k-space and ending at the edges, very short or even ultra-short echo times (UTE) can be achieved. Variations of this encoding exist, for example, using Cartesian encoding in the slice-selection direction that permits acquiring spiral planes (Stacks-of-Spirals method) with a cylindric-shaped k-space. Hybrid radial/spiral encoding has also proven useful for abdominal imaging in mice (Castets et al. 2017). This method also presents several disadvantages, such as sensitivity to chemical shifts, to variations of the magnetic field, and to gradients imperfections altering the trajectories (in particular, eddy currents or fluctuations in communication delays). However, these technical obstacles can be overcome owing to recent advances in terms of hardware and MRI methods. For example, spiral acquisitions can be easily combined with different fat-signal saturation modules. Trajectory monitoring methods can compensate for the gradients’ imperfections. Multi-shot encoding can also represent a solution, at the cost of longer acquisition time however. Nevertheless, it has recently been shown that spiral encoding can be combined with parallel imaging (Chen et al. 2016) or compressed sensing methods (Zhao et al. 2015) to accelerate acquisitions. Other methods based on spiral encoding have been developed, such as the twisted projection imaging (TPI) method (Boada et al. 1997b). Due to its very short TE, TPI has been used for the quantification and imaging of sodium in different organs. Similarly, the Cones method (Boada et al. 1997a) samples the signal very quickly and preserves excellent image quality in studies of short-T2* tissues. Finally, the spiral projection imaging technique (Boada et al. 1997a) consists of rotating the spirals by a certain angle around the rotation axis. This method keeps the scan time very short, albeit with lower image quality in terms of spatial resolution and signal intensity in tissues with short T2*. Today, the main method employing spiral single-shot encoding is fingerprinting (Bipin Mehta et al. 2019), which acquires several signals with different acquisition parameters and thus enables the reconstruction of multiparametric maps (see Chapter 10). Wave-Caipirinha (Wave-Caipi) trajectory (Bilgic et al. 2015) is another recent and particularly useful method for accelerating image acquisitions. In this encoding scheme, sinusoidal gradients Gy and Gz (with a phase shift of pi/2 between the two) are simultaneously applied during the readout of each k-space line, thereby creating offsets between the slices by modifying k-space phase and partition encodings. The result is a very efficient sampling model of the k-space that is uniformly filled in all the spatial directions (x, y and z). Given that this approach takes advantage of the spatial variation of the coils’ 3D sensitivity profiles, it enables highly accelerated volumetric imaging with negligible disadvantages in terms of signal-to-noise ratio (SNR). All of these encoding approaches provide images from undersampled k-spaces. In fact, according to Nyquist’s criterion, the sampling frequency has to be twice the highest frequency of the measured signal. In the cases when this criterion is not complied with, artifacts can appear in the final image. Several methods can improve the robustness of random undersampling schemes. Among these, using the golden angle leads to a random k-space filling while significantly reducing artifacts from the magnetic field heterogeneity. Some literature examples have demonstrated the possibility of combining spiral acquisitions with 2D or 3D golden angles thanks to hybrid radial/spiral encoding. Indeed, radial encoding (Lauterbur 1973) is particularly robust to undersampling. The principle of this technique consists of sampling the signal along diameters symmetrically centered at the origin of the k-space. This method oversamples the k-space center. Moreover, it is also very robust to physiological fluid motion, such as blood flow. This encoding enables the use of a sliding window to reconstruct images with a variable amount of raw data (GRASP) (Feng et al. 2014), and thus to reconstruct dynamic (cine) images, that is, resolving the movement of an organ (heart or lungs, for example). In practice, signal acquisition is either synchronized with the movement (prospective synchronization) or performed continuously until all of the k-spaces have been filled (retrospective synchronization). In both cases, the TR of the employed sequence has to be much shorter than the duration of a physiological movement cycle (cardiac or respiratory). The distribution of information is performed after the acquisition by sorting the k-space echoes according to each phase of the physiological cycle. Thus, the number of undersampled images per cycle can be chosen a posteriori. Radial encoding is quite suitable for obtaining dynamic images as it can avoid acquiring signals via external sensors (ECG or respiratory bellows) by recording the signal caused by the movement at each TR (self-gating or auto-synchronization method). This approach is particularly useful in the case of physiological cycle disruptions, such as arrhythmias. Many applications use this versatile acquisition method such as free-breathing acquisitions or first-pass transits of contrast agents, for example. In addition to modifying the main sequence parameters, there are other ways for accelerating scans, such as changing the undersampling of the k-space. Sections 3.4.1–3.4.4 present three types of commonly used undersampling strategies and the corresponding reconstruction approaches (Figure 3.4). Figure 3.4. (a) Typical k-space acquisition strategies with different sampling approaches. (b) k-Transform of the corresponding k-spaces showing the typical artifacts caused by these techniques The partial Fourier (PF) acceleration approach makes use of the k-space’s Hermitian symmetry property: where S is a point of the k-space at kx, ky coordinates and * corresponds to the complex conjugate operation. This relation is valid only when the image has a purely real component, which implies the absence of B0 field inhomogeneities, magnetic susceptibility differences between tissues, etc., which would produce phase variations in the image. In the ideal case, half of the k-space can be acquired and the second half can be retrieved because of equation [3.1], in order to reconstruct an image with good quality. In order to compensate for the phase variations appearing during an MRI scan, a minimum of 60–90% of the k-space has to be acquired (Figure 3.1). This enables the reconstruction of a low-resolution phase image using the central symmetric region of the sampled k-space. This phase estimation is then used to obtain an image where the phase is equal to 0 and is thus purely real, such that Hermitian symmetry can be applied. Several PF reconstruction approaches have been developed: Margosian (Margosian et al. 1986), Homodyne (Noll et al. 1991), as well as the iterative projection onto convex sets (POCS) method (Haacke et al. 1991). A Matlab implementation of these algorithms has been made available by Mark Chiew1. Many variations of these methods exist, concentrating mainly on the combination of PF with parallel-imaging reconstructions (Bydder and Robson 2005; Uecker and Lustig 2017; Kettinger et al. 2019) or on the estimation of a higher resolution phase image (Koopmans and Pfaffenrot 2021) which reduces the artifacts in the regions with large phase variations. This acceleration method is used very often as it can be applied in 2D or 3D and it reduces the acquisition time by 20–40%. Another possibility consists of applying partial Fourier along the readout direction (rather than along the phase direction) to reduce the sequence’s echo time. Figure 3.5. Image reconstruction using the POCS algorithm. Improvements in spatial resolution of the magnitude image are visible but the phase image has a resolution equivalent to a zero-filled image The main PF’s limitations are the low SNR, due to the reduction of the number of acquired lines, and the appearance of artifacts in the images whenever rapid phase variations are present (e.g. at tissue/air or tissue/biomedical material interfaces, etc.). Furthermore, this approach is not recommended for applications that require phase information such as susceptibility or temperature mapping (Figure 3.5). The improvement of MRI hardware and, in particular, the use of phased arrays with coils spatially distributed on the sample have enabled higher SNRs by reducing the field of view of each coil element. This led to the idea that fully encoding the phase direction via magnetic field gradients is not strictly necessary, giving birth to the so-called parallel imaging method. Parallel imaging consists of accelerating MRI scans by skipping some lines of the k-space (Figure 3.4). When images are reconstructed from such an incomplete k-space, filling artifacts appear. These artifacts can be explained by the following property of the k-space:
3
Fast Imaging and Acceleration Techniques
3.1. Introduction
3.2. Definition of fast imaging
3.3. Fast accelerated sequences
3.3.1. Sequence optimization
3.3.2. Turbo spin echo and echo-planar imaging
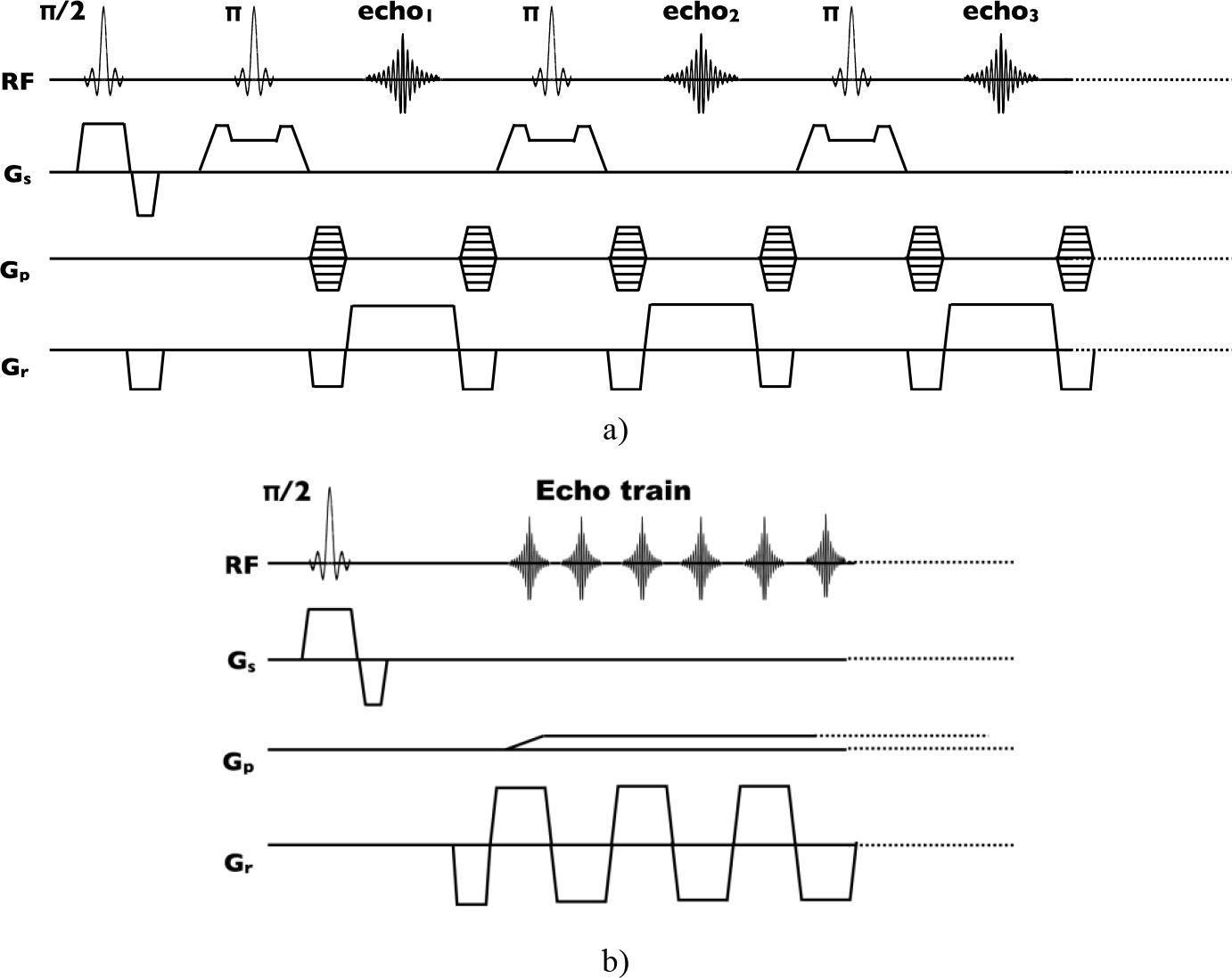
3.3.2.1. Echo train concept
3.3.2.2. Notion of contrast manipulation in RARE
3.3.3. Non-Cartesian methods

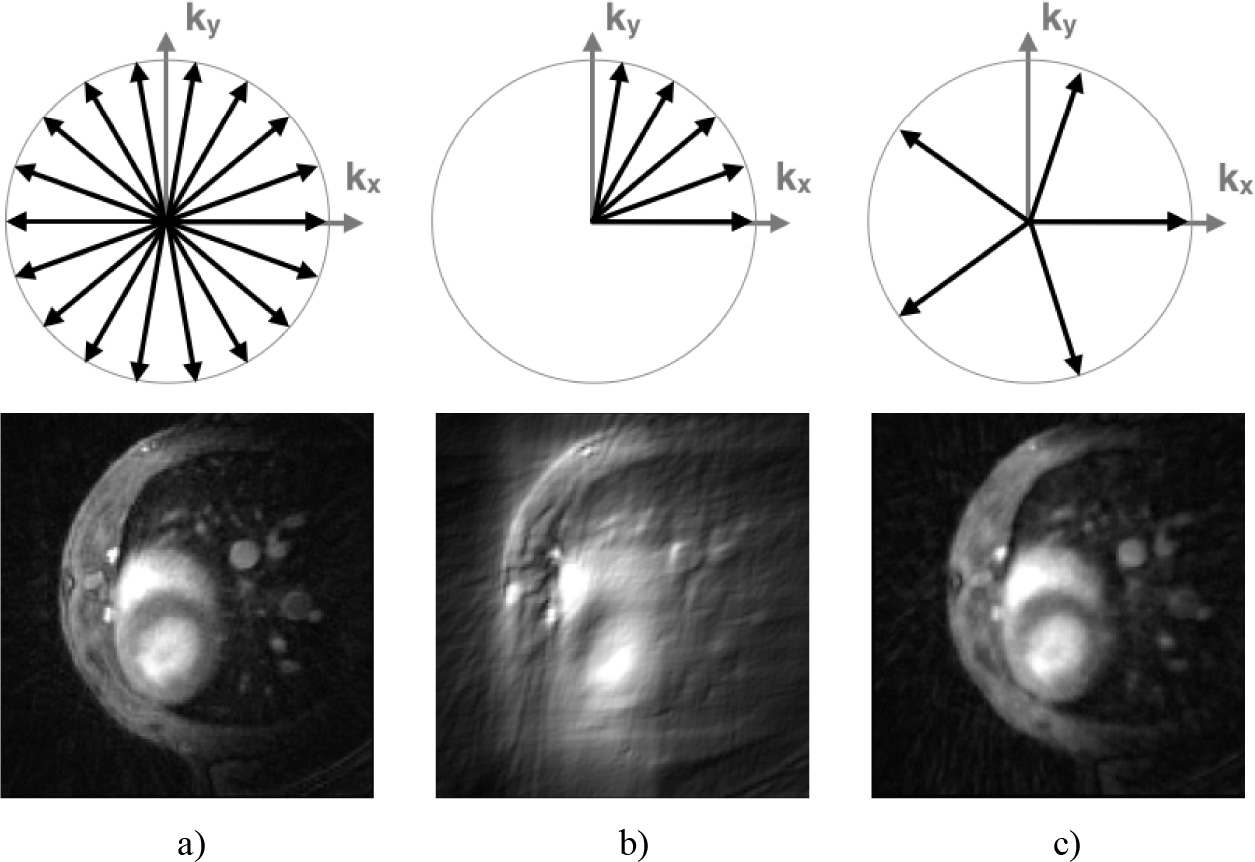
3.4. Acceleration methods
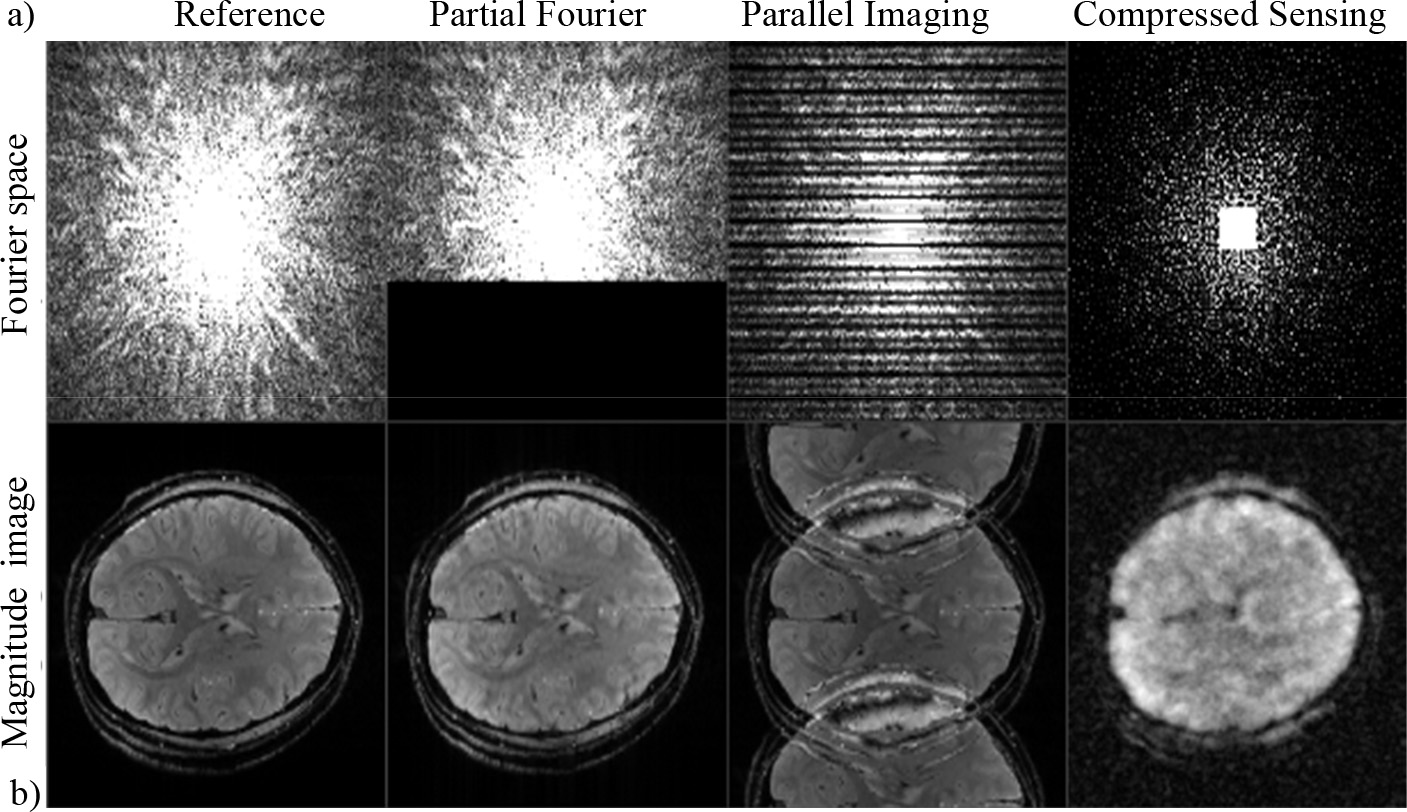
3.4.1. Partial Fourier
3.4.2. Parallel imaging
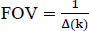
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree