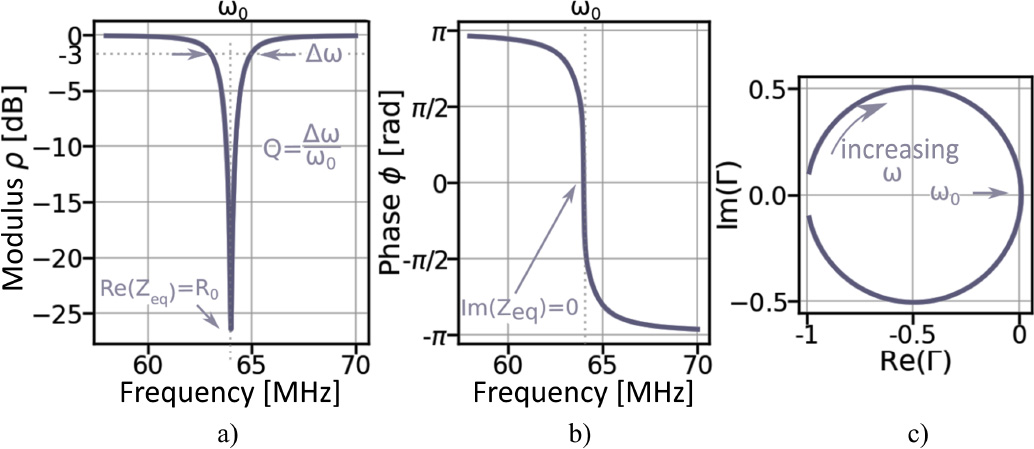
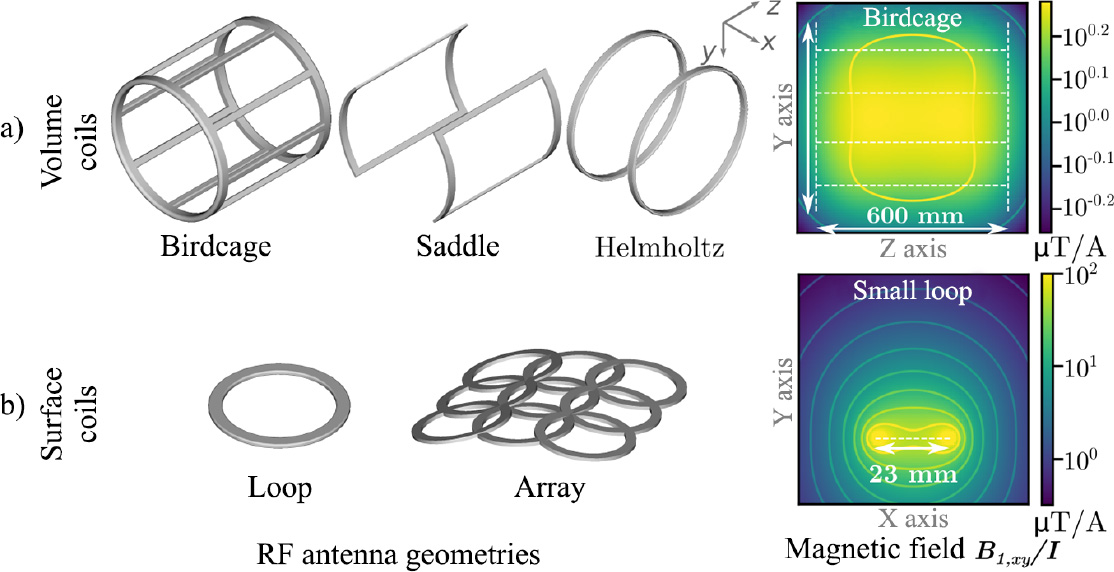
Aimé LABBÉ and Marie POIRIER-QUINOT BIOMAPS, CNRS, Inserm, CEA, Université Paris-Saclay, France A radiofrequency (RF) antenna is most fundamentally a resonant circuit designed to transmit and sense energy in the form of electromagnetic radiation. Nevertheless, in practice, the term “antenna” includes devices with completely opposite design goals. Under the same label, we can find radio broadcasting towers, which are rectilinear structures up to hundreds of meters tall, sensitive to the electric component of the electromagnetic fields and designed to emit energy in all directions to cover as wide an area as possible. On the other hand, the same term can be associated with MRI coils, which can have a loop shape of a few centimeters in diameter, are sensitive to the magnetic field component and aimed at concentrating the wave energy in a small volume and minimizing the radiated power far from the device. In addition to these functional differences, an RF coil can also be considered from several different points of view. For an MRI researcher, an RF coil is, above all, a tool for inducing (transmitting) and then collecting (receiving) a magnetic resonance signal that is required to obtain an MR image, without regard for the detailed functioning of the detector (Figure 2.1(a)). For an electronic engineer, an RF coil is an electric circuit composed of various electronic components: conducting wires, capacitors, diodes, etc., whose behavior is described by electrical circuit theory (Figure 2.1(b)). According to Maxwell’s equations, an antenna is a source of a magnetic RF field with a desired spatial distribution that is determined by the detector’s construction and properties (Figure 2.1(c)). Figure 2.1. Example of a “birdcage” RF coil. COMMENT ON FIGURE 2.1.– The coil structure is visible in (a) with (b) conducting wires and various electronic components, which can be modeled as an equivalent electrical circuit and generate (c) a magnetic RF field B1. In this chapter, the MRI antenna, which will be interchangeably referred to as sensor, detector and coil, will be presented to an audience of researchers from the point of view of an electronic engineer. Therefore, the chapter’s goal is to provide a general understanding of the fundamental concepts regarding RF coils. After recalling some fundamental notions of resonant circuits implemented in MRI, we will consider the RF coil as a generator of an RF magnetic field B1. Afterward, we will see how an RF coil is used in transmission first and in reception later, together with the main considerations involved in the design and characterization of coils for these two operation modes. In addition, we will tackle the decoupling challenges and safety issues regarding RF coils used in MRI. Finally, more advanced topics and recent developments of RF coils for some applications, in particular for high and low magnetic fields, will be presented. An RF coil can be modeled as a closed circuit composed of impedances with a reactive part made up of an inductance Lc in henry (H) and a capacitance Cc in farad (F) and a resistive component Req in ohm (Ω) related to the losses in the circuit, in particular due to the coil resistance Rc and the sample load Rs, as schematically represented in Figure 2.2. In this circuit, energy is alternately stored as electric energy in the capacitance and as magnetic energy in the inductance. The energy emitted by the RF coil originates from the coil’s inductance, whose value is determined by the geometry of the coil’s conducting wire. If assembled correctly, such a circuit can generate or detect an RF magnetic field B1 oscillating at a characteristic frequency ω0, which is defined as: Figure 2.2. Equivalent circuit of an RF coil COMMENT ON FIGURE 2.2.– The equivalent model of an RF coil in which the NMR signal induces an emf voltage is represented on the left of the reflection coefficient Γ; Lc, Cc and Rc are the main components of the coil, while Rs is the load resistance of the sample. Q · fem is the voltage across the capacitance, with Q being the quality factor. The RF coil presents an impedance Zc in input to the variable circuit used for frequency tuning and impedance matching to the RF signal channel with impedance Z0. Zeq is the impedance of the RF coil seen from the output of the matching circuit. For an efficient coil, the angular frequency ω0 must be equal to the Larmor frequency: where we recall that B0 is the static magnetic field strength and γ is the gyromagnetic ratio, a coefficient that depends on the considered nucleus. For most clinical studies focusing on MRI of protons 1H, this frequency corresponds to a resonant frequency ν0 = ω0/2π of 64 MHz at 1.5 T and 128 MHz at 3 T, which are both in the range of RF waves (between a few kHz and a few GHz). Besides its resonant frequency, the coil is also characterized by its spectral bandwidth Δω, that is, the range of frequencies in which the coil can efficiently transmit or receive an RF signal1, which defines the quality factor Q of the coil: From theory, the quality factor can be interpreted as the ratio between the energy stored for each cycle in the resonator (∝ ω0L) and the power dissipated by its resistance (∝ R). In practice, when an NMR signal induces a voltage emf in volts (V) in the coil, the voltage across the capacitance Cc will be Q · emf (see Figure 2.2). Since the coil is part of the RF signal channel of an MRI system, in addition to frequency tuning, it is also often necessary to perform the coil’s impedance matching2. The goal of this step is to optimize the power transmission between a source and a load. When an MRI coil is used in transmission, the matching condition ensures that the amplitude of the B1 field produced by the coil is maximized; in reception, impedance matching optimizes the power transmission of the NMR signal3. Since the impedance is intrinsically complex, a coil is said to be matched when its impedance Zc is transformed into an equivalent impedance Zeq thanks to a transformation circuit, which we call the tuning and matching circuit (see Figure 2.2). Additionally, Zeq = In practice, frequency tuning and impedance matching are generally linked and both are done via a matching circuit. The design of such a circuit can be more or less complex (Mispelter et al. 2015), but in general it involves (1) discrete reactive (capacitive and sometimes inductive) components of fixed value, carefully chosen after analyzing the coil’s electrical circuit (they provide an approximate starting coil matching), as well as (2) variable components allowing for fine tuning and matching adjustments. Often, coil matching operations and characterization measurements are performed by means of a vector network analyzer. This instrument measures the frequency dependence of the coil’s reflection coefficient Γ: where ρ and ϕ are the norm and phase of the reflection coefficient, respectively. To obtain this parameter, once the analyzer is connected to the coil via a port with a characteristic impedance Z0, the instrument sends an incident RF voltage Vinc toward the coil and measures the reflected wave Vref at the same port. The ratio of these two quantities defines the reflection coefficient Γ, which is also called S11 in literature. Supposing that Z0 = R0 is real, it is possible to express a relation between Γ and the equivalent impedance Zeq of the coil and its matching circuit: Typically, for a tuned and matched coil, the reflection coefficient Γ exhibits the shapes shown in Figure 2.3. Close to the resonant frequency, that is, when |ω − ω0| ≤ Δω, Zeq ≈ R0 and the reflection coefficient tends to zero, which corresponds to the incident wave being entirely transmitted. On the other hand, for |ω − ω0| ≫ Δω, Zeq is completely unmatched and the incident wave will be entirely reflected with ρ = 1 (or 0 dB in Figure 2.3(a)) and a phase opposition ϕ = ±π. The coil tuning and matching operations consist of adjusting the variable parameters of the tuning and matching circuit such that the modulus ρ is minimized and its phase ϕ is canceled out at ω = ω0, which is equivalent to canceling out the reactive part of Zeq (i.e. Zeq becomes purely real) and achieving Zeq = R0. From the modulus ρ(ω), it is possible to measure the coil’s bandwidth Δω and to calculate from its value the quality factor Q. Under the hypothesis of a tuned and matched coil, the bandwidth corresponds to the width at half-peak of the resonant response ρ(ω). If ρ(ω) is displayed in dB (which is generally the case), then Δω corresponds to the width at –3 dB with respect to the baseline of ρ(ω), as shown in Figure 2.3(a). The use of a network analyzer is rather common for characterizing these electrical properties. The precision of this method mostly depends on the instrument’s quality, in particular on its calibration, and care taken in choosing its settings for each measurement. Figure 2.3. Frequency dependence of the (a) modulus ρ and (b) phase ϕ of the reflection coefficient Γ = ρ exp (iϕ). c) Real and imaginary components of Γ for different frequencies From the point of view of signal reception, the issue of tuning and matching is more critical than for transmission. Indeed, in receive operation, the weak free induction signal of the coil has to reach the input of a low-noise preamplifier in an optimal way. As an example of the orders of magnitude, in the case of a 40 mm3 volume of water at 1 T, the amplitude of the electromotive force induced in a five-turn solenoid coil, 4 mm in diameter and 1 mm long, is of the order of 100 μV. In the context of an MRI scan, the RF coil is coupled to the patient via its magnetic field. Therefore, understanding the properties of the B1 field is fundamental for choosing a coil for a desired application. Let us remind you that a magnetic field is a vector field with an amplitude and orientation that potentially vary in space and over time. The behavior of electromagnetic fields is fully described by Maxwell equations. In practice, some parameters have a greater effect than others on the properties of this field. The first and foremost influencing aspect is the geometry of the coil used to generate the magnetic field B1 because this field is produced by the circulation of an electrical current with an intensity I in amperes (A) within the conducting wire of the coil. In MRI, a few common geometry shapes are encountered, some examples of which are presented in Figure 2.4. Usually, they are classified into two categories: (1) volume coils and (2) surface coils, depending on whether the coil is positioned around or on the patient, respectively. The choice of the type of coil is restricted by the geometrical constraints of an MR scan and by the orientation of the RF field B1 generated by the coil, which has to remain perpendicular to B0 in order to be useful. In addition, Figure 2.4 compares the transverse component of the magnetic field B1,xy per unit current I produced by a volume coil and a surface coil. Volume coils mostly create homogeneous magnetic fields, which is not the case with surface coils. On the other hand, a surface coil provides a higher amplitude of the magnetic field, as shown in Figure 2.4. To give an estimation, for a given current, a surface coil of 23 mm produces magnetic fields a hundred times stronger than a volume coil of 600 mm in diameter. Figure 2.4. Volume and surface coils. COMMENT ON FIGURE 2.4.– (a) Main RF coil geometries used in MRI. Here, the B0 field is oriented along the z-axis and the B1 field is produced along the x-axis for the volume coils and along the y-axis for the surface coils. (b) Comparison of the transverse components B1,xy/I of the magnetic field created by a volume coil and a surface coil. The variation between two isolines corresponds to dividing the amplitude of B1,xy/I by a factor of two. The white dashed lines indicate the projection of the coil’s geometry on the field map. A second important aspect is the wavelength λ in meters (m) of the magnetic signal produced or received by the coil: where ν = ω/(2π) is the frequency in hertz (Hz) and c = (μϵ)−1/2 is the wave propagation speed in a medium, which depends on the medium’s magnetic permeability μ and electric permittivity ϵ. Table 2.1 provides values of wavelengths for a few important media in MRI at 1.5 T and 3 T. The wavelength affects the propagation of a signal by modifying its phase in space and potentially distorting the magnetic field. In the coil’s conducting metal, propagation effects modify the current distribution in the wires. In tissues, where the wavelength is typically 10 times shorter than in air, this can lead to interference phenomena that can locally increase the magnetic field amplitude, as shown in Figure 2.5, and represent a potential safety risk by increasing the local specific absorption rate (SAR). The challenges of safety related to RF coils will be described in more detail in section 2.6. These effects become critical whenever the dimensions of the cables and imaging volume are not negligible with respect to the wavelength, which is the case of high frequencies, that is, at high static magnetic field B0. In practice, propagation effects can be ignored when the characteristic lengths of a system are shorter than λ/10. Approaches for reducing or removing propagation effects will be presented in section 2.6.2. Table 2.1. Wavelength λ in different propagation media Figure 2.5. Maps of local variations of the magnetic field B1 in a human abdomen at three different operating frequencies. A reference with a homogeneous magnetic field is provided. Figure inspired by the works of Cloos et al. 2016, under the license CC BY 4.0. Finally, the tissue electric conductivity σe in siemens per meter (S m−1) can also modify the magnetic field. As a response to an incident alternating magnetic field, eddy currents are created in conductive tissues. In turn, these currents induce a magnetic field that opposes the incident magnetic field. As a result, the magnetic field cannot efficiently penetrate into the tissues and remains confined to the surface with a characteristic depth δ, called the skin depth. While these effects are not critical at intermediate magnetic fields, they can become problematic at higher fields. For example, considering the electric properties of muscle at 1.5 T (intermediate field) and at 11.7 T (ultra-high field) (Gabriel et al. 1996), the skin depth of the RF wave changes from 20 to 7 cm at the respective fields. Skin depth will be discussed in more detail in section 2.4.1. The concept of polarization is employed to describe the temporal evolution of the amplitude and orientation of the magnetic field B1 produced by the coil. Two limit cases can be considered: the linear and circular polarizations, as depicted in Table 2.2. In the case of linear polarization, the wave vector is periodic in time, while its orientation is constant (up to a sign). On the other hand, circularly polarized waves have a constant modulus while their orientation rotates in a plane. In between these two cases are the “elliptical” polarizations. Table 2.2. Description of linear and circular polarizations Another useful representation considers the polarization of B1 with its components Initially, most MRI coils emit waves with linear polarization. To obtain a circular polarization, one requires a coil or a combination of coils that produces two orthogonal components of the magnetic field (along x and y) phase-shifted by π/2, one with respect to the other. The coil is said to be used in quadrature. Circular polarization is typically found in volume coils. In particular, “birdcage” coils are specifically designed for such a purpose. When powered at a single point, a birdcage coil produces a linearly polarized B1 field, which becomes circularly polarized when two input points are controlled in quadrature. Finally, anything that is capable of modifying the amplitude and phase of the wave in space, that is, coil geometry, propagation effects, as well as tissue shape and conductivity, will change the polarization of the coil’s field. In such conditions, elliptical polarization will usually occur as an intermediate case between linear and circular polarizations with non-zero In MRI, the RF coils are recruited for two roles: generating (transmission) and acquiring (reception) the magnetic resonance signal. Using two separate coils for transmission and reception is a very common configuration in clinical imaging. Such an approach offers the possibility of separately designing two optimized circuits that fulfill their respective role. During transmission, the magnetic field B1 with a frequency ν0 created by an RF coil flips the nuclear spins of the scanned subject to the transverse plane. The magnetization flip angle α (in radians) is:
2
Radiofrequency Coils: Theoretical Principles and Practical Guidelines
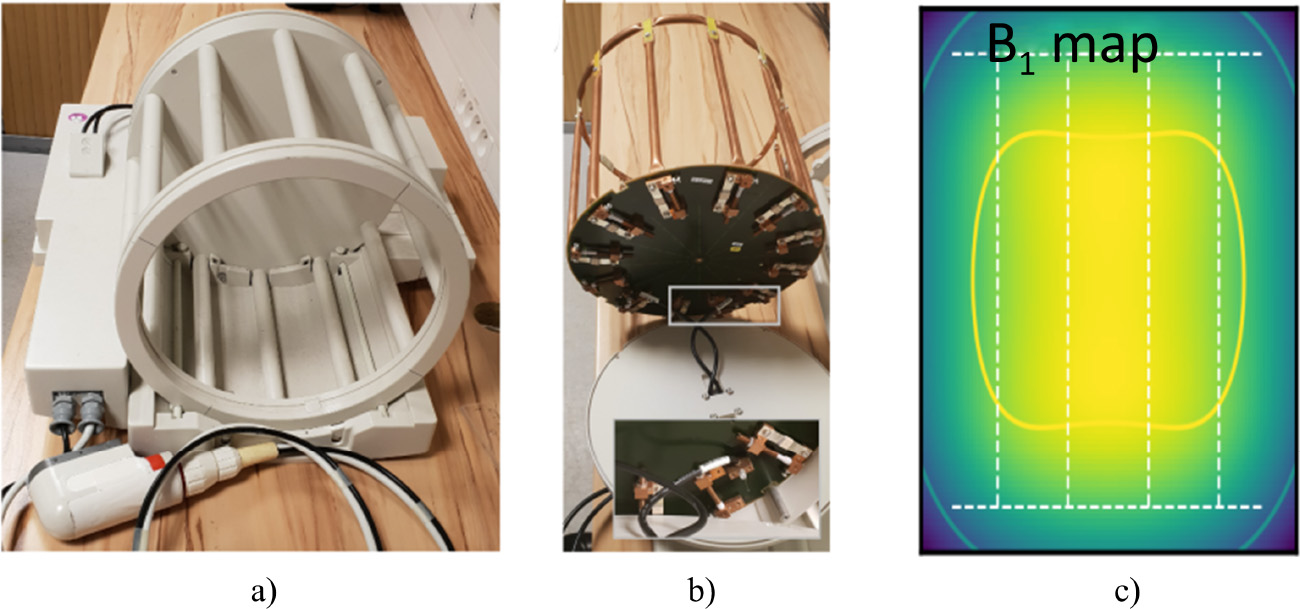
2.1. Coil as an electrical resonant circuit
2.1.1. Basic concepts
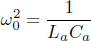
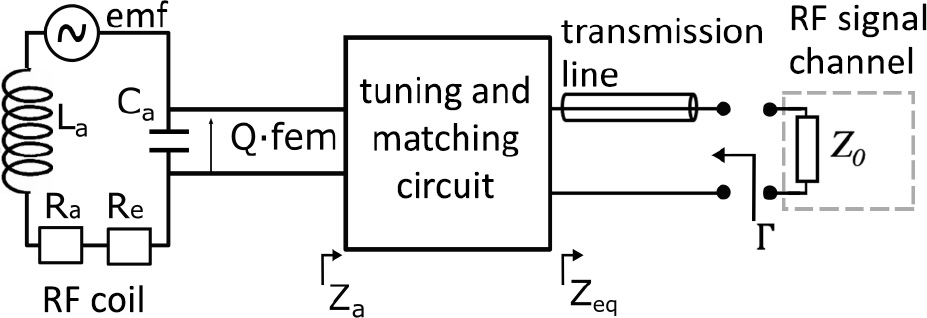
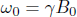
2.1.2. Coil tuning and matching
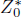, where 
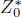is the complex conjugate of the characteristic impedance of the RF signal channel in the MRI system. Most often, Z0 is purely resistive, for example, Z0 = R0 = 50 Ω.
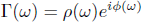
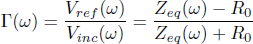
2.2. Coil as a source of a magnetic RF field
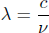
Frequency (B0)
Air
Coaxial cable
Brain
64 MHz (1.5 T)
4.7 m
3.1 m
0.50 m
128 MHz (3 T)
2.3 m
1.5 m
0.25 m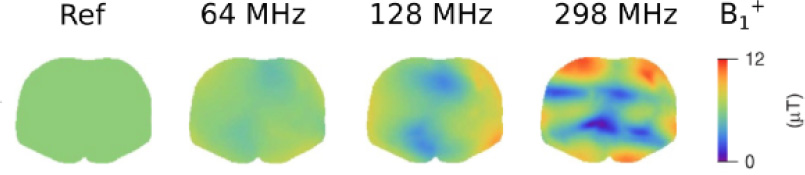 
2.2.1. Polarization and
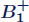and 
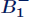fields
Type of description
Linear polarization
Circular polarization
Schematic representation
B1(t)= Bx(t)x + By(t)y
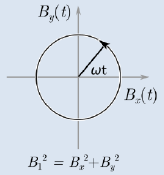
Decomposition into linear polarizations
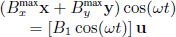
B1 cos(ωt)x + B1 sin(ωt)y
Decomposition into circular polarizations
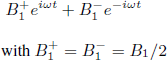
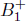and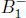 
, which are two circularly polarized waves rotating in opposite directions with angular frequencies +ω and −ω (Hoult 2000). In practice, the importance of this distinction lies in the fact that the 
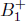component of the transmit coil is the only one actually contributing to the flipping of the magnetization during transmission. On the other hand, the 
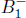component contributes to the undesired increase in power deposited in the tissues, and thus to the increase in the SAR. In the case of a linearly polarized coil, half of the RF power is lost via the 
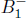component. Therefore, such a configuration has a relatively low efficiency in MRI. For circularly polarized coils, all the RF power of the coil field is useful and conveyed via the 
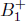component. In the same way, since the magnetic resonance signal is circularly polarized, half of its power is also lost when it is acquired using linearly polarized coils.
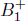and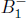 
and such that 
≠ 
.
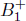2.3. Transmit coil
Related posts:
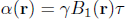
Stay updated, free articles. Join our Telegram channel
Full access? Get Clinical Tree
