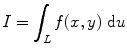(1)
Radiation Monitoring Devices Research Nuclear Medicine, Water town, Massachusetts, USA
Abstract
Mankind from the prehistoric age has admired crystals. However, the significance of that beauty for the engineers and the scientists is on the basis of structural symmetry, simplicity, and purity. These characteristics endow crystals with unique physical and chemical properties, which have been used to cause a major transformation of the electronic industry. The systematic growth of synthetic crystals might be viewed as an art rather than science and has been described by some experts in this field as a new agriculture. The list of the synthetic crystals grown in the laboratory is far from exhaustive, but it shows how several old substances develop unique properties when they have the form of crystals. Figure 6.1 shows the many facets cut in a synthetic diamond crystal—a highly refractive, colorless crystalline allotrope of carbon.
6.1 Introduction
Mankind from the prehistoric age has admired crystals. However, the significance of that beauty for the engineers and the scientists is on the basis of structural symmetry, simplicity, and purity. These characteristics endow crystals with unique physical and chemical properties, which have been used to cause a major transformation of the electronic industry. The systematic growth of synthetic crystals might be viewed as an art rather than science and has been described by some experts in this field as a new agriculture. The list of the synthetic crystals grown in the laboratory is far from exhaustive, but it shows how several old substances develop unique properties when they have the form of crystals. Figure 6.1 shows the many facets cut in a synthetic diamond crystal—a highly refractive, colorless crystalline allotrope of carbon.
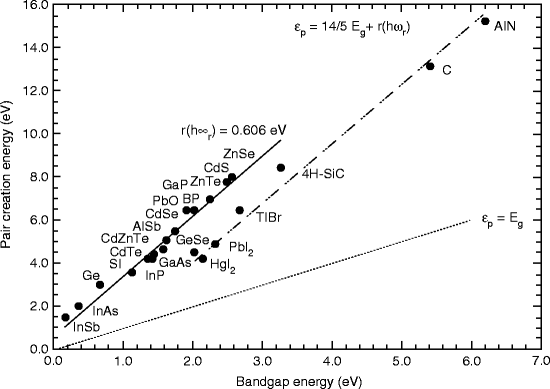

Fig. 6.1
Picture of a many facets cut diamond crystal—a symbol of bond (love?)
Diamonds feature more predominantly than any other gemstone in the history and cultural heritage of the human race. They were prized for their scarcity for centuries and still remain a symbol of wealth and prestige to this day [1].
Figure 6.2 shows a natural diamond approximately 35 × 29 mm in size embedded in the kimberlite host rock. From the figure, we can see numerous visible eyes in the kimberlite rock similar to olivine crystals called chrysolite.


Fig. 6.2
A natural diamond approximately 35 × 29 mm in size embedded in the kimberlite host rock (Photo courtesy: Gem and Mineral Miners Inc.)
Diamonds were first mined in India over 4,000 years ago, but the modern diamond era only began in 1866 [1]. But recently, a scientist named Tracy Hall of General Electric (GE) is the first person to report of synthesizing diamond in the laboratory. The crystals were grown in the GE laboratory, from a solution of graphite in metal solvents such as nickel at a very high pressure (between 50,000 and 70,000 AP) and temperature (about 1,700 K).
Recently, at the University of Florida, a pilot plant has been established to grow commercial grade diamond using BARS diamond growth equipment. The largest crystal examined to date is a 10-carat, half-inch thick single-crystal diamond manufactured by Carnegie Institution’s geophysical laboratory.
From time immortal diamond has been a fascinating gemstone. It has been used as an expression of beauty, love, and wealth, which has led to enormous amount of scientific research. As a result, besides being available in the market as a natural single-crystal gemstone, polycrystalline thin films of diamond have been successfully grown by plasma-enhanced chemical vapor deposition (PECVD) method. These films are very much in use as scintillator in high–energy physics and in high–radiation environments, such as hadron colliders and radiation–hard sensors [2–4]. The possibility of fabricating diamond detectors has been enticing research for decades. It is extremely rugged and possesses highest thermal conductivity at room temperature. On the other hand, the wide band gap (~6.6 eV) of diamond provides a low dark current—an important criterion for radiation detectors that are used in medical imaging [5–7].
Threat reduction, nuclear nonproliferation, and medical imaging activities require improved radiation detectors. The performance of these detectors can be significantly enhanced if the materials currently entrusted with the detection of radiation are placed with optimized materials. In order to gain a unique expertise knowledge about the requisite optimization of the radiation detector materials, one has to study the theory and nucleation of the growth of the materials either in the form of a crystal, a polycrystal, or in the form of a thin or a thick film.
6.2 Theory of Crystal Growth
Crystals are said to be the ordered arrangements of atoms (or molecules). In crystalline solids, the atoms are arranged in a periodic fashion and when this periodicity is seen to exist throughout the entire solid, the solid material is defined as being formed a single crystal. The crystal growth process starts with the nucleation stage. Several atoms or molecules in a supersaturated vapor or liquid start forming cluster. When the cluster is having a critical radius r, which is also defined as the critical energy barrier ΔG, the radius starts to grow larger and eventually forms a crystal. From the standpoint of thermodynamics, we can express this critical energy barrier ΔG as
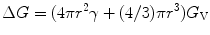
where γ is the surface tension and G V is free energy change per unit volume forming the stable solidification from vapor or liquid.
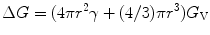
(6.1)
Figure 6.3 shows the

schematic of free energy vs. crystal–melt interface during attachment. Now, the free energy difference between the melt and the activated state ΔG′ can be related to molecular attachment M a as
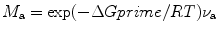
where R is the gas constant, T is the temperature in degree Kelvin, and ν a is the rate of molecular attachment. Similarly, we can write the rate of molecular detachment (M d) as
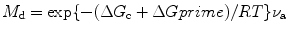
where ΔG c is the free energy difference between the melt and the crystal. Now, we can define the growth rate G r as the difference of these multiplied by the thickness per molecular layer d m and the fraction of the sites on the crystal surface available for attachment f [8]. Thus, the growth rate G r can be expressed mathematically as
![$$ {G_{\mathrm{ r}}} = f{d_{\mathrm{ m}}}\exp ( - \Delta Gprime/RT){\nu_{\mathrm{ a}}}[1 - ( - \Delta {G_{\mathrm{ c}}})/RT] $$](/wp-content/uploads/2017/03/A308734_1_En_6_Chapter_Equ00064.gif)

Fig. 6.3
Schematic of free energy vs. crystal–melt interface during attachment
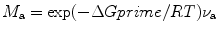
(6.2)
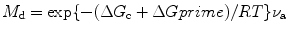
(6.3)
![$$ {G_{\mathrm{ r}}} = f{d_{\mathrm{ m}}}\exp ( - \Delta Gprime/RT){\nu_{\mathrm{ a}}}[1 - ( - \Delta {G_{\mathrm{ c}}})/RT] $$](/wp-content/uploads/2017/03/A308734_1_En_6_Chapter_Equ00064.gif)
(6.4)
Now, when the cooling rate is small, we can write ΔG c as

where ΔH c is the latent heat of crystallization and T L is the liquidus temperature.

(6.5)
Again, Stokes–Einstein relation for diffusion coefficient D can be expressed mathematically as
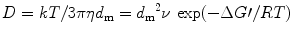
where η is the viscosity of the melt and ν is an attempt frequency. Finally, we can write the growth rate G r of the crystal as [9]
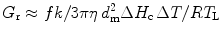
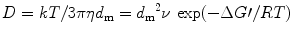
(6.6)
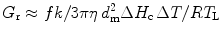
(6.7)
There are two broad categories of crystal growth mechanisms, namely, the lateral and continuous. These two growth mechanisms have been identified as the main growth techniques. The continuous growth rate is determined by the nucleation rate and the rate of layer spreading [10, 11].
Detailed studies of the growth mechanisms of the crystals confirm that the crystal growth is a process coupled with fluid dynamics, heat and mass transfer, surface kinetics and morphology, phase transitions, and chemical reactions. Nevertheless, it is very important to know the thermal history of the growing crystal, in order to improve the crystal quality and process yield. It is also true that the temperature distribution in the growing crystal has a dominating influence on the formation of thermal stress, which can ultimately lead to plastic deformations and initiation of defects of structures.
6.2.1 Factors Affecting Crystal Growth
The rapid advance in information processing is one of the greatest achievements in modern technology as well as human history. It has been accomplished through the development of devices that are being in use in different fields, and they are built on single-crystal wafers. Therefore, the growth of single-crystal wafers is regarded as the key foundation underlying these innovations.
Almost all crystal growth experiments involve phase reactions. It is the objective to grow a single phase, namely, single crystal, from some other phase, a liquid or gas, which may have the same composition or may have a composition quite different from the final product. Therefore, successful growth from phase requires knowledge of the thermodynamic equilibria among the reacting vapor constituents, which may involve species other than the initial gaseous species. The kinetic of growth is critically dependent on the concentration or the diffusion of the desired reacting species. Vapor phase growth can proceed either through reversible or irreversible reactions. In reversible reaction, the solid material can be brought into vapor phase and then reformed under controlled conditions by reversing the direction of the reaction  . On the other hand, irreversible reaction will proceed only one way
. On the other hand, irreversible reaction will proceed only one way 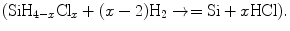
 . On the other hand, irreversible reaction will proceed only one way
. On the other hand, irreversible reaction will proceed only one way 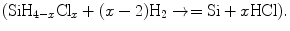
During the reversible or irreversible reaction, there are three primary modes of heat transfer that are considered to be present during the growth of a crystal. These are radiation, conduction, and convection.
Crystal growth melts are usually heated by radiation. Heat losses also occur by radiation from the surface of the growing crystal and/or from the free melt surface itself when not completely contained by the growing crystal. The two most important factors in radiative processes are the temperatures of the emitting and the absorbing surfaces and their geometric positions relative to each other.
The conduction–radiation process mostly controls solidification of the crystal. Considering the thermal conductivity, the steady-state energy balance can be modeled following temperature distribution in the melt. The mathematical expression can be expressed as
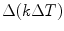
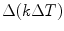
(6.8)
As the above process is coupled to radiation–conduction transport and accounting radiative heat flux in a particular medium, the above Eq. 6.8 will be modified to
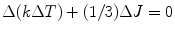
Or,
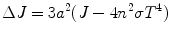
where J is the mean irradiance and (1/3) ΔJ acting as an additional volumetric heat source due to radiation and being used in energy equation [12, 13].
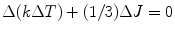
(6.9)
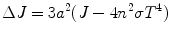
(6.10)
Due to inherent complexities associated with conduction–radiation transfer calculations such as long-distance nature of radiation (solid-angle interaction) and functional dependence of conduction–radiation properties, conduction–radiation transfer calculations are very often simplified. Consequently, simplification or neglect of detailed conduction–radiation analyses may inaccurately predict the temperature field, interface shape/location, and thermal stress distribution within the growth furnace [14]. A nonlinear heat conduction process has been attributed for a crystal growth from liquid melt [15].
Convection or the fluid motion, on the other hand, is due to natural or thermal convection where fluid motions are caused by the action of gravity field on density gradients in the fluid. It may also be thought due to the orientation of the heating or cooling surfaces with respect to vertical direction of gravity which provides an important source of enhancing mass transfer and accounts for the growth rates in crystal. Therefore, the interrelations of convective flows with compositional segregation effects have been thought as the most important part of the crystal growth especially for the melt growth where solid compositional profiles can be most easily measured. Another basic criterion of convection process on compositional profiles in growing crystals is the distribution of the solute along the growth direction, which occurs during the unidirectional solidification of the stirred melts.
The important aspects of crystal growth configurations with regard to thermal convection are (i) the confined nature of the fluid flows and (ii) the presence of vertical and non–vertical heat flows that depend on aspect ratio of the boundary walls. In addition to these two, there may be some forced convection introduced by the rotational field of the growing crystal and rotation of the fluid or melt around crystal.
The other convection-related phenomenon is surface tension due to temperature and compositional gradients that are produced during growth process at the contact point of the melt between the crystal and the container. The float zone growth of silicon is considered to be the predominant mode of convection. Above all, convective process can produce a wide variety of spatial and temporal patterns of transport rate that can be the source of undesirable macro- and micro-segregation patterns in the growing crystal [16].
6.3 Theoretical Modeling of Growing Single Crystal/Polycrystal Used in Radiation Detection and Medical Imaging
Most of the bulk single crystals are grown from solution or melt, where either solutal or thermal or both gradients are present. Although the solution growth has dominated in the field of single crystal growth, melt growth has also been very popular in semiconductor industry. The melt growth has been grouped mainly into three categories, namely, floating zone (FZ), Czochralski (CZ), and Bridgman–Stockbarger (BS) [17].
6.3.1 Floating Zone (FZ) Method
The method that is adopted for floating zone single-crystal growth is essentially a zone melting system in a vertical direction without a seed. The main advantage of the float zone process is that it is a containerless process, which can completely or partially eliminate contamination associated with the flux carrier. Another advantage is the reduction of thermal stresses that are caused by differential thermal expansion between the crystal and the crucible [18]. The FZ process is extensively used for the growth of silicon (Si) single crystal with a very low oxygen concentration. It is also used for high-purity crystals of metals. For refractory metals (with very high melting temperatures), this method is unique since virtually no suitable containers exist for these materials and the chance of contamination from the container does not exist. Figure 6.4 shows the schematic of a floating zone crystal growth apparatus. Floating zone’s unique advantage is the elimination of the melt container, which is invariably a source of contamination. The quartz ampoule is loaded with the source material. Near the middle is an additional heater is called the zone heater to add additional heat to the source. Outside is the main heater, which consists of the furnace frame.
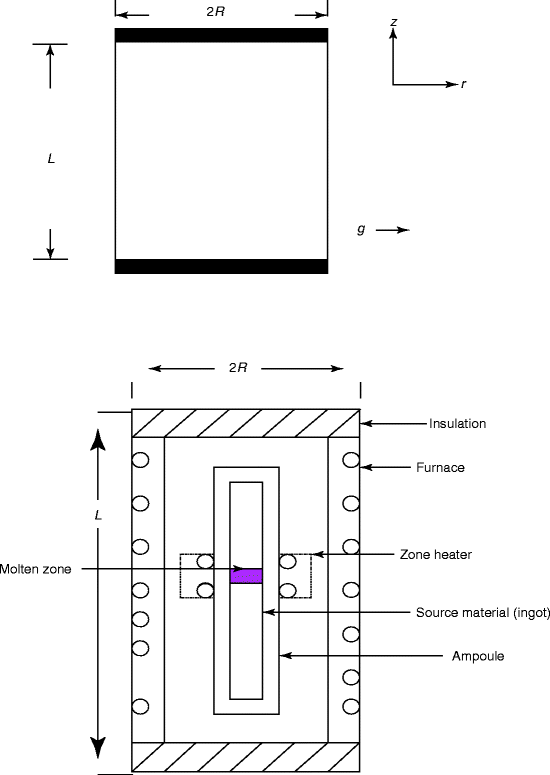

Fig. 6.4
Schematic of a floating zone crystal growth procedure. g gravity acceleration, z, r vertical and radial coordinates
Figure 6.4 shows the schematic of a floating zone crystal growth process. In the case of differential pulling, the crystal is pulled from a molten zone of the feed material. The zone remains stationary while the feed material is advanced upward. Floating zone disadvantage is that it does not lend itself to the growth of the material with high vapor pressure or materials with volatile constituents. From an economic perspective, floating zone (FZ) growth is more expensive than Czochralski (CZ) method.
In a literature, the floating zone method has been modeled as a cylindrical liquid bridge of radius R and length L hold between two disks (Fig. 6.4). It is assumed that a laminar incompressible fluid is present in presence of thermal fluctuation forces. As a result one can expect different steady thermo-capillary flows due to the variation of different parameters like temperature gradient conditions and surface tension gradient [19].
6.4 Growth of a Crystal on a Seed
Crystal growth is a complex process and it is sometimes called a state of the art because of an unbridged gap between theory and experiment. The crystal growth is influenced by several factors particularly when controlled chemical composition and homogeneity and crystalline perfection, orientation, and size are considered. There is no unique method for growing a single crystal of a material. As such there are several methods that have evolved during the course of experimental research. As a rule of thumb, the materials that have low melting points (<1,000 °C) can be grown by less expansive methods like Bridgeman–Stockbarger (BS) rather than Czochralski (CZ) method.
The advantages of control over orientation, growth rate, perfection, and doping make growth on a seed an attractive improvement over growth by random nucleation. Growth on a seed in a cool part of a system while excess solute (often called nutrient) is in contact with the solvent in a hotter region of the system. The method is often called growth in a thermal gradient and is in some respects analogous to hydrothermal gradient growth. The seed is mounted at the end of a platinum-sheathed ceramic rod (which is capable of withstanding the central zone (highest zone temperature)), which projected just below the molten source material inside a platinum or rhodium crucible (sometimes graphite element can be used). The rod carrying the seed is rotated (~200 rpm) by gear-train motor (30 s clockwise and 30 s counterclockwise).
The nucleation rate will depend upon stirring speed since stirring shortens the diffusion path close to the growing face [20]. Since supersaturation increases with cooling rate, sometimes a greater critical stirring speed is required. Surprisingly, it is observed that the greater the critical stirring rate, the larger the mass of the melt. As a matter of fact, the seed is the principal sink on which excess solute deposits, so apparently the larger the melt mass the longer the diffusion path.
Czochralski (CZ) growth, named after the inventor, is the most common and versatile method ever known to grow single crystal with the aid of a seed material. The simplicity of the process and relatively high degree of crystal purity helped establish the CZ process as the dominant crystal growing technology in the early years of crystal growing industry. The development of dislocation-free ingot growth and automatic diameter control in the late 1960s led to the rapid growth of growing high melting temperature materials mainly silicon.
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree