The human visual system is remarkably complex and sophisticated. To date there is no man-made system that can rival its dynamic luminance range – a ratio of about 105 for lowest to highest luminance in a single scene and a ratio of 1010 over all luminance adaptations. There is no machine vision system that can rival its pattern recognition capabilities. In this chapter, I will describe some of the past work on absolute thresholds of simple aperiodic signals as well as work using periodic signals (mostly windowed sine waves). This will be a short description of studies of human vision without noise – except for studies investigating photon capture fluctuations at the retina.
The chapter starts in the nineteenth century with very simple investigations designed to determine a few “laws” that describe human capabilities. A few important milestones are as follows. Blackwell (1946) started the era of modern work using rigorous psychophysical methods and aperiodic test patterns such as discs. One very important aspect of his work was to establish that human observers could perform in a consistent manner with similar results from different observers. This was very surprising to many psychologists. The next dramatic change was Schade’s (1956) measurements of contrast sensitivity using one-dimensional (1D) sine-wave test patterns. Perhaps the most important step of all was Campbell and Robson’s (1968) hypothesis of independent detection of spatial frequency components and subsequent psychophysics experiments to test their hypothesis. The term “multiple visual channels” came later.
3.1 Nineteenth-Century Work
The earliest investigations of spatial vision concentrated on acuity (the ability to distinguish details in compact signals), luminance increment discrimination, and spatial summation effects. One example of acuity measurement is use of the familiar Snellen letter chart, named after the Dutch ophthalmologist Hermann Snellen, who developed it in 1862. Ernst Weber (1795–1878) was one of the first to study the human response to a luminance increment stimulus in a quantitative fashion. He found that the relationship between stimulus magnitude and perceived intensity is logarithmic, with a discrimination just-noticeable difference (JND) given by a constant value, JND = ∆ L/L, where L is luminance. One application, for example, is that the brightness of stars is described on a logarithmic (magnitude) scale.
Gustav Fechner (1801–1887) later offered an elaborate theoretical interpretation of Weber’s findings, which he called Weber’s law, though his admirers made the law’s name a hyphenate, the Weber–Fechner law. Fechner related the sensation, Y, and the physical intensity, X, using Y = a log(X) + b.
The Italian astronomer Annibale Ricco (1844–1911) investigated spatial summation for compact signals. He found that, with a small area near the fovea, about 0.1 degrees2, there was complete summation described by the equation CA = k, where C is contrast threshold, A is signal area, and k is a constant. This is now known as Ricco’s law.
For larger signals, spatial summation for compact signals is often described by Piper’s law, CA=k
. The equation is often valid for aperiodic signals subtending an angle between 0.1 and 20°. It is named after the German physiologist Hans Edmund Piper (1877–1915) who formulated it. In the temporal domain, there is also Bloch’s law, R = It, where R is the response, I is intensity, and t is time; the law seems to hold for presentations under 100 ms.
3.2 Blackwell – The Modern Era Begins
Blackwell (1946) was one of the first to do psychophysics experiments to evaluate contrast thresholds over a wide range of conditions. This was part of a World War II program under contract to the US Office of Scientific Research and Development. Aside from the data provided, the major contribution of Blackwell’s work was the demonstration that such measurements could actually be done in a reliable and reproducible manner. Because of this situation, I will spend some time discussing it.
At the outset of Blackwell’s work, there was considerable skepticism about its feasibility. Therefore, he proceeded in a very careful manner. The experimental viewing conditions were meticulously designed and controlled. There were 19 observers who took part in a variety of preliminary experiments over 6 months to a year. So they were veterans of between 35,000 and 75,000 observations (decision trials) before the data collection reported in the publication.
Blackwell found in his preliminary experiments that the precision of the initial physical photometric measurements was less than the precision of responses to visual stimuli. Five (and occasionally eight) contrasts were used for each stimulus and were presented in random order using an eight-alternative forced choice (8AFC) procedure. The contrasts had been selected to give probability of detection between 10% and 95%. A small red indicator point was projected on the viewing screen (62 feet from the observers) and the stimulus was superimposed at one of eight locations on the circumference of a circle of 3° radius. After a 6-second viewing period, observers selected a location using an eight-position electrical indicator. Average cumulative normal detection probability versus contrast curves were constructed and thresholds were defined as 50% correct after allowance for guessing. One of the figures in the paper shows such a curve based on 450,000 observations! It should be carefully noted that Blackwell’s work was done before the development of signal detection theory so the proper analysis of forced-choice experiments lay in the future. He did mention that, upon interrogation, observers stated that they did not feel confident of having “seen” a stimulus unless the probability of detection was greater than 90%! This is consistent with my own experience in 2AFC experiments with signals in noise – where observers usually have little confidence when the probability of detection is less than 80%.
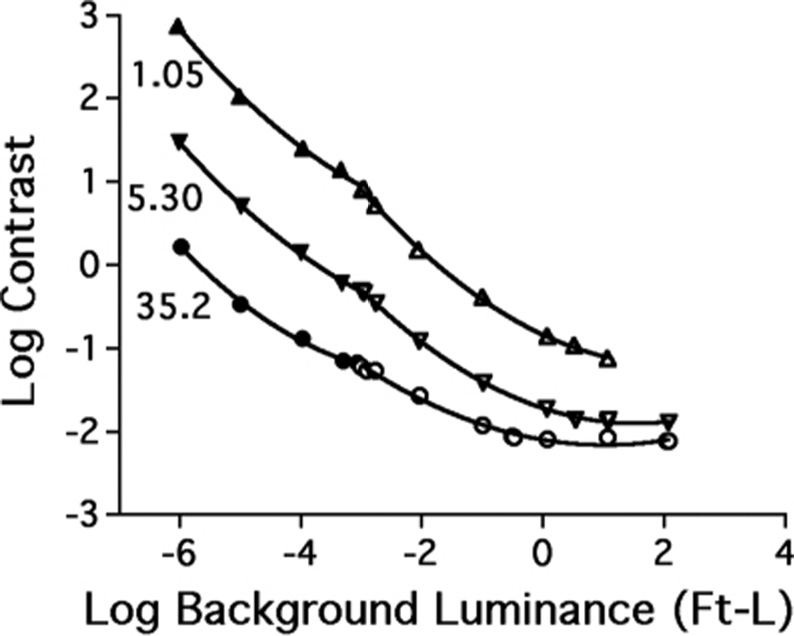
The stimuli were discs of various diameters and luminances, L, superimposed on uniform backgrounds of lower luminance, L0. Contrast was defined as C = (L − L0)/L0. Results for three of the five signal diameters (in milliradians) that were investigated are shown in Figure 3.1. The data were obtained from Table II of Blackwell’s (1946) paper. There is a cusp in the curves near a background luminance of 10−3 foot-lamberts (Ft-L) for all disc diameters. This corresponds to the point where cones are no longer activated and signals are detected using rods (scotopic vision).
Figure 3.1 Results of Blackwell’s experiments for detection of a disc signal over a range of background luminances. The associated numbers indicate the disc angular diameter in milliradians (1° = 17.5 mRad). The cusps in the curves occur near the luminance where cones begin to be activated. The data were obtained from Table II of Blackwell’s (1946) paper.
Blackwell also did contrast threshold experiments using signals darker than the background but they will not be discussed here. The Blackwell paper represented a historical moment in vision research. It demonstrated that psychophysical measurements could be done in a very reliable and reproducible manner.
3.3 Contrast Sensitivity Measurements
The next dramatic change in vision research (Schade, 1956) was the measurement of contrast sensitivity using 1D sine-wave test patterns. The patterns were 1D because of hardware limitations. The 2D displays that we now use did not become generally available until the 1970s and even then were very expensive. In 1981, my first 320 × 256 image display system cost about $30,000 – not including the minicomputer system (which cost another $70,000).
Otto Schade (1903–1981) published in 1956 the first measurement of the visual contrast sensitivity function (CSF) as a function of spatial frequency. Schade, a colleague of Albert Rose, worked at Radio Corporation of America (RCA) from 1931 until his retirement in 1968 and, among many other things, developed the concept of the modulation transfer function (MTF), which has had wide application in imaging. Schade made a number of contributions to vision research and was invited by Bob Wagner to speak at a Society of Photo-optical Instrumentation Engineers (SPIE) medical X-ray conference in 1974 on the topic of evaluation of noisy images. I had the pleasure of meeting him there. He was then retired but still had the enthusiasm of a graduate student for his subject – showing us an advance copy of his wonderful book on image quality (Schade, 1975). Wagner subsequently wrote a number of papers translating Schade’s work (based on a sampling aperture concept) into the more modern notation with which we are familiar.
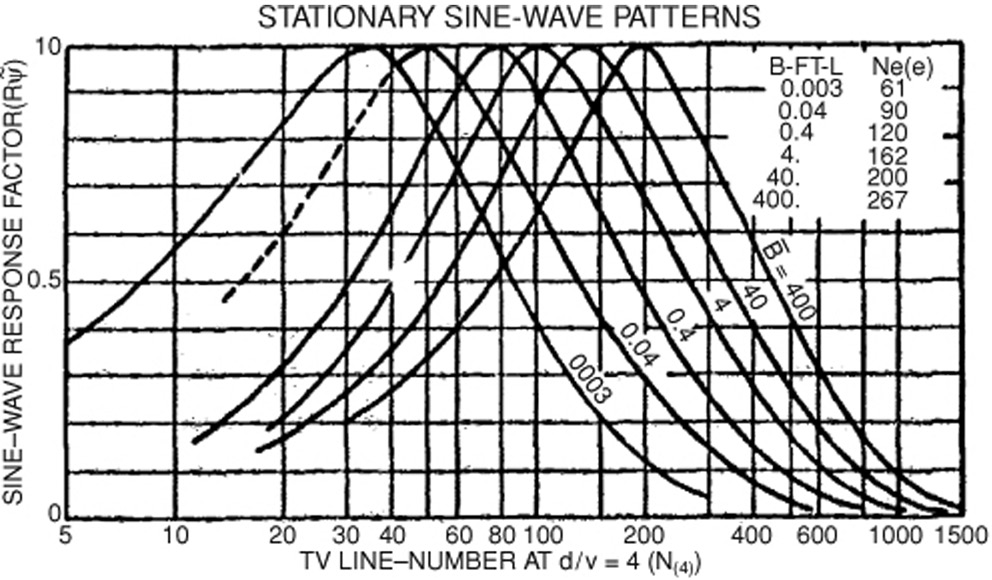
Schade’s CSF measurements for detection of static 1D sine-wave patterns on a special TV monitor (60 Hz frame rate and 500–800 vertical lines) are shown in Figure 3.2. He gave measurements for a wide range of luminances with the monitor mounted behind an opening in a large white adapting screen whose luminance was adjusted to match the average monitor luminance. He used a method of adjustment. The sine-wave amplitude was increased steadily from zero at a fixed rate until the observer subjectively “saw” the test pattern. This ascending threshold value was recorded. The procedure was repeated until the observer achieved an approximately steady threshold criterion and these “learning” results were discarded. Then 10–15 more trials were done and the results averaged for the threshold curve determination. Each curve in the figure is based on 200–600 trials.
Figure 3.2 Contrast sensitivity function measured by Schade (1956) using a one-dimensional sine-wave pattern at six average luminance values. The spatial frequency conversion factor is 1 cycle/degree = 28.6 TV line numbers. The peak for B of 400 Ft-L occurs at 7 cycles/degree.
Schade interpreted his CSF results as a combination of the low-pass characteristic of the MTF of the eye optical system followed by the effects of neural interaction at the retina. The low-frequency falloff is due to the physiology of the retina and the way the brain interprets visual information. From early times in vision research there had been interest in the phenomenon of Mach bands. This is an illusion that appears when there is a narrow gradient between a large dark gray region in an image and a large light gray region. We perceive a narrow darker band in the dark gray region adjacent to the gradient and a narrow lighter band in the light gray region. This can be interpreted as an indication of a decrease in the CSF at low frequencies. This view is consistent with the physiological discovery that the retinal ganglion cells of the frog (Barlow, 1953) and cat (Kuffler, 1953) respond to contrast rather than absolute brightness.
Note that Kuffler had earlier suggested this possibility and was Barlow’s PhD thesis supervisor. They found receptive fields with antagonistic regions – an “ON” (excitatory) center and an “OFF” (inhibitory) surround. If this receptive field is interpreted as a point spread function, then the corresponding Fourier transform has a band-pass form. Note there is a marked dependence on mean luminance in Schade’s CSF results due to retinal adaptation.
3.4 Channels – Independent Detection of Some Fourier Components
DePalma and Lowry (1962) investigated the frequency dependence of both sine-wave and square-wave detection. They claimed that, at high spatial frequencies, sine-wave gratings were seen at lower contrast than square waves of the same frequency – with the implication that there was something very special about the smoothly changing luminance. This indirectly led to the spatial frequency channels concept discussed below.
The following is John Robson’s version of the story (personal communication). He had been a graduate student of Fergus Campbell at Cambridge University. Fergus asked John for his opinion about the Lowry and DePalma results. John said that, unless the laws of physics had recently changed, their results could not be correct and he didn’t think there was any point in trying to repeat their observations, which must simply be wrong. This was due to the fact that the first harmonic of the square wave would be at three times the frequency of the fundamental and at high enough frequencies it would be outside the optical pass band of the lens. The fundamental of the square wave would have a frequency the same as the sine wave, but as is shown in Equation (3.1), the fundamental would have an amplitude that was higher by a factor of 4/π. So one would expect that the square wave would be detected at lower contrast. This is the opposite of the Lowry and DePalma results.
They did a preliminary experiment and found that the relative visibility of sine and square-wave gratings of high spatial frequency was, as well as they could measure it, exactly what would be expected if the optics filtered out the higher harmonic components of the square wave when they fell above the optical resolution limit. They then did investigations of the relative visibilities at frequencies below the CSF peak. This was based on the view that the harmonic components were not removed (or might even be relatively enhanced within the neural structures), so the square waves might become relatively more visible.
John said that, making some assumption about the neural MTF (i.e., interpreting the CSF as an overall MTF of the optics + nervous system), it ought to be possible to predict the deviation of the threshold contrast ratio from its asymptotic high-frequency value. So he tried to make the predictions assuming various detection criteria for the effective internal image (e.g., peak-to-peak, maximum luminance slope) but couldn’t get any predictions to fit their data. The contrast threshold ratio only deviated from the high-frequency value when the spatial frequency was quite a lot lower than would be predicted by any of those detection criteria. It looked as though the harmonic components were of no consequence until they could be independently detected.
They then did some experiments with two-component compound gratings, which gave results consistent with this somewhat surprising notion that “components of the retinal image” might be detected independently. This ultimately led to the “multiple channels” hypothesis, which makes sense since the human visual system obviously needed many channels in order to see – a single-channel device would simply represent a spatial frequency filter and a photometer.
Campbell and Robson (1968) described a number of psychophysics experiments to test their hypothesis of independent detection of spatial frequency components. The term “multiple channels” came later – but they understood that was what their work was all about. After developing a high-quality apparatus, their first experiment involved repeating the comparison of thresholds using sine-wave and square-wave test signals (gratings, as they are called in spatial vision research). A square wave, FSQ(x), of period X, can be described by its Fourier series components according to:
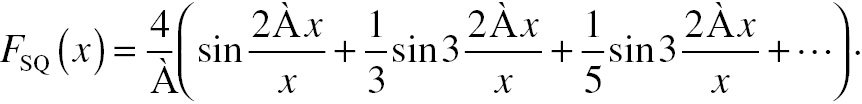(3.1)Related posts:
Stay updated, free articles. Join our Telegram channel
Full access? Get Clinical Tree
