CHAPTER 81 Magnetic Resonance Angiography
Physics and Instrumentation
There have been dramatic improvements in MR hardware and acquisition approaches over the past decades, many of which have proved beneficial for or were inspired by angiography applications; these now allow for more rapid imaging with improved image contrast and reproducible image quality. This chapter discusses some underlying physical principles of vascular imaging using MRI and the instrumentation and acquisition approaches for typical MRA examinations. In addition, the various mechanisms for vascular bright blood vascular depiction using CE-MRA, time-of-flight MRA (TOF MRA), phase contrast MRA, and steady-state free precession MRA will be discussed. It should be noted that the terminology for MR techniques can sometimes be confusing, not only because there are many variants of related schemes, but also because various vendors usually market these technologies under different trade names.1
DATA ACQUISITION AND IMAGE RECONSTRUCTION
MRI is based on the nuclear magnetic resonance phenomenon, which arises in atoms with an odd number of protons and/or an odd number of neutrons. Almost all clinical MRI, including angiography applications, is based on the signal from the hydrogen nucleus, 1H, a single, positively charged proton that is the most abundant in the body (mainly in H2O but also in fat and other compounds). From a classic physics perspective, each proton has a magnetic dipole moment and behaves like a tiny bar magnet when interacting with a magnetic field. In the absence of an external magnetic field, the axes of the magnetic dipole moments are randomly arranged so that they in aggregate cancel each other out and result in a net magnetization of zero. However, in the presence of an external magnetic field, or B0, the magnetic dipole moments of the protons will align parallel or antiparallel with the external field. In thermal equilibrium, slightly more spins are aligned parallel than antiparallel with the external magnetic field because it requires less energy. The result is a net magnetization that becomes the signal source in MR experiments. As shown in Figure 81-1A, this vector quantity is aligned with the direction of the main magnetic field. To measure signal from the net magnetization, it has to be “tipped” away from its orientation along the main magnetic field into the transverse plane. Once it is tipped away from the longitudinal axis, the magnetization vector rotates, or precesses, around that axis with a characteristic frequency, as given by the Larmor equation:
where ω0 is referred to as the Larmor frequency, γ is the gyromagnetic ratio of protons (42.58 MHz/T) and B0 is the magnetic field flux of the main field. The precessing transversal magnetization Mxy creates a weak radiofrequency (RF) field that can be recorded with a properly tuned coil (e.g., 63.87 MHz at B0 = 1.5 T) circularly polarized about the axis of precession. As illustrated in Figure 81-1B, the tipping of the net magnetization vector is accomplished with a second RF system that can generate an oscillating magnetic field, B1, close to the resonance frequency, a concept called RF excitation. In practice, the RF pulse is played out very shortly (in the order of milliseconds) and a remarkably small B1 field is sufficient for RF excitation (e.g., ~50 mT in comparison to B0 = 1.5 T).
Multiple RF excitations are needed to acquire enough data for a two-dimensional image. The time between successive excitations is controlled by the MRI parameter repetition time (TR). The time between an RF excitation and the recording of the signal is controlled by the MRI parameter echo time (TE). Both of these parameters have an impact on the measured MR signal because they dictate how much time is allowed for the decay of the transversal magnetization (TE) or the regrowth of the longitudinal magnetization (TR). The actual measured MR signal depends on the T1 and T2 relaxation times, proton density, choice of TR and TE, and other imaging parameters, but also on factors such as motion, diffusion, and temperature. In MRA, the goal is to generate high signal differences between blood and surrounding background tissues, which is achieved by incorporating the motion of the blood using noncontrast MRA techniques or T1 shortening of blood by a circulating Gd chelate contrast agent using CE-MRA. Typical relaxation times are T1/T2 = 1200/250 ms for arterial blood and T1/T2 = 1200/220 ms for venous blood at 1.5 T.2 Whereas T1 increases with field strength for most tissues (1600 ms for arterial blood), the T2 undergoes a small decrease.
Image Encoding
To create an image, the spatial distribution of the protons has to be resolved. Spatial encoding is achieved with the use of spatially and temporally varying magnetic fields. In the first step, the signal-generating volume is reduced to a slice (two-dimensional imaging) or volume (three-dimensional imaging) by selective excitation. A linear magnetic field gradient is superimposed onto the main magnetic field in the slice (or volume) direction simultaneously with the RF excitation. Consequently, the resonance frequency of the spin system is linearly varying as well. The RF system generates a pulse that excites not only a single precession frequency but all the frequencies contained in the desired slice or volume. Spins located outside the desired volume are not excited because their precession frequency is higher or lower than the frequency band covered by the RF excitation. Theoretically, this concept could be repeated in orthogonal directions ultimately to excite only a single point in the object, and then repeat the measurement until all points in the image are sampled. However, a much more efficient approach is Fourier encoding, whereby each sampled signal contains contributions from all spins in the object, therefore dramatically increasing the SNR of the acquisition. Figure 81-2 shows a basic example for a Fourier-encoded two-dimensional acquisition in the form of a pulse sequence diagram that illustrates the timing for RF excitation, the gradients, and data acquisition.
The third gradient, called the phase-encoding gradient, is switched on and off prior to the frequency-encoding gradient. This leads to a linearly varying phase of the net magnetization vectors along the direction of that gradient, providing encoding for a single spatial frequency. In other words, with properly controlled gradients, the time-dependent MR signal actually samples the spatial frequencies of the object in two dimensions. Figure 81-2 shows how the sampling trajectory in the spatial frequency space, also called k-space, is controlled by the area under the gradient waveforms.
If that experiment is repeated several times with varying phase-encoding gradients, then multiple spatial frequencies are sampled in the phase-encoding direction as well and an image can be reconstructed via inverse two-dimensional Fourier transform. Figure 81-3 shows an example of a sagittal head scan. The received signal is digitized by an analog to digital converter and a discrete inverse two-dimensional Fourier transform generates the corresponding image, which contains magnitude (length of the transversal magnetization) and phase information (position in the transverse plain in respect to the coil axis) for each voxel. Most MRI relies on the magnitude image only. However, in some applications, such as phase contrast MRA, the phase information plays an essential role in generating image contrast.
Figure 81-4 provides some insight about the signal distribution in k-space. For this image, most of the signal energy is located around the origin of k-space. This area defines the weighting for the low spatial frequencies, which define the coarse outline of the imaged object yet lacks information on edges. The broad pattern of the phantom can be identified even with less than 0.5% of the k-space samples from the full-resolution image. However, all the detail is lost, which is defined in the unsampled higher spatial frequencies. The difference in images between the low-resolution images and the fully sampled image reveals the errors caused by limiting the acquisition to lower spatial frequencies only.
The basic signal-to-noise ratio (SNR) in MR acquisitions is given by
where Tacq represents the data acquisition time.3 Based on this relationship, one notes that the desirable properties for MRA, such as small voxel size for higher spatial resolution imaging and short acquisition times for fast scans, are detrimental to the obtainable SNR. Frequently, compromises in scan parameter choices are necessary for optimization of MRA for imaging individual vascular territories, with each offering unique challenges for proper visualization by MRA.
TECHNICAL REQUIREMENTS
Magnetic Resonance Scanner
Each MR scanner is designed to provide a magnetic field, B0, that is homogeneous over the imaging volume, stable over time, and against the influence of external sources. The magnetic induction of the field, also frequently referred to as the magnetic field strength, is measured in tesla (T) or gauss (G), where 1 T = 104 G. The most common modern MR scanners used in clinical practice are whole-body scanners that rely on a superconducting magnet with a field strength of 1.5 or 3 T. For comparison, the field strength of a 1.5-T MR scanner is about 30,000 times stronger than the earth’s magnetic field (~0.5 G). Superconducting MR scanners contain several coils that run in series to form a solenoid and create a cylindrically symmetrical magnetic field, with the main magnetic field aligned with the z-axis of the cylindrical housing (Fig. 81-5). They operate near absolute zero temperature (~4 K, or −270° C) to effectively eliminate electrical resistance in the coil wires, allowing large currents that generate high magnetic fields. Other MR scanner designs include resistive magnetic fields (without superconductivity) that operate at lower magnetic field strengths (<0.15 T), permanent magnetic fields that have intermediate field strength (<0.7 T), smaller bore diameter MR scanners for extremity imaging, and open bore designs (usually <0.7 T) to improve patient comfort and reduce claustrophobia. Imaging at lower field strengths reduces some of the problems encountered at higher fields, such as reduced RF penetration and increased energy absorption. However, MRA applications greatly benefit from imaging at higher field strength because the SNR increases linearly with field strength. MR scanners with field strengths of 3 T are becoming more prevalent in clinical settings and favorable results have been reported with these systems4; more recently, higher field 7-T research MR systems are being investigated.5
Gradient System
The magnetic field gradient system typically consists of three orthogonal gradient coils embedded within the housing of the scanner. Gradient coils are designed to produce a spatially varying magnetic field that can be temporally altered. Although these additional magnetic fields are dramatically smaller than the main magnetic field, they are an essential component for spatial encoding of the signal distribution in the imaging volume of interest. Gradients are rated by their maximum gradient strength (the higher the better) and the time needed to rise to the maximum gradient strength (slew rate—the faster the better). The gradient waveforms in Figure 81-2 are idealized in that they can be switched on and off instantaneously.
Radiofrequency System
The RF system serves the purpose of converting pulsed oscillating currents from a power amplifier into an oscillating magnetic field, also referred to as the B1 field, for the excitation of the nuclear spin system. This is accomplished by a transmitter coil tuned to the resonance frequency of the spin system under investigation. Such coils are designed to provide a uniform B1 field over the imaging volume to ensure uniform spin excitation. So-called birdcage coils are well suited for this purpose and exist as whole-body coils built into the scanner housing and as specialty coils, such as for head or knee imaging (Fig. 81-6).
A receiver coil is used to detect the RF field created by the precessing magnetization of the nuclear spins after their excitation. The same coil may be used for transmitting and receiving the RF signals, which are referred to as transmit-receive coils (see Fig. 81-6A and B). However, in many applications, it is advantageous to use special local coils that are sensitive only to the signal from the region close to the coil. Such local coils can have a significantly better SNR, as described by equation 2, especially compared with the whole-body transmit-receive coil that encounters noise contributions from the whole volume within it that is much larger than that of smaller local coils. Figure 81-6 shows examples of such local coils, including a shoulder coil (C) and a multipurpose flex coil (D).
Coil arrays consist of multiple local receiver coils to provide a larger coverage with the SNR advantage of local coils. These multielement RF coils have become very common and are available for many applications in many body regions (Fig. 81-6E-H). Figure 81-7 demonstrates the local coil sensitivities of an eight-channel head coil in a three-dimensional TOF MRA and the cumulative image obtained from combining all channels. Data from multiple coils cannot be combined during acquisition and must be stored individually. Therefore, the acquired data size increases linearly with the number of coils, and image reconstruction must be completed for each channel before they can be combined. However, current reconstruction engines are prepared to handle the additional workload for 8 to 16 channels without significant delays.
Parallel Imaging
More recently, scan time reduction methods were developed that explore the redundancy in the information contained in multiple receiver coils with partly overlapping sensitivity regions. SMASH (simultaneous acquisition of spatial harmonics),6 SENSE (sensitivity encoding),7 and GRAPPA8 are acquisition and reconstruction methods from this group referred to as parallel imaging, which have found rapid commercial adaptation for cardiovascular imaging, especially CE-MRA. With this approach, the equivalent of multiple-phase encoding steps is acquired during a single readout. Although the advantage of accelerated imaging comes at the expense of a reduction in SNR, it can be highly beneficial in situations in which there is sufficient SNR and rapid image acquisition is desired. Basically, all CE-MRA applications fall into this category because faster imaging can improve the frame rate for dynamic acquisitions or reduce breath-hold lengths or other artifacts related to patient motion. As shown in Figure 81-8, attention has to be paid to potential local noise amplifications from the reconstruction process, frequently described as g-factor maps, when using high acceleration factors.
The scan time reduction factor that can be obtained with parallel imaging depends on the number of independent coil elements and their arrangement. Therefore, further increases in the number of coil elements and receiver channels promise even greater reductions in scan time. Consequently, the recent progress in parallel imaging has led to renewed interest in coil design and receiver systems. New MRI platforms provide the ability to connect many coil elements and acquire the signal from as many as 16 to 32 receivers simultaneously and systems with even more channels are being explored. With higher receiver coil counts, the SNR and coverage can be further improved or higher acceleration factors can possibly be obtained. Current design problems, such as with the decoupling of individual coil elements, has limited commercial coil designs to 8 to 32 elements. In research settings, systems with up to 128 receiver channels have been recently evaluated and demonstrated regional SNR benefits and high acceleration factors for cardiovascular applications as compared with lower count coil arrays.9 However, the lack of penetration for small coils might limit additional benefits for regions of interest that are more distant from the body surface. It remains to be seen at which point additional coil elements are still beneficial, especially when the noise in the MR system becomes dominated by electrical noise from the receiver system, instead of intrinsic noise contributions caused by random motion of electrons in the patient’s tissue.
Power Injector
For CE-MRA, a Gd-based contrast agent is injected intravenously, usually into an antecubital vein. The injection of the contrast agent is followed by the injection of sterile saline to flush the contrast material out of the delivery system and ensure that the contrast bolus is sufficiently central within the body. Although manual injections are possible, the use of a computer-controlled, MR-compatible power injector is usually preferred. The power injector is more precise in delivering reproducible injection rates and its use omits the need for an operator in the scan room during imaging. Power injectors also provide features to assist in dynamic timed studies and breath-hold maneuvers and allow for multiphase injections. A typical system consists of a delivery component in the scan room and a user console in the operator room (Fig. 81-9). Manual injections have the advantage that the physician is right next to the patient and can better monitor the administration of contrast agent and the status of the patient.

 FIGURE 81-1
FIGURE 81-1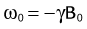
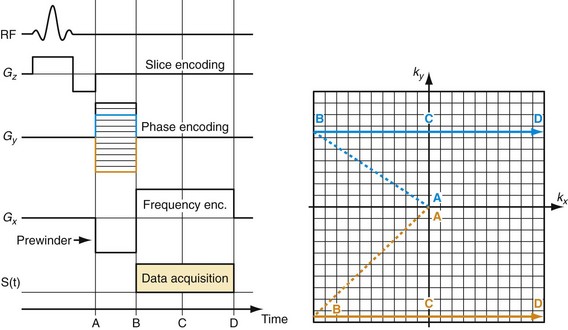
 FIGURE 81-2
FIGURE 81-2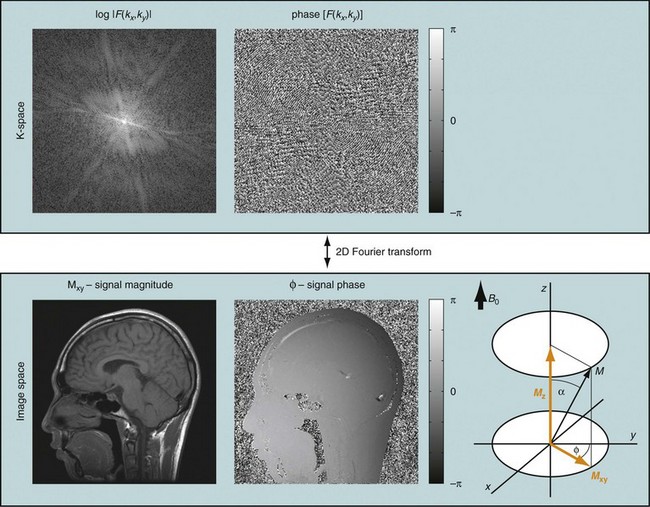
 FIGURE 81-3
FIGURE 81-3
 FIGURE 81-4
FIGURE 81-4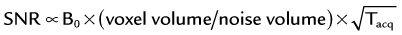
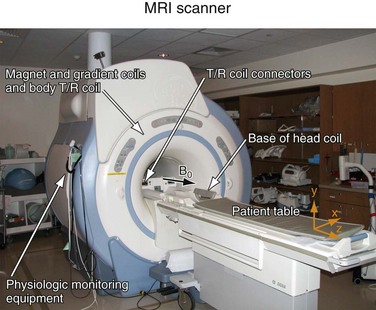
 FIGURE 81-5
FIGURE 81-5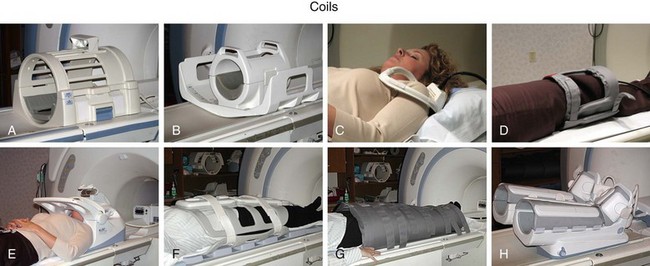
 FIGURE 81-6
FIGURE 81-6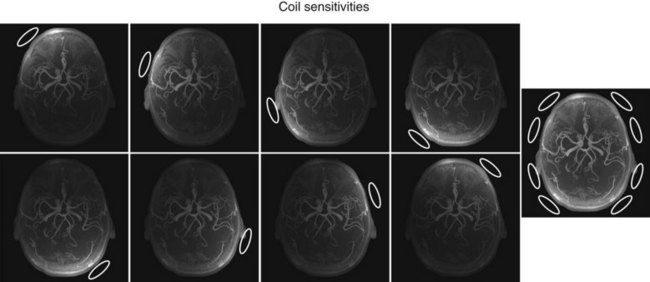
 FIGURE 81-7
FIGURE 81-7
 FIGURE 81-8
FIGURE 81-8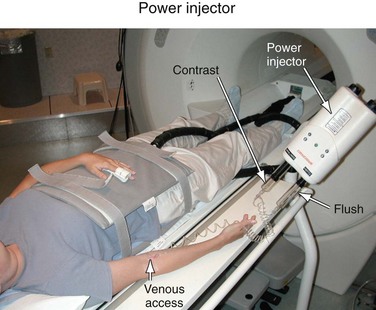
 FIGURE 81-9
FIGURE 81-9


