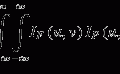Fig. 13.1
Characteristic of dose distributions in the depth direction in proton therapy and common X-ray therapy

Fig. 13.2
Proton therapy system in National Cancer Center Hospital East Japan
Proton therapy can provide high dose concentration on a target tumor. Without precise irradiation following a treatment plan, a lower rate of treatment control and a higher risk of serious damage than conventional radiotherapy may be caused by proton irradiation to a target region including organs at risk (OAR), as shown in Fig. 13.3.
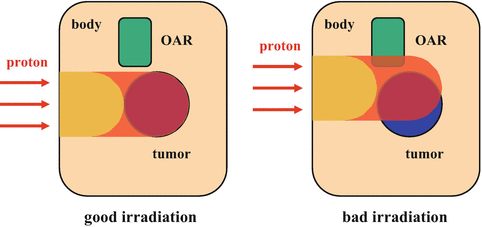

Fig. 13.3
Conceptual diagram expressing differences in dose concentrations depending on accuracy of proton beam irradiation
Although various advances have been made in irradiation technologies to deliver prescription dose to a tumor more accurately for proton and other radiation therapies, it has been impossible to know whether proton irradiation is performed to a target tumor following a treatment plan during a patient being irradiated. It is essential to confirm whether prescription dose is delivered to a tumor in order to provide radiation therapy with high dose concentration for patients precisely and safely.
Research on imaging of an actual irradiated region in proton therapy has been conducted, which utilizes positron-emitting nuclei generated by target nuclear fragment reactions between incident protons and nuclei in a human body in the irradiated region.
13.2 Principle of Imaging a Region Irradiated by Proton Beam Using Target Nuclear Fragment Reaction
Proton beam with around 220-MeV kinetic energy is used in proton therapy. There are four nuclear reactions around this energy: elastic and inelastic scattering reactions, Coulomb dissociation reaction, and target nuclear fragment reaction. A reactant nucleus is fragmented into several pieces in Coulomb dissociation reaction and nuclear fragment reaction. Coulomb dissociation reaction occurs when a huge Coulomb force is generated from a reactant nucleus with a large atomic number and another nucleus is at close range. A patient’s body is mainly composed of hydrogen nuclei, carbon nuclei, nitrogen nuclei, oxygen nuclei, and calcium nuclei (see Table 13.1) (ICRU Report 46 1992). Therefore, there is a slight possibility of Coulomb dissociation reaction in proton therapy.
Table 13.1
Examples of composition of human organs
Body tissue | Element composition [%] | Mass density [kg/m3] | |||||
|---|---|---|---|---|---|---|---|
H | C | N | O | Ca | Others | ||
Liver (adult) | 10.2 | 13.9 | 3.0 | 71.6 | 0.0 | 1.3 | 1060 |
Adipose tissue (adult) | 11.2 | 51.7 | 1.3 | 35.5 | 0.0 | 0.3 | 970 |
Soft tissue (adult) | 10.5 | 25.6 | 2.7 | 60.2 | 0.0 | 1.0 | 1030 |
Lung (adult) | 10.3 | 10.5 | 3.1 | 74.9 | 0.0 | 1.2 | 260 |
Muscle (adult) | 10.2 | 14.3 | 3.4 | 71.0 | 0.0 | 1.1 | 1050 |
Skeleton sacrum (male) | 7.4 | 30.2 | 3.7 | 43.8 | 9.8 | 5.1 | 1290 |
Skeleton cranium (adult) | 5.0 | 21.2 | 4.0 | 43.5 | 17.6 | 8.7 | 1610 |
Skeleton femur (adult) | 7.0 | 34.5 | 2.8 | 36.8 | 12.9 | 6.0 | 1330 |
Skeleton ribs (adult) | 6.4 | 26.3 | 3.9 | 43.6 | 13.1 | 6.7 | 1410 |
Target nuclear fragment reaction is defined as nuclear fragmentation in a limited region where a nucleus collides with another one (Goldhaber 1974). The reaction occurs by incident protons and target nuclei in a patient’s body, which is like incident protons ripping nucleons from target nuclei. Many different nuclei which are lighter than target nuclei are generated by the reaction, and its yield is strongly related to energy of incident protons. The generated nuclei include positron-emitting nuclei which are proton-rich nuclei. Positron-emitting nuclei generated by proton irradiation release positrons, and pairs of annihilation gamma rays with 511 keV are emitted when a positron and an electron collide. We can derive the position where a positron-emitting nucleus was generated and its intensity from simultaneous detecting pairs of gamma rays. Figure 13.4 shows a conceptual diagram illustrating target nuclear fragment reaction between an incident proton and a target nucleus and a method for deriving the position of the generated positron-emitting nucleus. Positron emission tomography (PET) is a type of nuclear medicine imaging using this principle and method. Five elements, hydrogen nuclei, carbon nuclei, nitrogen nuclei, oxygen nuclei, and calcium nuclei, are regarded as the main human body’s composition, and target nuclear fragment reactions occurred by four of five elements except hydrogen nuclei in an energy range used in proton therapy. Table 13.2 shows target nuclear fragment reactions X(p,A)Y, which we should take into consideration, for imaging of a region irradiated by proton beam and positron-emitting nuclei generated by the reactions. Figure 13.5 shows the partial reaction cross sections of generating positron-emitting nuclei by nuclear fragment reactions about carbon nuclei and oxygen nuclei (NNDC 2016). Data on reaction cross sections of target nuclear fragment reactions for proton beam energy is shown in Fig. 13.5. It is insufficient in an energy range of proton therapy as far as we know from the newest data on the reaction cross sections reported in nuclear physics field which was released by National Nuclear Data Center (NNDC). A recoil shift of nuclei generated by target nuclear fragment reactions can be negligible according to the law of conservation and momentum (law of conservation of four-momentum) in the special theory of relativity, amounts of kinetic energy of incident protons. Imaging an actual proton-irradiated region from information of detected positions and intensity of positron-emitting nuclei generated in a patient’s body enables us to verify whether accurate proton irradiation is performed to a target tumor.
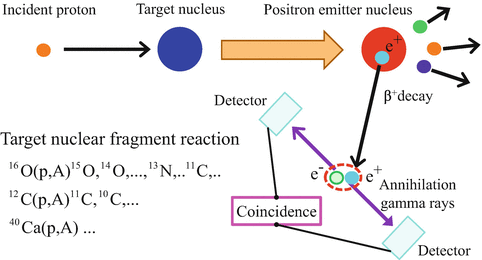
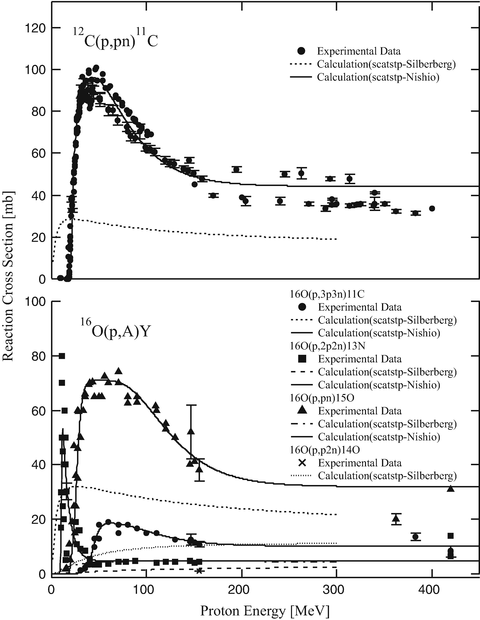

Fig. 13.4
Conceptual diagram expressing target nuclear fragment reaction between an incident proton and a target nucleus and a method for deriving the position of the generated positron-emitting nucleus
Table 13.2
Target nuclear fragment reactions X(p,A)Y which we should take into consideration for imaging of a region irradiated by proton beam and positron-emitting nuclei generated by the reactions
Reaction channel | Half-life [min] | |
|---|---|---|
X | Y | |
12C | 11C | 20.39 |
1 °C | 0.321 | |
16O | 15O | 2.037 |
14O | 1.177 | |
13N | 9.965 | |
11C | 20.39 | |
1 °C | 0.321 | |
14N | 13N | 9.965 |
11C | 20.39 | |
1 °C | 0.321 | |
40Ca | 38K | 7.636 |
30P | 2.498 | |
15O | 2.037 | |
13N | 9.965 | |
11C | 20.39 | |

Fig. 13.5
Experimental and calculated partial reaction cross sections of generating positron-emitting nuclei by target nuclear fragment reactions about carbon nuclei and oxygen nuclei (Nishio et al. 2005)
Recently, a number of researchers have been studying imaging of a region irradiated by proton beam using target nuclear fragment reactions using PET in order to provide highly accurate proton therapy which takes advantage of high dose concentration. The papers by Bennett et al. indicated the possibility of imaging the region irradiated by high-energy proton beam to matter (Bennett et al. 1975, 1978). Other studies including measurement and simulation of artificial phantoms and real animals for clinical use with images of irradiated regions (Litzenberg et al. 1992, 1999; Miyatake et al. 2011; Miyatake and Nishio 2013; Nishio et al. 2005, 2006; Oelfke et al. 1996; Paans and Schippers 1993; Parodi and Enghardt 2000; Parodi et al. 2002; Parodi et al. 2005; Parodi et al. 2007a, b, Parodi et al. 2007c; Vynckier et al. 1993) and approaches to measured PET images (Hishikawa et al. 2002; Miyatake et al. 2010; Nishio et al. 2001, 2008, 2010) have also reported. Especially, each research team has worked on more actively since commercial PET systems began to spread widely in 2000.
13.3 Beam OFF-LINE and ON-LINE PET Systems
Target nuclear fragment reactions between incident protons and nuclei generate many different positron-emitting nuclei in a region irradiated by proton beam in a patient’s body. A positron-emitting nucleus generated in a patient’s body decays (β + decay) and emits a positron according to the half-life of the nucleus. Thus,
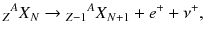
(13.1)
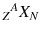 is the parent isotope,
is the parent isotope, 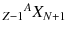 is the daughter isotope, e + is a positron, and ν + is a neutrino. Energy of β + decay is expressed by
is the daughter isotope, e + is a positron, and ν + is a neutrino. Energy of β + decay is expressed by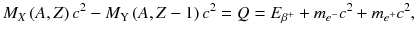
(13.2)
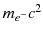 is the rest mass energy of an electron,
is the rest mass energy of an electron, 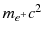 is the rest mass energy of a positron, and
is the rest mass energy of a positron, and 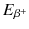 is the kinetic energy of a positron. Besides, to lead to β + decay, the Q value is given by
is the kinetic energy of a positron. Besides, to lead to β + decay, the Q value is given by![$$ Q\ge {m}_{e^{-}}{c}^2+{m}_{e^{+}}{c}^2=0.511+0.511=1.022\ \left[\mathrm{MeV}\right]. $$](https://radiologykey.com/wp-content/uploads/2017/07/A340376_1_En_13_Chapter_Equ3.gif)
(13.3)
Positrons emitted by β + decay lose their energy continuously through interactions with orbital electrons of atoms in a human body. When a positron and an orbital electron collide near the end of the positron track to annihilate, they can produce two gamma rays whose energy is equal to the total of their rest mass energy (1.022 MeV). The energy of the two particles is equivalently divided into the two gamma rays according to the law of conservation of energy and momentum. Each of the gamma rays with 511 keV is released in opposite direction. To be precise, we cannot say that each annihilation gamma ray has an exact energy of 511 keV or that the two annihilation gamma rays travel at exact 180 degrees to each other because the rays obtain energy which the positron has as kinetic energy when annihilation occurs.
A procedure for imaging a region is irradiated by proton beam: (1) target matter or a patient receives proton irradiation in a treatment room, (2) they move to a room where a commercial PET system or PET-CT system has been installed, and (3) PET images of each target are taken. In this procedure, proton treatment system and PET system or PET-CT system have been installed in separate rooms. The proton treatment system following this procedure is called beam OFF-LINE PET system. The most critical issue in this system is that PET images cannot be taken right on the spot after the proton irradiation. The most essential positron-emitting nuclei with large yields for imaging the region irradiated by proton beam are 15O nuclei (half-life, 2 min) generated by a reaction of 16O(p,pn)15O in a human body. We cannot get enough information of 15O nuclei for the imaging because it takes several minutes to start taking PET images after the irradiation in this system. The patient needs to move to the PET room after the proton irradiation, and thus, the patient’s conditions in proton irradiation and a PET scan are different. Therefore, the precision of imaging the region deteriorates. The patient has to spend extra time which is a burden to the patient, until finishing the PET scan after proton irradiation. However, this system has the advantages of an easy operation, enough preparation of image-reconstruction algorithms, and functions such as a fusion function between PET images and other medical images (e.g., CT images) or a function of analyzing PET or PET-CT images because commercial PET or PET-CT systems are used.
A system with a PET scanner installed on the beam line in a proton irradiation room is called beam ON-LINE PET system. Installing new PET developed with higher spatial resolution and positional accuracy on the beam line enables us to confirm which region proton beam is delivered in real time during a target tumor in a patient’s body being irradiated. Precision of proton irradiation can be observed and kept ensured highly during a treatment period which has the possibility of changing patient’s condition such as a reduction in tumor size. Finding out about some changes immediately and coping with them lead to providing patients with high-precision proton therapy. This system also enables us to verify the precision of calculating dose distribution in a patient’s body using a proton treatment planning system, which leads to proposing a more optimal treatment plan and judging the most optimal condition of proton beam for the patient.
Beam ON-LINE PET system has some difficulties to obtain a large detection field and to reconstruct three-dimensional images due to a geometrical arrangement of proton beam and PET apparatus. It is necessary to construct a system with a device which can take images in a proton irradiation room such as CBCT (cone-beam CT) in order to improve the accuracy of fusion of PET and CT images. However, there is a great advantage of beam ON-LINE system; PET images are immediately taken, keeping the patient condition after proton irradiation. Therefore, it is possible to detect activity of positron-emitting nuclei with faster half-lives including 15O nuclei which is the most essential nuclei for imaging the irradiated region. Furthermore, patients do not need to have extra time for moving to another room to take PET images. This advantage overwhelms above difficulties, and beam ON-LINE PET system would be very useful. Figure 13.6 shows an explanatory diagram of beam OFF-LINE PET system and beam ON-LINE system.
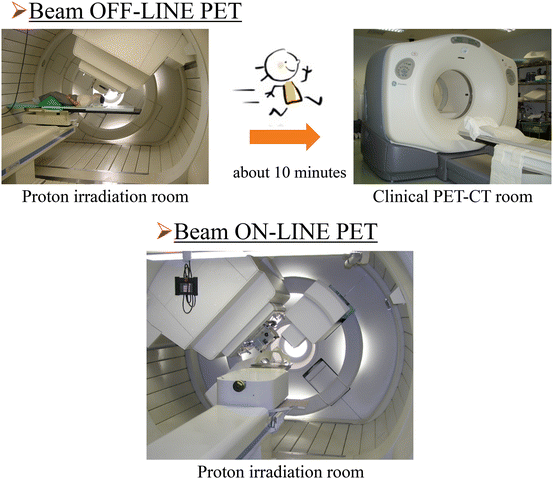

Fig. 13.6
Explanatory diagram of beam OFF-LINE PET system and beam ON-LINE system
13.4 Studies on Imaging of the Irradiated Region Using Beam OFF-LINE PET System
Imaging of a region irradiated by proton beam using beam OFF-LINE PET system is defined as a procedure: target matter or patients are irradiated by proton beam and move to a room where a commercial PET system or PET-CT system has been installed; after that PET scans of target matter or patients are performed.
Let us show some experiments using a commercial PET system. Targets of polyethylene (CH2) and water were irradiated by proton beam, and their activities were measured using the PET system. Mono-energy proton beams with about 30 mm FWHM at 138, 179, and 223 MeV were used in this experiment. The targets were irradiated for 10 s with around 10 GyE measured at a point of targets’ surface where proton beam arrives. It took about 2 min to start measurement of activity in the targets in the PET scan room after the irradiation. PET scan took 2 min for each irradiation. The width of PET scanning was 144.5 mm in the depth direction per one scan, and an overlap between each scan was 8.5-mm width. The two-dimensional OSEM (ordered-subsets expectation maximization) method (Boellaard et al. 2001) was used to reconstruct PET images from the measured activity data. The spatial resolution of this PET is 4.2–5.8 mm. Figure 13.7 shows the image of measured activity distribution of the positron-emitting nuclei generated in CH2 target using the PET when the target was irradiated by mono-energy proton beam at 179 MeV.
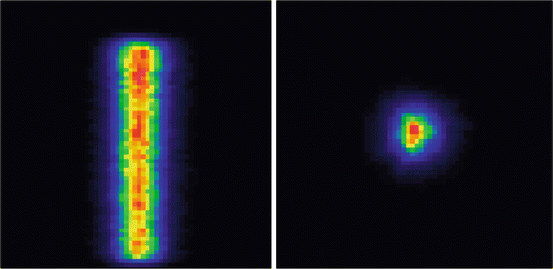

Fig. 13.7
Images of the measured two-dimensional activity distribution in CH2 target in the depth direction (left) and the lateral direction (right) (Nishio et al. 2005)
Target nuclear fragment reactions do not occur between protons from proton beam and other protons from CH2 target. The positron-emitting nuclei generated in CH2 target can be narrowed down to only 11C nuclei generated by 12C(p,A)11C, considering extra time from the end of proton irradiation to the beginning of measurement using PET and results of calculations for yields of various positron-emitting nuclei by target nuclear fragment reactions. Figure 13.8 shows measured activity distributions and measured dose distributions in CH2 target in the depth direction and in the lateral direction irradiated by mono-energy proton beams at 138, 179, and 223. The range of PET scan and the number of PET scans were changed by the energy of proton beam (see Fig. 13.8 top). Raw data of measured activity was corrected by using half-life of 11C nuclei (20.4 min) as attenuation correction, taking into consideration activity attenuation during PET measurement time. In Fig. 13.8, the intensity of activity in the depth direction which depends on a dose quantity corresponding with stopping power and flux increases gradually up to beam range with an increase of dose and then decreases sharply. In the lateral direction, shapes of activity distributions are similar to shapes of dose distributions. Since a plane at a same depth position in a homogeneous target has almost uniform energy of proton beam, shapes of both distributions depend on flux of proton at the depth position. When a target is irradiated by a proton beam of 2.5 GyE which is generally used in clinical proton therapy, the intensity of activity in the target is estimated at about 3 kB/cc.
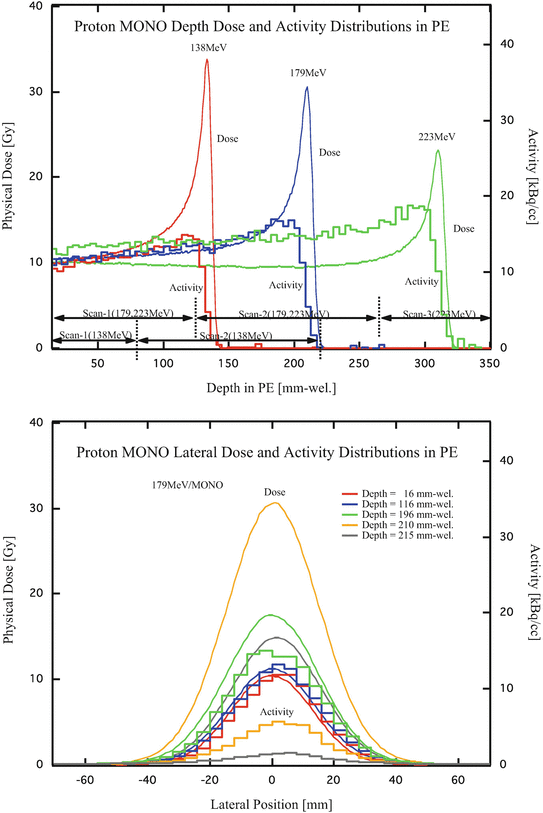

Fig. 13.8
Measured activity distributions and measured dose distributions in CH2 target in the depth direction (top) and in the lateral direction (bottom) with mono-energy proton beams at 138, 179, and 223 MeV (Nishio et al. 2005)
The flux of incident protons to a target decreases with nuclear reactions between protons and nuclei in the target as proton beam travels through the target in the depth direction. The nuclear reaction rate is determined by the total reaction cross section which depends on relative kinetic energy of protons and nuclei in the target. Figure 13.9 shows the experimental results of the total reaction cross section of protons and carbon nuclei. Semi-empirical formulas expressing experimental results approximately for the total reaction cross section have been reported by Sihver et al. (1993) and are as follows. When a target nuclear (mass number, A t , and atomic number, Z t ) is irradiated by proton beam with kinetic energy E p in the laboratory system, the total reaction cross section on the condition of E p ≥ 200 MeV is given by
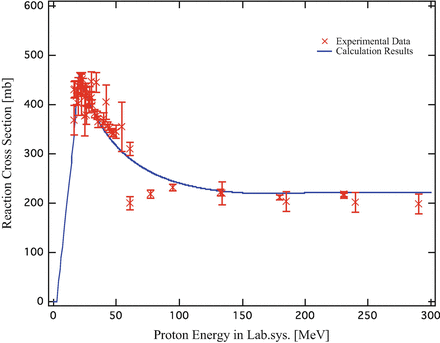

Fig. 13.9
Total reaction cross section of protons and carbon nuclei with kinetic energy of proton beam (Nishio et al. 2005)
![$$ \begin{array}{l}{\sigma}_{\mathrm{reac}}\left({E}_p,{A}_t,{Z}_t\right)={\sigma}_0\left({A}_t\right)=\pi {r_0}^2{\left[1+{A_t}^{1/3}-{b}_0\left(1+{A_t}^{-1/3}\right)\right]}^2,\\ {}{b}_0=2.247-0.915\left(1+{A_t}^{-1/3}\right),\end{array} $$](https://radiologykey.com/wp-content/uploads/2017/07/A340376_1_En_13_Chapter_Equ4.gif)
(13.4)
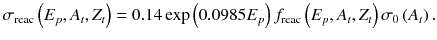
(13.5)
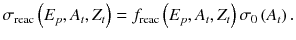
(13.6)
![$$ {\sigma}_{\mathrm{reac}}\left({E}_p,{A}_t,{Z}_t\right)=\left[{f}_{\mathrm{reac}}\left({E}_p,{A}_t,{Z}_t\right){g}_{\mathrm{reac}}\left({E}_p,{A}_t,{Z}_t\right)+{h}_{\mathrm{reac}}\left({E}_p,{A}_t,{Z}_t\right)\right]{\sigma}_0\left({A}_t\right). $$](https://radiologykey.com/wp-content/uploads/2017/07/A340376_1_En_13_Chapter_Equ7.gif)
(13.7)
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree