Fig. 7.1
Schematic of a typical shock wave pulse used in SWL. High pressure, which exceeds 40 MPa, is followed by a long tail of negative pressure. The repeated positive and negative pressure yields dynamic stress in the stone. The negative pressure also produces cavitation on the propagating path. Both the shock wave stress and collapse pressure of the cavitation bubble are important mechanisms of stone disintegration
In this chapter, high-intensity focused ultrasound (HIFU), which has a higher frequency (>500 kHz) than a lithotripsy shock wave, is used to erode kidney stones. Specifically, a tone burst of one frequency is used to generate a cloud cavitation (bubble cloud) at the surface of a kidney stone. A second lower frequency wave is used to collapse the cloud and this erodes the stone (cavitation control (C-C) waveform). We investigated the development and optimization of focused ultrasound lithotripsy using the C-C waveform (Matsumoto et al. 2002; Ikeda et al. 2006; Yoshizawa et al. 2009). Different from a lithotripter shock wave, focused ultrasound has a smaller focal volume. As the cavitation phenomenon is highly unpredictable and destructive, a fine control system is needed. We propose a control method composed of three steps for kidney stone treatment. The first step is control of localized high pressure on the stone. The second step is monitoring of cavitation activity and feedback on the optimized ultrasound conditions (Yoshizawa et al. 2009). The third step is stone tracking and precise ultrasound focusing on the stone, which is altered in accordance with respiration (Koizumi et al. 2009a, b). The three control steps are outlined, and the feasibility of a focused ultrasound lithotripter is discussed in this chapter.
7.2 Localized High Pressure on Kidney Stones
7.2.1 Cavitation Control Waveform (C-C waveform)
The two-frequency ultrasound wave of the C-C waveform used in this study is shown in Fig. 7.2. The acoustic pressure is recorded using a membrane hydrophone (MHB200B, NTR systems, Seattle, WA, United States) placed at the focus of a concave lead zirconium titanate (PZT) transducer.
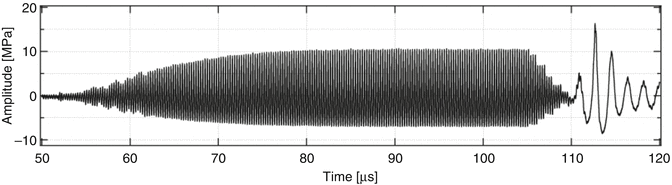

Fig. 7.2
Acoustic pressure of typical cavitation control (C-C) waveform. Ultrasound acoustic pressure was measured with a membrane hydrophone located at the focal point. The ultrasound wave is a high frequency one (3.82 MHz, 175 cycles (46 μs)), and a low frequency (545 kHz pulse (6 cycles)) ultrasound follows immediately after the high frequency wave has stopped
First, the high frequency ultrasound wave (3.82 MHz, 175 cycles in this case) is transmitted. Immediately after the high frequency wave is stopped, a short pulse of a low-frequency wave (545 kHz, 6 cycles) follows. For the high frequency wave in Fig. 7.2, the focal pressure is the maximum positive pressure |P + | = 10.0 MPa, and the maximum negative pressure |P−| = 6.0 MPa. For the low frequency part; |P+| = 16.0 MPa and |P−| = 6.5 MPa. High frequency ultrasound is designed to produce a localized cavitation bubble cloud on a stone, and low frequency ultrasound triggers the bubble cloud into violent collapse.
Cloud cavitation is a densely populated cluster of cavitation bubbles that has lost the characteristics of individual bubble behavior and the global behavior as a cluster increases. Bubbles are dynamically coupled through fluid motion and acoustic wave propagation inside the cloud (d’Agostino and Brennen 1988, 1989). Cloud collapse has been shown to be more destructive to high speed turbo-pumps and ship propellers than the collapse of individual bubbles. Numerous experimental studies (Knapp 1955; Soyama et al. 1992; Kato et al. 1996; Reisman and Brennen 1996; Reisman et al. 1998; Konno et al. 2002) and analytical/numerical studies (van Wijngaarden 1964; Mørch 1981; Omta 1987; Chahine and Duraiswami 1992; d’Agostino and Brennen 1988, 1989; Wang and Brennen 1995, 1999) have been conducted to investigate the dynamics of cloud collapse and to calculate the pressure generated in a cloud. The indirect experimental measurements of the maximum pressure inside a cloud of up to O(108 ~ 109) Pa have been reported (Kato et al. 1996; Reisman et al. 1998). Shimada et al. (2000) used a numerical model for a spherical bubble cloud considering the individual oscillating bubbles to investigate the converging shock wave within the cloud. They argued that the shock wave convergence inside the cloud led to a center bubble collapse pressure of O(109) Pa, when a bubble cloud with bubbles of radius 20 μm and a cloud radius of 5 mm was subjected to stepwise pressure decrease and increase. Their work has been extended for initial and boundary conditions appropriate for focused ultrasound applications (Yoshizawa et al. 2004; Matsumoto and Yoshizawa 2005), although the model was restricted to lower ultrasound pressure amplitudes than those used clinically. They investigated the behavior of spherical cloud cavitation with uniform bubble size and distribution excited using medical ultrasound. They also showed that a violent cavitation cloud collapse occurs with a frequency range much less than the resonance frequency of the individual bubbles inside the cloud, with a collapse pressure up to 1000 times the ultrasound pressure amplitude, which forces the cloud into strong collapse. They also showed a broadening of the resonance bandwidth when the cloud was subjected to relatively large pressure amplitude. Their results provide the basis for our method for eroding kidney stones. A one-frequency wave is used to generate a cloud of bubbles, and then a lower frequency wave excites the cloud at its broad resonance to create high collapse pressure. Due to the broad resonances of the cloud, the low and high frequencies do not need to be precisely fixed.
7.2.2 Observation of Cavitation on Stones
The cavitation phenomenon at the focus of the focused ultrasound is recorded with a high speed image converter camera, Imacon 200 (DRS Hadland; at present, DRS Data & Imaging Systems, Oakland, NJ, USA). The Imacon 200 can take 8 photographs at a rate of up to 200 million frames/s, with a 5 ns minimum exposure. This is suitable for observing cavitation in the frequency bandwidth of the experiment (0.5–4 MHz), as this corresponds to a comparably shorter exposure time than the ultrasound cycle (250 ns–2 μs) and a faster frequency than ultrasound. Interframe and exposure time are varied by the software control, and the double shutter mode of Imacon 200 can obtain 16 frames with time intervals between frames of greater than 300 μs. An air-backed ultrasound transducer with a concave PZT ceramic element (C-213, Fuji Ceramics, Japan) was fixed in an acrylic water tank. Both the aperture and focal length of the PZT element were 80 mm.
7.2.2.1 High Frequency
Acoustic cloud cavitation in the focus of the focused ultrasound is visualized for the different ultrasound frequencies of 1.08, 1.64, 2.75, 3.27 and 3.82 MHz, after 100–200 μs irradiation. Figure 7.3a–d show the bubble clouds made from four of the five different ultrasound frequencies at the focal point.
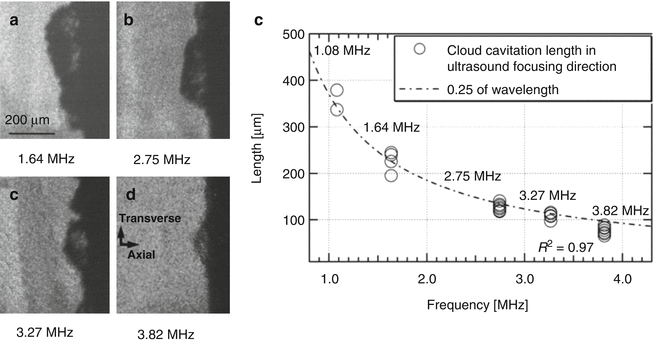

Fig. 7.3
Frequency dependence of cloud cavitation. (a–d) Cloud cavitation exhibits constant shape and size for different ultrasound frequencies. Ultrasound irradiation duration is from 100 to 200 μs. (e) Characteristic length of cloud cavitation: For different frequencies, the length of the acoustic cloud cavitation generated at the surface of the aluminium ball was measured. In every case, the ultrasound irradiation duration was 100–200 μs and the length was measured in the axial direction (parallel to the ultrasound focusing direction). The cloud cavitation length showed strong relationship with 1/4λ the ultrasound wavelength (R 2 = 0.97)
After 100–200 μs of ultrasound irradiation, the hemispherical bubble clouds maintained their shape and size. Figure 7.3e shows the measured characteristic lengths of the bubble clouds for different frequencies. This length is the maximum length of the cloud cavitation in the axial direction of focused ultrasound, i.e., the horizontal direction in Fig. 7.3a–d. The length of the cloud approximately fits one-quarter of the ultrasound wavelength. The determination coefficient R 2 is 0.97. This is consistent with the distance from the antinode (at the solid surface) to the node of the standing wave created by the incident and reflected ultrasound wave. These results demonstrate that the size of the bubble cloud generated by the focused ultrasound can be controlled with respect to the ultrasound frequency. The cavitation region is within 1 mm (axial direction) when the ultrasound frequency is higher than 1 MHz. Hence, in the focused ultrasound field, the acoustic cavitation at the solid surface can be controlled in space by altering the ultrasound frequency.
7.2.2.2 Low Frequency
The forced collapse of the bubble cloud and the shock wave emission from the cloud were also photographed. The bubble clouds, which were generated using higher frequency focused ultrasound (2.75 and 3.82 MHz, shown in Fig. 7.3), were forced to oscillate due to low frequency ultrasound (545 kHz).
Figure 7.4 shows photographs of the bubble cloud forced into oscillation. Immediately after the 100 μs irradiation of the 2.75 MHz ultrasound was stopped, the 545 kHz pulse ultrasound was focused on the cloud. The interframe and exposure times for the photograph were 325 and 50 ns. In Fig. 7.4, the first to the fifth frames correspond to one cycle of the 545 kHz ultrasound, and the sixth to the tenth frames correspond to the next cycle. In the first frame in Fig. 7.4, a hemispherical cloud cavitation was observed on the solid surface. The cloud was forced to oscillate due to the 545 kHz ultrasound. The bubble cloud shrank during the positive phase of the 545 kHz ultrasound and was forced to collapse, as shown in the fourth frame. In the fifth frame, the bubbles rebounded, and the next collapse occurred at the eighth frame. During the photographic sequence, the boundary of the cloud (the border between the water and the two-phase medium) did not significantly change its position, whereas the image density of bubbles in the bubble cloud drastically changed.
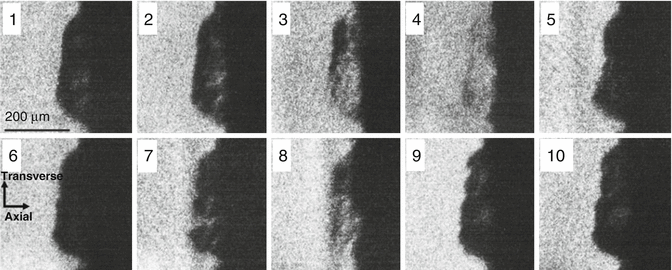

Fig. 7.4
Forced collapse of cloud cavitation: Hemispherical cloud cavitation generated due to 2.75 MHz focused ultrasound (shown in Fig. 7.3) forced into collapse by 545 kHz ultrasound. The camera interframe time was 325 ns with an exposure of 50 ns. During the first frame, the low frequency ultrasound’s second positive phase started. During the fourth frame, the bubble cloud collapsed with each bubble radii decreasing in size. At that time, high pressure was hypothesized to be localized at the solid surface. The bubbles rebounded during the fifth frame, and the next collapse occurred during the eighth frame
The phenomena exhibited in Fig. 7.4 shows the resonance phenomena as the cloud underwent oscillation. Every bubble in the cloud collapsed at approximately the same time. In the fourth frame of Fig. 7.4, it is thought that the bubbles close to the solid surface, i.e., near the center of the hemispherical bubble cloud, violently collapsed, such that a very high pressure might have occurred at the aluminum ball surface. The boundary of the cloud (interface of bubble flow and water) maintained its position, but the individual bubbles collapsed, decreasing in their respective sizes. Likewise, this result also suggests that the cloud cavitation collapse in Fig. 7.4 is related to the wave propagation of the low frequency part through the bubble flow, rather than the volumetric oscillation of the cloud cavitation region, such that the boundary of the cloud behaved like a wall of single bubbles (collapse of a bubble cloud similar to that reported previously, Pishchalnikov et al. 2003). This cloud cavitation collapsing phenomena occurred almost at the same frame for each test run, indicating the high reproducibility of the phenomena.
Figure 7.5 shows a shadowgraph photograph of the bubble collapse. The shock wave emitted from cloud cavitation (produced by the C-C waveform) is visualized. The high frequency (3.82 MHz, 175 cycles) wave was immediately followed by the 545 kHz ultrasound. In the first frame of Fig. 7.5, low-frequency ultrasound just reached the bubble clouds on the kidney stone. In the second frame, upon the low frequency hit on the bubbles, the bubbles were simultaneously forced into collapse. The sizes of the bubble in the images are very small (almost disappeared). In the third frame, a strong shock wave shadow, with a high contrast to background, was emitted from the cloud cavitation. In the fourth frame, hemispherical shock wave propagated out of the imaging area. This shock wave emission was photographed in the same frame for different test runs, again demonstrating the reproducibility of the phenomena. These results suggest that the C-C waveform successfully localizes the cavitation bubbles on the stone and controls the high collapse pressure of cloud cavitation acting on the stone surface.
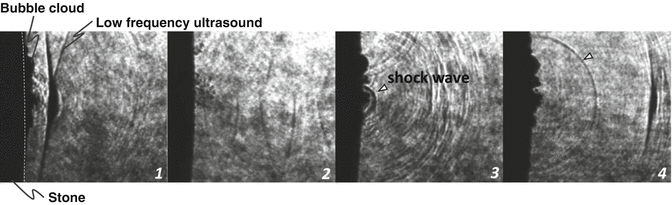

Fig. 7.5
Shock wave emission from cloud cavitation. Forcing ultrasound in C-C waveform (Fig. 7.2, high frequency 3.82 MHz, low frequency 545 kHz). The camera interframe time was 105 ns with an exposure of 5 ns. The photograph time corresponds to the low frequency wave immediately focused after the high frequency was stopped. Shortly after the second positive phase of the 545 kHz ultrasound hit the cloud cavitation (first frame), the bubble cloud collapsed in the second frame, then a spherical shock wave propagated outwards from the cloud cavitation (third and fourth frames)
7.2.3 Stone Fragmentation
Stone-erosion tests were conducted to investigate the applicability and efficiency of C-C waveform for lithotripsy applications. The erosion rate was also determined to investigate the efficiency of cavitation erosion in stone disintegration. The model stones are the test materials that were previously developed for research with commercial SWL machines. The U-30 stones were supplied by McAteer et al. (2005). These stones were cylindrical and longer (6.4 ~ 10.1 mm) than the wavelength of ultrasound (0.38 ~ 3.0 mm), and a -6 dB beam width of the focused ultrasound (0.6 ~ 4.0 mm). It took 72 h to completely rehydrate the U-30 stones in the atmospheric pressure (McAteer et al. 2005). Since the trapped gas inside the stone potentially affects the amount of erosion and distorts the acoustic profile, every stone was dipped in a desiccator filled with degassed water maintained at 10 kPa. They were left to stand for more than 48 h until there were no observable gas bubbles rising from the stone surfaces.
The acoustic waves for the stone-erosion tests were designed to demonstrate the advantage of the C-C waveform, which has combined high and low frequency waves. The C-C waveform was compared with waves with only a high or low frequency wave. The test waves for stone erosion were as follows: (a) High frequency alone (3.82 MHz, 175 μs), (b) low frequency alone (545 kHz, 6 cycles), and (c) high frequency and low frequency combined (C-C waveform). The ultrasound wave of the C-C waveform is shown in Fig. 7.2, and was also used in the experiment shown in Fig. 7.5.
In each case, the pulse repetition frequency (PRF) of the waves transmitted from the PZT transducer was fixed at 25 Hz, such that a 39.94 ms interval elapsed before the next pulse of the C-C waveform (c) (39.95 ms for (a) and 39.99 ms for (b)).
The upper graphs in Fig. 7.6 show the measured weight losses of the U-30 stones for each wave. The ultrasound irradiation time was 3, 6, 12, 18 min. Two samples were tested for each wave and irradiation time. The lower photographs correspond to the eroded model stones for each ultrasound irradiation time for the C-C waveform. In this experiment, each stone was moved in an attempt to achieve erosion over the entire stone surface. Two operators moved the stones while monitoring them with a microscope equipped with an ocular ruler with 0.1 mm resolution. In the transverse direction, the operators moved the stone so that the focus entirely covered the whole area of the stone’s base circle. The round trip of diameter scanning was maintained at 4 s. In the axial direction, the stones were also moved as the ultrasound focus was maintained on the base circle. This stone-erosion test protocol was applied for every sample (48 samples). The erosion rate was calculated using the least square fit of linear regression between the weight loss and ultrasound irradiation time. The calculated erosion speeds of the C-C waveform, low frequency alone and high frequency alone were 0.3, 2.2 and 5.0 mg/min, respectively. The determination coefficient R 2 between the weight loss and ultrasound irradiation time was 0.66, 0.91 and 0.99, respectively.
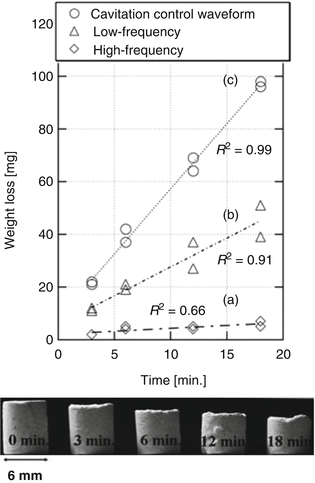

Fig. 7.6
Erosion rate measurement using U-30 model stones. The weight losses for different times were measured for different waves; (a) high frequency wave (3.82 MHz) alone, (b) low frequency wave (545 kHz) alone, and (c) C-C waveform. The PRF of each ultrasound wave was 25 Hz. The lines in the upper figures are the results of the least square fit of linear regression between the weight loss and ultrasound irradiation time (R 2 is also shown). The lower photographs correspond to the eroded model stones for each ultrasound irradiation time for the C-C waveform
As shown in Fig. 7.6, the C-C waveform eroded the stones more efficiently compared with the high frequency alone and low frequency alone waves. The calculated erosion rate reveals that the erosion rate of the C-C waveform (c) is double the rate of the sum of the high frequency alone (a) and low frequency alone (b). The high frequency causes localized cloud cavitation on the stone surface, but the amount of erosion is small, possibly because the cloud cavitation shields the stone surface. The low frequency wave alone creates a wider region of cavitation bubbles, so the eroded region is wider than the high frequency alone case. These bubbles collapse independently, such that the maximum pressure is not as high in comparison to the collapse of the cloud cavitation. The C-C waveform, with the high and low frequency in combination, forces the bubble cloud into a violent collapse, and the C-C waveform’s advantage might be a result from the controlled cloud cavitation collapse on the stone surface.
Ultrasound irradiation of the C-C waveform was applied to two types of natural kidney stones: Cystine and staghorn. The ultrasound wave was the same as that in Fig. 7.2, and the PRF of the cycle was 25 Hz. The upper photographs in Fig. 7.7 are of the eroded stones and their fragments, (a) cystine stone and (b) staghorn stone.
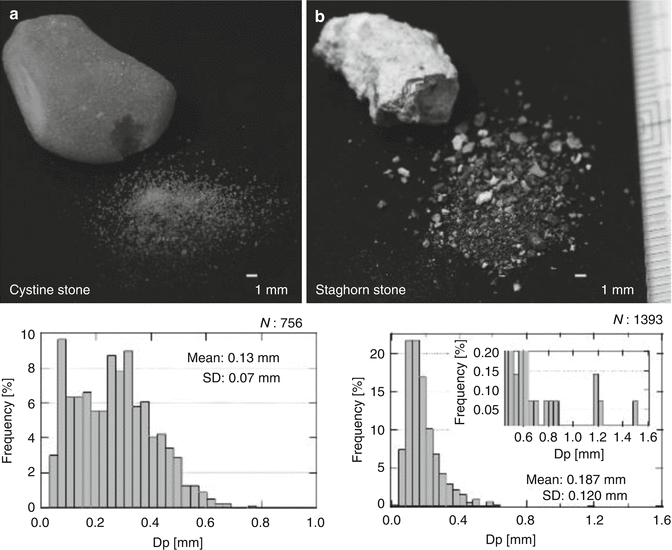

Fig. 7.7
Natural kidney stones and their fragments after irradiation by C-C waveform. (a) Cystine stone and fragments, (b) staghorn stone and fragments. Upper figures are photographs of each stone and lower figures are the size-frequency of the number-size distribution of fragment diameter D p. The resulting fragments were typically less than 1 mm in diameter. Three fragments of D p = 1.2 ~ 1.5 mm were calculated for the staghorn stone
Erosion holes can be seen in both. The lower figures are the size-frequency of the number-size distribution of the fragment diameter D p for (a) cystine stone and (b) staghorn stone. The D p was calculated from the binary processed image of photographed stone fragments. From the area and peripheral length of the fragments, the D p was calculated for each particle. For the cystine stone, the mean diameter was 0.13 ± 0.070 mm for 756 fragments, and 0.187 ± 0.120 mm for 1393 fragments of the staghorn stone. Every cystine stone particle was less than 0.8 mm. Almost all the staghorn fragments were less than 1.0 mm, although three fragments of D p =1.2 mm, and one particle of D p = 1.5 mm, were observed. The size distribution for each stone had slightly different characteristics. The staghorn stone fragments had a typical size-frequency distribution of fragments, with a long tail for increasing fragment size. The cystine stone’s distribution also had a long tail for the larger fragment sizes, whereas the small fragments did not have a local maximum. This is possibly due to the fact that very small fragments could not be completely collected due to water convection in the tank, so they did not all fall into the beaker placed just beneath the stone.
Almost all the eroded fragments were less than D p = 1 mm, except the three fragments of D p = 1.2–1.5 mm for the staghorn stone. These results show one of the main advantages of using cavitation erosion. Using cavitation erosion, fragmentation is expected to result in small stone fragments (1–2 mm) of clinically passable size from the initial stage of treatment. Cystine stones have been reported to be the most difficult to break by SWL. Zhong et al. (1992) argues that cystine stones are non-breakable due to their ductility in spite of their small surface hardness value. Figure 7.7 shows that the cystine stone was eroded by cavitation from the surface into small fragments of less than 1 mm.
Finally, the estimated acoustic power of the C-C waveform is in the range between the intensity of the Power Doppler (15 W/cm2) and typical HIFU for the treatment of tumors (500–2000 W/cm2) (ter Haar 2001; Bailey et al. 2001). Although the intensity was small compared with that of HIFU treatment, and there is the large time interval between C-C waveform pulses, the effect of unwanted tissue heating should be examined through in-vivo studies.
7.3 Ultrasound Lithotripsy with Cavitation Monitoring
7.3.1 Experimental Setup and Ultrasound Sequence for Subharmonic Detection
In the experiment discussed in Sect. 7.2, the cavitation activity in the C-C waveform sequence was well visualized and seemed to be well controlled in-vitro in degassed and distilled water. Whereas in the human body, the cavitation phenomena should be different and unpredictable because the physical property of the medium in which the stone exists is totally different. Thus, specially designed cavitation monitoring is necessary to optimize stone comminution under in-vivo conditions.
Passive and active cavitation detection has been investigated through SWL (Coleman et al. 1996; Cleveland et al. 2000a, b; Bailey et al. 2005). In two-frequency ultrasound lithotripsy using the C-C waveform, it is important to detect cavitation generation as stone erosion efficiency by the low frequency ultrasound wave depends on the state of cavitation bubbles induced by the high frequency wave. Cavitation bubbles were generated on a stone in a small region from the high frequency wave, as shown in Fig. 7.3, and acoustic emission intensity from the bubbles during high frequency exposure was much lower than that of the reflected ultrasound from the stone surface. Therefore, a sensitive detection method is required. The subharmonic ultrasound signal is a specific frequency component emitted from highly, nonlinear scatter, such as cavitation bubbles. The subharmonic detection method and the relation between the signal amplitude and stone erosion volume (Yoshizawa et al. 2009) are introduced in this section.
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree