where D T = absorbed dose (Gy) in target organ T
à S = the number of nuclear transitions in a source organ S, sometimes referred to as the “cumulated activity” (MBq-s)
n i = number of radiations with energy E i emitted per nuclear transition
E i = energy per radiation (MeV)
ϕ i f(T←S) = fraction of energy emitted that is absorbed in target organ T from source organ S for radiation i
m T = mass of target region (kg)
k = a proportionality constant (e.g., Gy-kg/MBq-s-MeV)
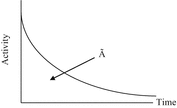
The term à is the integral of the time-activity curve for a “source” organ, i.e., an organ that exhibits a significant uptake of the administered activity. As becquerel is the number of disintegrations per second that occur at a given time, the integral over time is Bq-s (MBq s was used above), which is the total number of disintegrations that occurred in the organ over the period of integration (almost always to infinite time). The activity at any given time is determined in the biokinetic analysis; data are obtained in animals or human subjects at specified times after administration of the radiopharmaceutical, and then these data are integrated over time to give Ã. First, a review of how to obtain the biokinetic data will be provided, and later we will return to show some actual sample dose calculations.
3.3 Biokinetic Analyses
- (i)
Study design
Time-activity curves for most organs are usually characterized by one or more exponential terms, i.e.,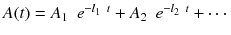 . The rate constants λ 1 and λ 2 describe the kinetics of removal of activity components A 1 and A 2; we can define half-times T 1 and T 2, by λ = 0.693/T; the half-time is the time needed for half of that component of activity to be removed from an organ. Each exponential term has two unknown variables; therefore, the time-activity curve has to have two data points for each phase of clearance for which we wish to define an exponential term. So, if a given time-activity curve is best characterized by two exponentials, an absolute minimum of four data points is needed to define the curve, with two points during each phase (i.e., not one during the first phase and three during the second). The starting point in a kinetic analysis is thus deciding on when to obtain samples, whether they are from a study involving animals or human subjects. For a completely new agent, this can obviously be a challenge, as the biokinetics are not yet known. An absolute limitation is the physical half-life of the radionuclide employed; F-18 has a half-life of 1.83 h, so data may be gathered over several hours after administration, but C-11 has a half-life of only 20 min, so data must be gathered much more quickly. Then, the biological clearance of the agent must be considered. The equation above with the exponential terms was not explicit, but the complete removal of activity from an organ or the body is due to both radioactive decay and biological clearance; this is characterized by a rate constant λ e, an “effective rate constant,” which is the sum of the biological rate constant (λ b) and the physical rate constant (λ p). Some agents are cleared very quickly, others more slowly. So the study must be planned to get the needed number of data points taking into account both physical and biological clearance. Often experience with other similar agents can be helpful, or the data points can be spaced out over the range of times possible over several physical half-lives of the radionuclide, with several early time points and several later time points. There is not an exact formula for guiding the selection process of sampling times; care must be taken to provide enough data before spending the time and money on gathering the data so that the data collection process will be successful and the experiments will not have to be repeated. In addition to activity levels in organs and the body, an essential element of a biokinetic analysis is evaluation of the excretion of activity from the body (via the urinary or intestinal pathway). It is not uncommon for excretory organs to receive the highest doses of any other organ in the body, as often 100 % of the activity eventually passes through these organs (urinary bladder or intestines). For PET radiopharmaceuticals, excretion may be more limited than for other agents, due to their short physical half-lives. For C-11 compounds, for example, it is common to assume that there is no time for appreciable excretion from the body to occur, although activity might accumulate in the urinary bladder and decay there. So for studies using imaging data, if activity is seen in the urinary bladder, the activity levels may be quantified, and the activity may be integrated to get the number of disintegrations in the urinary bladder; collecting urine data is unlikely to provide any useful information. For F-18, it is common for there to be urinary excretion during the duration of the study, and the use of image data or urine collection may be helpful.
. The rate constants λ 1 and λ 2 describe the kinetics of removal of activity components A 1 and A 2; we can define half-times T 1 and T 2, by λ = 0.693/T; the half-time is the time needed for half of that component of activity to be removed from an organ. Each exponential term has two unknown variables; therefore, the time-activity curve has to have two data points for each phase of clearance for which we wish to define an exponential term. So, if a given time-activity curve is best characterized by two exponentials, an absolute minimum of four data points is needed to define the curve, with two points during each phase (i.e., not one during the first phase and three during the second). The starting point in a kinetic analysis is thus deciding on when to obtain samples, whether they are from a study involving animals or human subjects. For a completely new agent, this can obviously be a challenge, as the biokinetics are not yet known. An absolute limitation is the physical half-life of the radionuclide employed; F-18 has a half-life of 1.83 h, so data may be gathered over several hours after administration, but C-11 has a half-life of only 20 min, so data must be gathered much more quickly. Then, the biological clearance of the agent must be considered. The equation above with the exponential terms was not explicit, but the complete removal of activity from an organ or the body is due to both radioactive decay and biological clearance; this is characterized by a rate constant λ e, an “effective rate constant,” which is the sum of the biological rate constant (λ b) and the physical rate constant (λ p). Some agents are cleared very quickly, others more slowly. So the study must be planned to get the needed number of data points taking into account both physical and biological clearance. Often experience with other similar agents can be helpful, or the data points can be spaced out over the range of times possible over several physical half-lives of the radionuclide, with several early time points and several later time points. There is not an exact formula for guiding the selection process of sampling times; care must be taken to provide enough data before spending the time and money on gathering the data so that the data collection process will be successful and the experiments will not have to be repeated. In addition to activity levels in organs and the body, an essential element of a biokinetic analysis is evaluation of the excretion of activity from the body (via the urinary or intestinal pathway). It is not uncommon for excretory organs to receive the highest doses of any other organ in the body, as often 100 % of the activity eventually passes through these organs (urinary bladder or intestines). For PET radiopharmaceuticals, excretion may be more limited than for other agents, due to their short physical half-lives. For C-11 compounds, for example, it is common to assume that there is no time for appreciable excretion from the body to occur, although activity might accumulate in the urinary bladder and decay there. So for studies using imaging data, if activity is seen in the urinary bladder, the activity levels may be quantified, and the activity may be integrated to get the number of disintegrations in the urinary bladder; collecting urine data is unlikely to provide any useful information. For F-18, it is common for there to be urinary excretion during the duration of the study, and the use of image data or urine collection may be helpful.
- (ii)
Animal data
Preclinical studies are required for any New Drug Application (NDA) to the US Food and Drug Administration (FDA). Most research is done with some rodent species, but any animal species in theory is acceptable. Some have an inclination to use primate species, with the idea that they may produce results more similar to humans. Extrapolation of animal data to humans has produced misleading information in many cases, in most any animal species [2]. So, this is a necessary step in the process of evaluating the dosimetry of a new radiopharmaceutical, but one should bear in mind that the real dosimetry will not be known until well-designed and executed studies using human subjects are performed. Time-activity curves for radiopharmaceuticals using animals may be established by administering the radiopharmaceutical and either sacrificing the animals, extracting tissue samples, and performing a radioassay or by small animal imaging studies.
- (a)
Tissue extraction – using a minimum of three animals per time point, individual samples of organs and tissues may be extracted from the animals after sacrificed and counted in any radiation detector system (e.g., sodium iodide scintillation, liquid scintillation). Collection of urine and/or fecal samples via the use of “metabolic cages” may characterize the excretion of the agent. Extrapolating the organ/tissue data to humans is not an exact science. One may assume that the percent of the administered activity seen in any organ at a given time will likely be the same concentration seen in humans; one may say that this is a “direct extrapolation.” One may assume that the percent of administered activity per gram in an organ will be the same in humans; due to the normally considerable differences in body and organ masses, this is likely to produce erroneous results. Many have evaluated various extrapolation methods proposed in the literature. One method of extrapolating animal data that has been applied by many is the % kg/g method [3]. In this method, the animal organ data need to be reported as % of injected activity per gram of tissue, and this information plus knowledge of the animal whole body weight is employed in the following extrapolation:
![$$ {\left(\frac{\%}{\mathrm{organ}}\right)}_{human}=\left[{\left(\frac{\%}{{\mathrm{g}}_{\mathrm{organ}}}\right)}_{\mathrm{animal}}\times {\left({\mathrm{kg}}_{TB\kern0.5em weight}\right)}_{animal}\right]\times {\left(\frac{{\mathrm{g}}_{organ}}{{\mathrm{kg}}_{TB\kern0.5em weight}}\right)}_{\mathrm{human}} $$](https://radiologykey.com/wp-content/uploads/2017/10/A328756_1_En_3_Chapter_Equb.gif)
The percent uptake per gram of tissue is multiplied by the animal whole body weight in kg; the percent in any organ in humans is obtained by applying the corresponding organ and body masses of a reference adult human. A numerical example using this method was provided by Stabin [2] (Table 3.1):
Table 3.1
Animal data extrapolation example (mass extrapolation)
Animal
Source organ
1 h
3 h
6 h
16 h
24 h
%ID/organ
3.79
3.55
2.82
1.02
0.585
(%ID/g)
38.1
36.6
30.8
11.3
5.70
Human
%ID/organ
3.26
3.12
2.63
0.962
0.486
The animal whole body weight was 20 g (0.02 kg), and the source organ chosen had a mass of around 299 g. The human total body weight for the standard adult male of 70 kg was then applied in the transformation. For example:
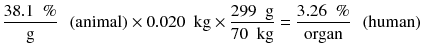
The idea of this method is that the percent in an organ is weighted for the fraction of total body mass that the organ comprises. As noted above, this is not a “gold standard” method by any means; it is an attempt to perform a reasonable extrapolation that avoids some pitfalls in other methods. Some also suggest adding a scaling in time, to account for the different metabolic rates of species of different size:
where t a is the time at which a measurement was made in an animal system, t h is the corresponding time assumed for the human data, and m a and m h are the total body masses of the animal species and of the human. Again from Stabin [2], Table 3.2 shows an example case with data extrapolated from an animal species to the human using this time scaling approach:
![$$ {t}_h={t}_a{\left[\frac{m_h}{m_a}\right]}^{0.25} $$](https://radiologykey.com/wp-content/uploads/2017/10/A328756_1_En_3_Chapter_Equd.gif)
Table 3.2
Animal data extrapolation example (time extrapolation)
Animal time scale
5 min
15 min
30 min
60 min
1.5 h
Extrapolated human time scale
22 min
1.1 h
2.2 h
4.3 h
6.5 h
Here, a human body mass of 70 kg was used, and the animal whole body mass was assumed to be 200 g. Sparks and Aydogan [4] studied the success of animal data extrapolation for several radiopharmaceuticals, using direct extrapolation, and mass and/or time extrapolation. They found that no particular method was superior to any other and that, in many cases, extrapolated animal data significantly underestimated observed uptakes in human organs. So, in conclusion, choice of an animal species and extrapolation method are areas of freedom in designing an animal study, and results obtained from animal studies must be recognized as only preliminary estimates of the dosimetry for any radiopharmaceutical.
- (b)
Small animal imaging
Small animal imaging techniques have greatly improved the science of drug development. It has also allowed the characterization of radiopharmaceutical dosimetry in living animals, eliminating the need to sacrifice them. A drawback, however, is that the animals generally need to be anesthetized for the imaging session. Anesthetics may alter the distribution of the radiopharmaceutical, leading to inaccurate evaluation of organ uptakes and subsequent dosimetric analyses. Quantitative analyses of organ uptake at any imaging time are the same as those from human imaging studies. PET images are inherently quantitative; counts in a given voxel are easily related to absolute values of activity. Drawing volumes of interest (VOIs) over recognized organ regions provides values of activity in the organ that can be related to percentages or fractions of the administered activity, which is always known. Thus time-activity curves can be readily (but not easily!) developed from the image data and integrated to obtain the time-activity integrals needed for development of dose estimates. Organ and whole body biokinetics, combined with analyses of excretion, will allow development of a complete dosimetric analysis.
- (c)
Small animal dosimetry
Some researchers have expressed interest in calculating dose to animal organs. As with the development of human dosimetry phantoms, to be discussed below, early efforts involved animal dosimetry phantoms comprised of geometric shapes to describe organs and progressed to more realistic, image-based voxel phantoms. Yoriyaz and Stabin [5] constructed a geometric model of the mouse and generated dose factors (DFs) for a selected number of source and target pairs for 213Bi and 90Y. Muthuswamy et al. [6] developed a dosimetric model of mouse marrow and provided DFs for 131I, 186Re, and 90Y. Konijnenberg et al. [7] developed a stylized representation of Wistar rats and performed Monte Carlo calculations to develop DFs for several radionuclides. With the advent of small animal imaging technologies, it became possible to move away from the use of stylized, equation-based body models and develop representations that more realistically define organ size, shape, and proximity. Kolbert et al. [8] used MR images of a female athymic mouse to develop realistic models of several organs and estimated self-dose and cross-dose values for these organs. Hindorf et al. [9] developed a model of a mouse using geometric shapes to define ten organs and then converted the model to a voxel format. Stabin et al. [10] segmented micro-CT images of a mouse and rat and developed specific absorbed fractions (SAFs) for photon and electron sources within the animal organs and DFs for several source and target regions. Keenan et al. [11] developed three mouse and five rat dosimetric models, using the MOBY and ROBY models developed by Segars and Tsui [12] and provided photon and electron absorbed fractions and dose factors for several radionuclides. These animal models have been incorporated into the OLINDA/EXM dosimetry code [13], thus facilitating dose calculations for these eight small animal types.
- (a)
- (iii)
Human data
Imaging studies with either patients or healthy volunteers, required by the FDA for new drug approval (strict dose limits for new drugs do not exist, but dosimetry must be presented and is usually compared to other similar agents), need to be done according to the same requirements for number and spacing of time points as described above. The two basic imaging methods are planar imaging and tomographic imaging. For PET radiopharmaceuticals, tomographic methods are by far the most common approach, so an extensive description of planar methods will not be presented here. Briefly, anterior and posterior images are taken, regions of interest (ROIs) are drawn over recognized organs, and corrections for attenuation and scatter should be made [2]. Relating counts in an ROI to absolute values of activity is usually not straightforward, unless calibration factors have been developed from phantom image analysis, which is not common. Usually counts in the total body at the earliest imaging time, corrected for radioactive decay, are used to define 100 % of the administered activity, and counts in the body and organs at later times are related to this value. In tomographic images, as for animal studies, counts in voxels associated with an organ may be related to absolute values of activity via a calibration factor, and time-activity curves may be developed from this information. PET images are inherently quantitative, as PET analyses evaluate absolute levels of activity in organs and tumors (standardized uptake values, SUVs). In contrast, few centers practice quantitative analyses with single-photon emission computed tomography (SPECT); Dewaraja et al. [14] outlined the many steps required to obtain quantitative SPECT data for therapy dosimetry calculations, including choice of collimator, choice of energy windows, reconstruction methods, attenuation and scatter correction, dead time corrections, compensation for other image-degrading effects, choice of target regions, corrections for dose nonuniformities, and other aspects to be considered to obtain quantitative information in individual voxels used to define source and target regions.
- (iv)
Kinetic analyses
The result of the biokinetic analyses described above is a series of values of percent or fraction of administered activity over time (Fig. 3.1). Values of doses for individual organs rely on the integration of these values over time. The most common method for performing this integration is to fit a function comprised of one or more exponential terms to the data. One may also integrate the data “directly,” i.e., by a “trapezoidal” integration, simply directly calculating the area under the curve between any two time points and adding up the values. A drawback of this approach is that estimating the area under the curve after the last data point is complicated; various approaches include assuming only radioactive decay after the last point, using a straight line defined by the last two or three data points, and other approaches. When an exponential function is fit to the data, the time-activity integral is easily calculated. If the function is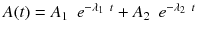 , and the values of λ are effective rate constants (including both radioactive decay and biological removal), the integral of this function to infinite time is just A 1/λ 1 + A 2/λ 2. If values of A are in MBq and values of λ have units of s−1, then the integral has units of MBq-s or millions of disintegrations. These integrals are often normalized to the amount of activity administered (MBq), so that doses are developed per unit administered activity. The units of this normalized integral are thus MBq-s/MBq. It is tempting to think of this as having units of time (here s), but this is not any measure of time.
, and the values of λ are effective rate constants (including both radioactive decay and biological removal), the integral of this function to infinite time is just A 1/λ 1 + A 2/λ 2. If values of A are in MBq and values of λ have units of s−1, then the integral has units of MBq-s or millions of disintegrations. These integrals are often normalized to the amount of activity administered (MBq), so that doses are developed per unit administered activity. The units of this normalized integral are thus MBq-s/MBq. It is tempting to think of this as having units of time (here s), but this is not any measure of time.