Clinical Applications and Theoretical Principles: Primer on Physics of MR and Hardware
Maxim Zaitsev
Michael Markl
Introduction
Nuclear Spin and Magnetic Resonance
Magnetic resonance imaging is based on nuclear magnetic resonance (NMR) as a primary means of generating signal. The phenomenon of NMR was independently observed by two groups in the United States in 1946: One at Stanford under the leadership of Felix Bloch1 and the other at MIT led by Edward Purcell.2 Both observations were made within a few days of each other and were reported in the same issue of Physical Review. NMR has quickly gained importance as a powerful analytic tool and brought scientists working in the area several Nobel Prizes, including one to Bloch and Purcell in 1952.
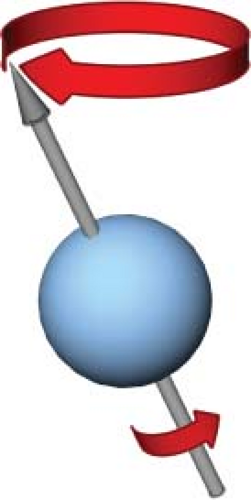
It is a basic property of nuclei with an odd number of protons or neutrons to have a nonzero angular momentum and an associated magnetic moment. The phenomena of nuclear spin and magnetic moment have a purely quantum nature. For nuclei with a spin number of one-half, however, such as protons of hydrogen atoms predominantly used in magnetic resonance imaging (MRI), a classical description suffices. In a classical picture a nucleus is modeled as a charged particle rotating around its axis, where the mass and the rotation velocity of the particle define the angular momentum, and the charge and rotation are responsible for the magnetic moment. In a simpler, more intuitive picture the spinning charged particle is often replaced by a microscopic magnet (Fig. 4.1).
The magnetic moment of nuclei becomes important once they are exposed to an external magnetic field. Without an external field the elementary magnetic moments have no preferential direction. Upon introduction of the external magnetic field, often termed B0 in MRI, nuclei tend to align their magnetic moments with the field to minimize their energy state.
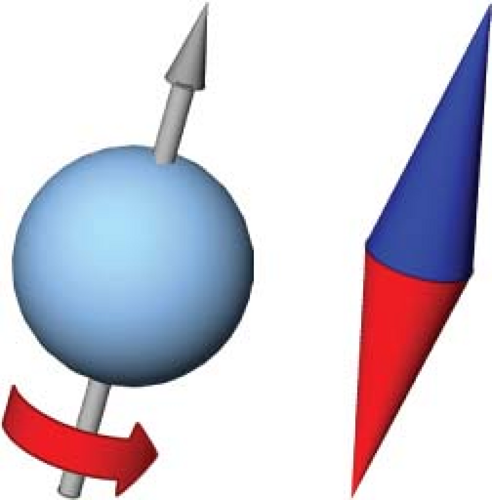 FIGURE 4.1. Illustration of the semiclassical model of a spin as a spinning charged particle (left) and a corresponding simplified magnet arrow representation (right). |
If the orientation of a nucleus is perturbed, the external magnetic field B0 exerts a torque on the magnetic moment of the nucleus aligning it back to the field’s direction. At this point the mechanical angular momentum of the nucleus becomes important. It is helpful to draw an analogy to a spinning top toy: If its rotation axis is tilted, the gravity exerts a torque attempting to increase the tilt angle. Because of its angular momentum, though, the toy spinning top does not fall, but rather starts to precess around the vertical axis. A similar effect is observed with a spin in a magnetic field: It does not immediately align with the B0 field, but rather starts to precess about its direction (Fig. 4.2). This phenomenon is termed Larmor precession, named after Irish physicist Joseph Larmor. The frequency of Larmor precession is independent of the orientation of the nuclear magnetic moment and is solely defined by the external magnetic field and the quantity, termed gyromagnetic ratio, which is the proportionality constant between the magnetic moment and the angular momentum of a given nucleus:
where the negative sign reflects the fact that for nuclei with a positive γ the precession is clockwise. A precessing magnetic moment produces an oscillating magnetic field, which can be detected with appropriate instrumentation (e.g., a loop coil placed close to the sample). The Larmor equation lays out the basics of the NMR phenomenon: Exposed to the external magnetic field, nuclei with a nonzero spin gain the ability to absorb and irradiate electromagnetic waves with a frequency characteristic to the given nucleus and proportional to the applied magnetic field. Remarkably, the classically derived Larmor precession frequency corresponds to the energy difference in spin-up and spin-down transitions in a quantum-mechanical description, which underlines the validity of the semiclassical approach for nuclei with spin of one-half. Table 4.1 lists parameters of some common elements relevant for in vivo applications of magnetic resonance. As seen, at the field strengths typical for whole-body human
applications, resonance frequencies of the relevant nuclei lie in the FM radio range. Therefore, relevant signals and pulses are referred to as radiofrequency (RF) pulses and signals.
applications, resonance frequencies of the relevant nuclei lie in the FM radio range. Therefore, relevant signals and pulses are referred to as radiofrequency (RF) pulses and signals.
Because the energy difference between the parallel and antiparallel spin alignments is vanishingly small compared to the kinetic energy of thermal motion, the ordering of the spins within the sample is strongly suppressed. Nuclear polarization (i.e., the measurable difference in magnetization between spins aligned parallel and antiparallel to B0) in a water sample at physiologic temperatures exposed to a magnetic field of 1 Tesla reaches only about 3.3 ppm (parts per million). Luckily, owing to the high number of particles present in a sample on the order of 1023, even this tiny polarization creates a detectable macroscopic magnetization. Noteworthy is that this magnetization increases linearly with the strength of the B0 field, which gives a rationale of using the ever higher magnetic fields as this would proportionally increase the available signal. Complete analysis of the signal behavior should also include the signal reception process, dependence of the measurement noise, and other experimental conditions; however, in a first approximation, the signal-to-noise ratio (SNR) in MRI increases linearly with the field strength. An increase in the main magnetic field strengths used in the diagnostic MRI is limited, however, by multiple factors, such as technical constraints in magnet design, magnetic field inhomogeneities induced by the body owing to its magnetic susceptibility, changing signal relaxation conditions, difficulties with a homogeneous signal excitation, and associated safety constraints. At present, 1.5T is considered standard in clinical settings, 3T is very common for neurologic and dynamic imaging applications, and 7T and above belong to a research area.
TABLE 4.1 GYROMAGNETIC RATIOS, LARMOR FREQUENCIES, AND RELATIVE NATURAL ABUNDANCES of SOME COMMON ELEMENTS DETECTABLE WITH MRI | ||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
The equilibrium magnetization condition can be perturbed by applying an additional magnetic field oscillating at the Larmor frequency and thus meeting the resonance condition. A general resonant system being exposed to the external interaction meeting the resonance condition has a property of adsorbing the external energy and conserving it within the internal oscillatory degrees of freedom. For the classical model of a spin, it means that the precession of the nuclear magnetization is observed at an ever increasing angle between the magnetization and the B0 field direction. The trajectory of the tip of the magnetization vector is thus a spiral placed on the surface of an imaginary sphere (Fig. 4.3A). It is instructive to consider the evolution of the magnetization in a coordinate system that rotates together with the magnetization around the direction of B0 at exactly the Larmor frequency. It is common in NMR to select coordinate systems such that the z-axis is aligned with B0. The rotating coordinate frame will thus share the z-axis with the laboratory frame. Because spins always remain on resonance and the rotating coordinate frame compensates for their precessional motion, the trajectory of the magnetization transforms from a spiral into a simple arch (Fig. 4.3B). Optimal experimental conditions are met if the RF field is applied such that its magnetic component, termed B1, is orthogonal to B0 and is circularly polarized to become stationary in the rotating frame (the non-circularly polarized RF component can typically be ignored). Under the action of such RF field, the spin magnetization, when observed in the rotating frame, is rotated away from the equilibrium
state (Mxy = 0, Mz = M0) around the direction of the applied B1 field at a constant rate. An interesting condition is reached if the B1 field is switched off once Mz = 0 and Mxy = M0, which corresponds to a 90-degree rotation of the initial magnetization. By doubling the B1 amplitude or the pulse duration, it is also possible to achieve a 180-degree rotation. In a case when B1 is constant for the whole pulse duration, a tip angle (also known as a flip angle) can be defined as:
state (Mxy = 0, Mz = M0) around the direction of the applied B1 field at a constant rate. An interesting condition is reached if the B1 field is switched off once Mz = 0 and Mxy = M0, which corresponds to a 90-degree rotation of the initial magnetization. By doubling the B1 amplitude or the pulse duration, it is also possible to achieve a 180-degree rotation. In a case when B1 is constant for the whole pulse duration, a tip angle (also known as a flip angle) can be defined as:
where B1 is the magnitude of the RF field and t is an RF pulse duration.
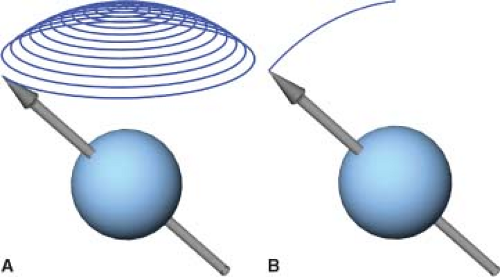 FIGURE 4.3. Action of radiofrequency pulses (A) in the laboratory and (B) in the rotating frame of reference. |
Initial NMR experiments were performed in a continuous-wave mode, where the resonance of the nuclei was detected by their ability to adsorb RF energy when the resonance frequency was met. A key property of NMR, however, which makes it particularly suitable for imaging, is its ability to operate in a pulsed mode. A pulsed NMR experiment consists of an application of a short RF pulse that tips the spins from the equilibrium state followed by a signal reception period where the free Larmor precession of the spins can be observed. Because of relaxation processes this precession does not last infinitely long, causing the corresponding signal to decay with time. Therefore, the signal observed in a pulsed NMR experiment is often called free induction decay (FID).
In 1973 the ability of NMR to separate signals originating from different spatial regions within a sample was discovered by two independent groups: Lauterbur3 at the State University of New York at Stonybrook, who was the first to publish an NMR image, and Mansfield and Grannell,4 who were involved in NMR “diffraction” studies at Nottingham University. These two works have paved the way for the development of NMR imaging methods. The original term “Zeugmatography” coined by Lauterbur to denote the new tomographic imaging technique did not find a wide acceptance. For obvious reasons, in particular to underline the noninvasive character of the new imaging modality, the word “nuclear” was dropped from the acronym “NMR,” resulting in the nowadays commonly accepted terms magnetic resonance imaging, MR imaging, or MRI.
MR Hardware
Main Magnetic Field
The process of imaging using the magnetic resonance phenomenon involves several hardware components. The main magnetic field is required to polarize the spins and produce the nuclear magnetization. This field needs to be strong, on the order of several Tesla, and at the same time very homogeneous, with the magnetic field deviations over the size of the imaged object on the order of a few parts per million (ppm) of the main field strength. This high homogeneity is required both to create long-lived FID signals with narrow spectra and to have a well-defined starting point for spatial encoding and signal localization, as well as for chemical-shift selective lipid suppression. High temporal stability of the main magnetic field is yet another of the stringent requirements, which is of particular importance for functional MRI, MR spectroscopy, and, of course, perfusion imaging.
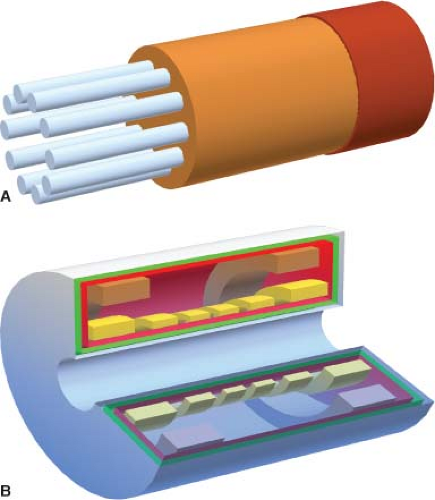
The only technology available that is capable of creating magnetic fields of sufficient strength and stability over a volume sufficient to fit an entire human body is based on superconducting electromagnets. Several materials, when cooled below a critical temperature, typically about several Kelvin above absolute zero, exhibit a phenomenon of superconductivity, where their electrical resistance reduces exactly to zero. Alloys of several metals, among them the most commonly used is the alloy of niobium and titanium (NbTi), have an additional useful property of being able to maintain superconductivity in strong magnetic fields (type II superconductors). Most modern MRI magnets are wound of the NbTi wire (Fig. 4.4). Unfortunately, this involves a need to handle cryogenic temperatures required to maintain the main magnet windings in the superconducting state. The cryostat of the magnet consists of a liquid helium bath,
large enough to host the main magnet coils, surrounded by several thermal shields. Cryostats of the modern MRI systems contain only one cryogenic agent, liquid helium, typically at atmospheric pressure, maintaining a temperature of 4.2 K. The external thermal shield (so-called radiation shield) is cooled with a cold helium gas. The loss of liquid helium is compensated for by a cryocooler (also known as a “cold head”), which recondenses helium and produces the well-known squeaky pump-like sound in the magnet room. To date, many of the MRI magnets are rated as “zero boil-off” magnets, which means that no helium is lost at least when no scanning is performed.*
large enough to host the main magnet coils, surrounded by several thermal shields. Cryostats of the modern MRI systems contain only one cryogenic agent, liquid helium, typically at atmospheric pressure, maintaining a temperature of 4.2 K. The external thermal shield (so-called radiation shield) is cooled with a cold helium gas. The loss of liquid helium is compensated for by a cryocooler (also known as a “cold head”), which recondenses helium and produces the well-known squeaky pump-like sound in the magnet room. To date, many of the MRI magnets are rated as “zero boil-off” magnets, which means that no helium is lost at least when no scanning is performed.*
It is important to also consider the field outside the magnet because the stray field of a high-field MRI magnet can range very far out. This causes significant safety concerns and results in siting difficulties in the hospitals; for example, patients with pacemakers are not allowed to be exposed to fields stronger than 5 Gauss (0.5 mT). Additionally, motion of large ferromagnetic objects (e.g., elevators, cars parked outside of the building) within the stray field of the magnet will affect the homogeneity and stability of the field and may result in artifacts. Therefore, most of the MRI magnets these days are shielded either passively (by enclosing the magnet into a thick-walled iron room) or actively.5 Active shielding (available up to field strength of 4T and now also 7 T) is realized by introducing additional windings outside of the main magnet bobbins set to cancel or reduce the field outside of the bore. Of course, this also reduces the field inside the bore; consequently, shielded magnets are considerably larger and heavier and use more wire than their unshielded counterparts. Nonetheless, the additional effort needed for active shielding turns out to be very beneficial when improved stability and reduced siting costs are considered. Moreover, active shielding reduces the overall weight of the system if the weight of the thick iron cage for passive shielding of unshielded magnets is considered.
Magnetic Field Gradients
To realize the spatial encoding in MRI, one needs a means of inducing spatially inhomogeneous changes of Larmor frequency. The most intuitive and straightforward spatial encoding approach in MRI is based on using linear field variations, so-called magnetic field gradients. A three-dimensional (3D) imaging system has to be equipped with specialized coils, each of them capable of inducing approximately linear Larmor frequency variation along each of the three principal spatial directions and a corresponding driving system to switch the gradient fields on and off or modulate their strength over time. Do note that by energizing more than one of these physical gradient coils simultaneously, gradient fields in arbitrary directions can be achieved.
Strictly speaking, despite their name, magnetic field gradients are designed to create a linear variation of the Larmor frequency rather than the magnetic field itself. It is important to note that the magnetic field is a vector quantity characterized by a direction and strength at every point in space, whereas Larmor frequency is a scalar, proportional to the magnitude of the local magnetic field. It is important to keep in mind that the local magnetic field at every point in space, upon the action of the gradients, turns out to be a vector sum of a strong and homogeneous main magnetic field B0, typically oriented along the z-axis, and a spatially dependent magnetic field induced by a gradient coil, BG. Note that in typical MRI settings, the magnitude of the main magnetic field |B0| is by several orders of magnitude larger than the field induced by the gradient coil |BG| (compare 1.5 ÷ 3T vs. 20 ÷ 50 mT/m). Figure 4.5 visualizes several cases of a vector field addition, where it becomes apparent that primarily the component of BG that is collinear to B0 is able to change the magnitude of the resulting vector, whereas the effect of the component orthogonal to B0 has a much smaller effect and can typically be ignored. Therefore, for high-field MRI the objective of creating a linear Larmor frequency dispersion is equivalent to the requirement of producing a magnetic field BG with the z-component, BGz, having a linear spatial dependency. It is noteworthy that the transverse components of BG, which are often termed “Maxwell fields” or “concomitant fields,”6 still induce minor frequency offsets, which can be ignored in conventional imaging but become important in applications using very high gradient amplitudes, such as diffusion or flow imaging,7 or at lower magnetic field strengths.
The linear spatial dependency of a scalar field in 3D (e.g., resonance frequency or magnetic field magnitude) can be characterized with a single vector, called a gradient, whose magnitude corresponds to the rate of change of the scalar parameter per unit length and whose direction points toward its steepest increase. MRI systems are equipped with specialized coils capable of creating such gradients in the three principal spatial directions. As already mentioned, superposition of the three fields allows one to generate a field gradient of an arbitrary orientation.
The gradient coil of commercial MRI systems with a conventional geometry is a cylindrical structure with a relatively thick wall of about 5 to 8 cm, which occupies the space between the warm bore of the magnet (i.e., bore size defined by the outer wall of the magnet’s cryostat) and the patient-accessible bore. It includes highly optimized copper winding patterns targeted at creating the desired gradient fields while minimizing the switching
time (reducing inductance) and energy dissipation (keeping resistance of the windings low).8 Modern gradient coils are equipped with a water cooling system to transport the generated heat from the coil. Commercial MRI systems are driven by high-power gradient power amplifiers, capable of generating currents of about 500 to 800 A and voltages of 2 kV and above. High currents are required to achieve high gradient amplitudes, whereas high voltages are needed to enable fast switching (i.e., short rise times). Up-to-date MRI scanners are typically equipped with gradients reaching 40 to 70 mT/m per channel with a switching time of about 200 μs. Another important characteristic of the gradient coil is the size of the homogeneous gradient region, which alternatively can be specified as a maximum gradient deviation over the target imaging volume. Do note that the amount of energy needed drive a gradient coil is proportional to the fifth power of its radius. Thus, scaling up a coil by 15% will approximately double its power requirements.
time (reducing inductance) and energy dissipation (keeping resistance of the windings low).8 Modern gradient coils are equipped with a water cooling system to transport the generated heat from the coil. Commercial MRI systems are driven by high-power gradient power amplifiers, capable of generating currents of about 500 to 800 A and voltages of 2 kV and above. High currents are required to achieve high gradient amplitudes, whereas high voltages are needed to enable fast switching (i.e., short rise times). Up-to-date MRI scanners are typically equipped with gradients reaching 40 to 70 mT/m per channel with a switching time of about 200 μs. Another important characteristic of the gradient coil is the size of the homogeneous gradient region, which alternatively can be specified as a maximum gradient deviation over the target imaging volume. Do note that the amount of energy needed drive a gradient coil is proportional to the fifth power of its radius. Thus, scaling up a coil by 15% will approximately double its power requirements.
To understand how gradient fields may be created, it is instructive to consider simplified topologies based on simple current loops. The easiest to visualize is the basic design of the z-gradient, which consists of two loop coils with the current flowing in an opposite direction separated by a certain distance (Fig. 4.6A). This coil arrangement is capable of generating a magnetic field with the approximately linearly varying BGz component within a region close to the center of the coil. The most simple coil for generating a transverse gradient field (along the x- or y-axis) requires at least four current loops (Fig. 4.6B, C). Only the central arch segments of these coils are important for generating the corresponding gradient; other segments are required to feed the gradient-generating segments and to close the current loop and are termed feeding or return current paths.
Gradient coils as described earlier not only produce magnetic field gradients inside the bore but also create significant fields outside. When gradients are switched, these fields induce eddy currents in the cold conductive structures of the magnet (i.e., aluminum walls of the cryostat, thermal shields, etc.). This may have two adverse effects: on one hand, eddy currents inside the magnet may cause heating and vibrations, which increases helium boil-off and may eventually cause the magnet to quench. on the other hand, eddy currents in the cold metal have very long decay times (100 ms up to several seconds) and induce secondary undesired magnetic fields, which distort the spatial encoding or affect the magnetization evolution and result in image artifacts.9,10 Gradient coils with active shielding11 (not to be confused with active magnet shielding mentioned before) solve this problem by introducing additional windings close to the outer cylindrical surface of the coil that are designed to cancel the fields created by the primary windings outside the coil. Availability of actively shielded gradients in commercial MRI scanners made it possible for such fast imaging sequences as echo-planar imaging (EPI) or balanced steady-state free precession (bSSFP) to enter into clinical practice.
Radiofrequency Transmit and Receive Coils
As mentioned previously, nuclear magnetization can be manipulated (e.g., excited or refocused) using RF fields tuned to the Larmor frequency. These fields are produced by specialized transmit antennas, traditionally termed transmit RF coils,12 driven by powerful RF amplifiers (in the range of tens of kilowatts for whole-body systems). Precessing magnetization produces weak magnetic fields oscillating at Larmor frequency, which can be converted back to electrical signals by a corresponding receive coil. These weak signals, on the order of tens of μV (i.e., in the nanowatt range), are amplified by a specialized low-noise amplifier and converted thereafter to a digital representation by an analog-to-digital converter (ADC). Nowadays preamplifiers are placed as close as possible to the RF coil as electrons in copper wires undergo thermal motion and give rise noise. To minimize this noise cable lengths are kept as short as possible
before the first amplification stage. It is possible to share the same coil for both transmission and reception using a so-called T/R switch, which protects the preamplifier during RF transmission; such coils are referred to as transmit-receive (Tx/Rx) coils. There are also transmit-only and receive-only coils, which are then used in various combinations in a so-called cross-coil configuration.
before the first amplification stage. It is possible to share the same coil for both transmission and reception using a so-called T/R switch, which protects the preamplifier during RF transmission; such coils are referred to as transmit-receive (Tx/Rx) coils. There are also transmit-only and receive-only coils, which are then used in various combinations in a so-called cross-coil configuration.
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree