Oxygen Extraction Fraction (OEF) Imaging

Weili Lin

Hongyu An

Thomas Christen

Energy in most of the human organs is provided through oxidative metabolism. O₂ is bound to the hemoglobin molecule, carried by the red blood cells in arteries, and then transferred to the mitochondria by molecular diffusion between capillaries and cells. Because the storage capacity of oxygen in tissues is limited, a tight coupling exists between blood flow and the tissue oxygen extraction fraction (OEF) to ensure a constant level of oxygen metabolism. This balance between supply and demand, however, is challenged in numerous pathologies including cancer, stroke, and neurodegenerative diseases. For instance, a reduction of cerebral blood flow (CBF) under ischemic conditions leads to an increase in the OEF to protect neuronal function. Yet, the tissue will stay viable only if the OEF doesn’t reach the maximal available capacity. In the opposite case, a cascade of cellular events could lead to possible cell death.

Although there is considerable interest in acquiring oxygenation information in complement to perfusion measurements, a gold standard method for imaging the OEF is still missing. Positron emission tomography (PET) imaging using oxygen-15 provides quantitative mapping of oxygenation but is not widely available, and the ionizing radiation limits its repeated use in the same patient. Polarographic or fluorescence quenching electrodes are highly invasive, and the optical methods such as near-infrared spectroscopy (NIRS) offer limited coverage and poor spatial resolution. Magnetic resonance imaging (MRI) would be a good candidate for oxygenation imaging. The technique is noninvasive and offers good spatial and temporal resolution. More important, oxygenation information could be directly related to perfusion measurements obtained with arterial spin labeling or perfusion-weighted imaging. This would offer a global and comprehensive view of the lesion environment in one single exam.

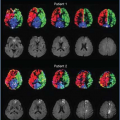
It has been widely demonstrated that when the concentration of deoxyhemoglobin is increased, a shortening of T₂ and T₂^* is anticipated, resulting in a decrease of MR signal intensity in both T₂– and T₂^*-weighted images and vice versa. This phenomenon is widely known as the blood oxygen level–dependent (BOLD) contrast.¹^,²^,³^,⁴ In addition to delineating brain functional activation, BOLD contrast has also been evaluated for its utility in discerning pathophysiologic underpinnings of various brain disorders with impaired cerebral oxygen metabolism. In particular, using the BOLD effect for monitoring changes of blood oxygenation in vivo under pathophysiologic conditions, such as hypoxia,⁵^,⁶^,⁷^,⁸^,⁹^,¹⁰^,¹¹ hyper- and hypocapnia,⁷^,¹²^,¹³ hemodilution,¹⁴ and ischemia,¹⁵^,¹⁶ has been reported by many groups. Results from these studies implicated that MR BOLD contrast could provide insights into alterations of blood oxygenation, but most of the reported results have largely focused on relative measurements and few offered quantitative aspects of BOLD effects. Recently, extensive efforts have been devoted to developing approaches for obtaining quantitative measures of BOLD effects including experimental¹⁷^,¹⁸^,¹⁹^,²⁰^,²¹^,²² and theoretical²³^,²⁴^,²⁵^,²⁶^,²⁷^,²⁸^,²⁹^,³⁰^,³¹ methods. In the context of this chapter, discussion will be concentrated on approaches capable of measuring the cerebral OEF, including the underlying theoretical frameworks, sequences, validations, and potential applications.

Global Oxygen Extraction Fraction Measurements: T₂ and Phase-Based Approaches

Thulborn et al.¹⁸ first reported a linear relation between R₂ (i.e., 1/T₂) and the square of the fraction of deoxyhemoglobin ex vivo in 1982. Subsequently, Wright et al.¹⁹ proposed a simplified signal model characterizing the relation between R₂ and blood oxygenation. A calibration curve was obtained by imaging blood samples with different oxygenation levels, which in turn provided a means to convert in vivo blood T₂ into oxygenation. This model has been extensively described and applied to the human brain,³²^,³³^,³⁴ but it is limited to the study of large vessels only because of the difficulties in isolating pure blood signal. Recently, Qin et al.³⁵ used the same principle and rapid data acquisition (<1 minute) to obtain quantitative measures of OEF in normal subjects by simultaneously measuring blood oxygenation in the carotid artery and the internal jugular vein. Lu et al.³⁶ proposed a different scheme called T₂-relaxation under spin tagging (TRUST) MRI to measure blood oxygen saturation in large veins. TRUST uses pulsed arterial spin labeling (pASL) MRI theory, but instead of arteries it labels blood on the venous side of the circulation. Images are acquired at multiple echo times (TE) and T₂ measured in a large vein was used to estimate global OEF. While these approaches are promising, a calibration curve is still needed and depends on the experimental protocol.

Alternatively to T₂-based approaches, one can leverage the phase difference (Δφ) between blood and surrounding brain parenchyma in a flow-compensated image (to avoid additional phase accrual from moving spins) to measure blood oxygenation.³⁷ Considering the blood
vessels as long paramagnetic cylinders, an exact theoretical expression can be derived to quantify the OEF:

where Δχ_do = 0.27 ppm (in centimeter-gram-second [CGS] units) is the susceptibility difference between fully deoxygenated and fully oxygenated erythrocytes, θ is the angle of the cylinder relative to the applied main magnetic field B₀, TE is echo time, and Hct is the local hematocrit fraction. Fernandez-Seara et al.³⁸ used this method in five volunteers and found an OEF of 34% ± 8% in the internal jugular vein and a decrease in oxygen saturation during breath hold, whereas Jain et al.³⁹ reported a global brain OEF of 36% ± 4%. This technique includes self-calibration, has equal sensitivity to all oxygenation levels, and is relatively straightforward to implement. Possible errors resulting from vessel tilt, noncircularity of vessel cross-section, and induced magnetic field gradients have been evaluated and corrections have been implemented.⁴⁰^,⁴¹

One of the common emphases of the aforementioned approaches is to offer an approach to measure oxygenation in large vessels as opposed to regional brain tissues. As a result, only a global measure of OEF is available and its potential clinical application is limited to disorders where global alterations are anticipated. Although recent technical developments, such as velocity selective pulses⁴²^,⁴³ (for T₂-based approaches) and quantitative magnetic susceptibility mapping⁴⁴ (for phase-based approaches), might provide a way of mapping oxygenation on a per voxel basis, different theoretical frameworks that are capable of providing oxygenation measures at the tissue level have been proposed to allow discerning focal pathology. They mainly focus on information carried through T₂^* dephasing and are the subject of the next sections.

Regional Blood Oxygenation Mapping using a Biophysical Model

Yablonskiy and Haacke²⁵ proposed in 1994 a theoretical framework of spin dephasing in a vascular network, which has been shown to be promising in measuring regional tissue oxygenation. The biophysical model considers a virtual voxel modeled as being composed of two compartments: (a) A vascular compartment is represented as an ensemble of long cylinders with negligible wall thickness and small volume fraction. The cylinders are homogeneously distributed and randomly oriented. (b) The second compartment is the extravascular compartment, which is homogeneous and surrounds the vessels. An important assumption of the model is that the diffusion distance of water molecules (Brownian motion) is small compared to the extent of magnetic field inhomogeneities that are created by deoxyhemoglobin molecules. This is favored by high magnetic field strengths and large differences in magnetic susceptibilities between the blood and tissue compartments. In such cases, the system is considered to be in the static regime, and it is possible to derive a simple equation of the evolution of the MR signal. Two important conclusions have been extracted from this model. First, the MR signal in the static regime does not depend on the distribution of vessel radius. This means that the model can be applied throughout the brain, regardless of the vessel size distribution. Second, instead of a pure mono-exponential decay, the signal evolution versus time has two distinct patterns: (a) during the first few milliseconds after spin excitation, the signal has a quadratic exponential decay; (b) then the MR signal decays mono-exponentially as

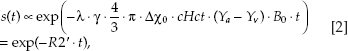
where λ is the fractional blood volume containing deoxyhemoglobin; γ is the gyromagnetic ratio; cHct is the fractional cerebral hematocrit; B₀ is the main magnetic field strength; Y_a and Y_v are the arterial and venous blood oxygenation, respectively; and Δχ₀ is the susceptibility difference between fully oxygenated and deoxygenated blood. By incorporating all constants into a constant c and assuming that arterial blood is fully saturated, this equation can be further simplified as

where c consists of 3/4π,γ, cHct, B₀, and Δχ₀; νCBV (λ) reflects fractional venous cerebral blood volume; and OEF = 1 − Y_v. In other words, if R₂′ and vCBV can be experimentally obtained, it becomes feasible to quantitatively measure in vivo OEF by means of MRI.

Do note, however, that in vivo there are two other main sources of magnetic inhomogeneities that also affect the MR signal evolution within a voxel. Specifically, (a) microscopic nuclear electron interactions between neighbor atoms give rise to the transverse relaxation mechanism described by an exponential decay. (b) Macroscopic field inhomogeneities, which may originate from magnet imperfections, poor shimming, tissue–air interfaces, and so forth affect signal evolution according to

where b_macro describes the smooth variations of magnetic field within the voxel, γ = 2.67502 * 108 rad/s/T is the nuclear gyromagnetic ratio, and V is the voxel volume. Both of these effects are potential confounds for measuring the OEF by means of MRI.

Three Different Approaches

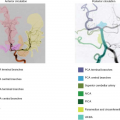
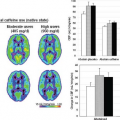
The first validation of the use of the mathematical model to obtain quantitative information about a network was shown by Yablonskiy⁴⁵ in a phantom containing polyethylene tubes. A gradient-echo sampling of the spin-echo (GESSE) sequence was used to acquire the MR signal formation at both early and long times of spin dephasing. This information was used to estimate the magnetic susceptibility and volume fraction occupied by the tubes with good accuracy. In vivo, three different approaches have been proposed to derive hemodynamic parameters such as the OEF or the cerebral blood volume (CBV). An and Lin⁴⁶^,⁴⁷ used a GESSE based approach to measure OEF and vCBV in normal subjects. In a later work by He and Yablonskiy,⁴⁸^,⁴⁹ this analytical model was refined to take into account the intravascular contribution to the signal, different tissue compartments (with different T₂s), and macroscopic magnetic field inhomogeneity. It is then directly fitted to the signal with a least-squares optimization. An attractive feature of this approach (called quantitative BOLD or qBOLD) is the determination of multiple parameters (eight in total, including vCBV and OEF) with a single noninvasive experiment. Yet, numerical studies and phantom experiments²⁷^,⁵⁰ have shown that reliable estimates are achieved only with a very high signal-to-noise ratio (SNR). Particularly, the distinction between vCBV and OEF effects can be critical. To address this issue, Christen et al.²²^,⁴⁶^,⁵¹ proposed a multiparametric qBOLD approach where certain parameters of the model are estimated from independent experiments: (a) the cerebral blood volume from measurement with a contrast agent; (b) B₀ using MR phase signal analysis; and (c) tissue T₂ from a standard multiple spin-echo technique. The analysis focuses on the late section of the free induction decay (FID) (acquired with a multiple gradient-echo sequence) to simplify the model function. This approach allows an increase of the spatial resolution and improves the accuracy of the oxygenation estimates compared to the original quantitative BOLD approach. The use of a contrast agent for CBV estimates complicates the experimental protocol, however. This approach also uses the total cerebral blood volume (tCBV), which leads to oxygenation measurements that correspond quantitatively to the oxygen saturation averaged across the arteriovenous networks within the voxel (MR_SO₂).³¹ The third approach, proposed by An and Lin, uses an asymmetric spin-echo sequence and asymptotic behaviors of the model.⁵² This method will be described in detail in the following section.

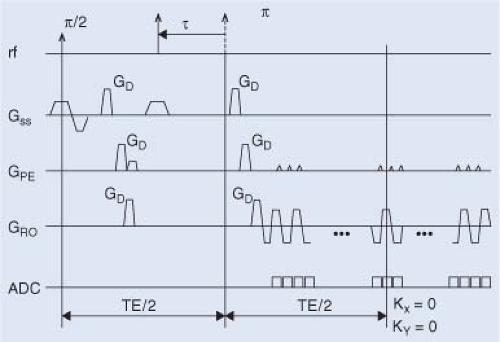FIGURE 14.1. Sequence diagram for the asymmetric spin-echo sequence. A series of images are acquired by varying τ while keeping the echo time (TE) constant, allowing estimates of venous cerebral blood volume (vCBV) and oxygenation extraction fraction (OEF).

Only gold members can continue reading. Log In or Register to continue