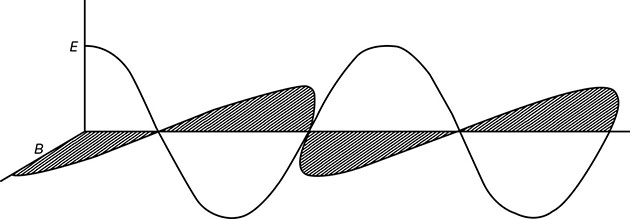
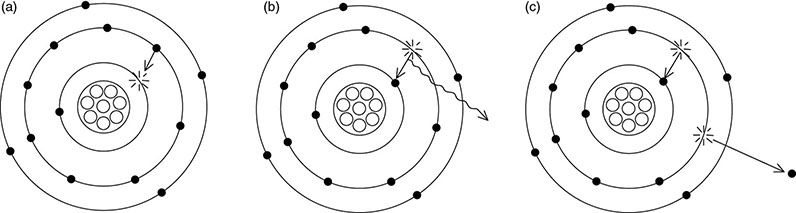
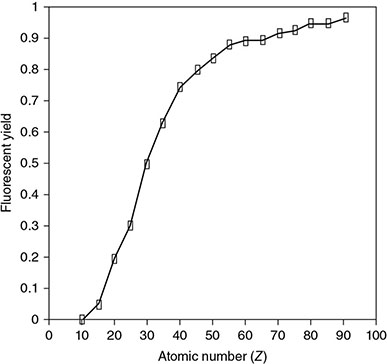
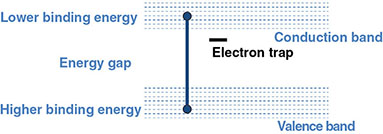
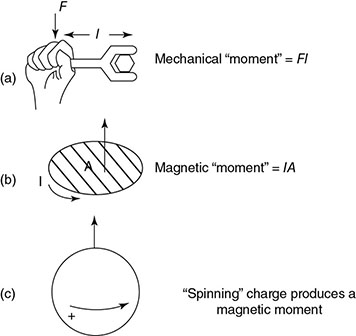
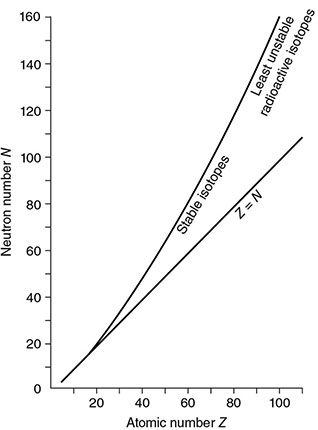
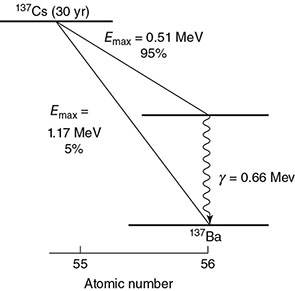
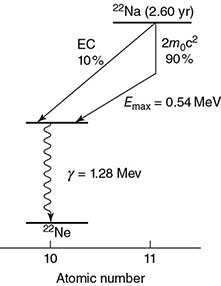
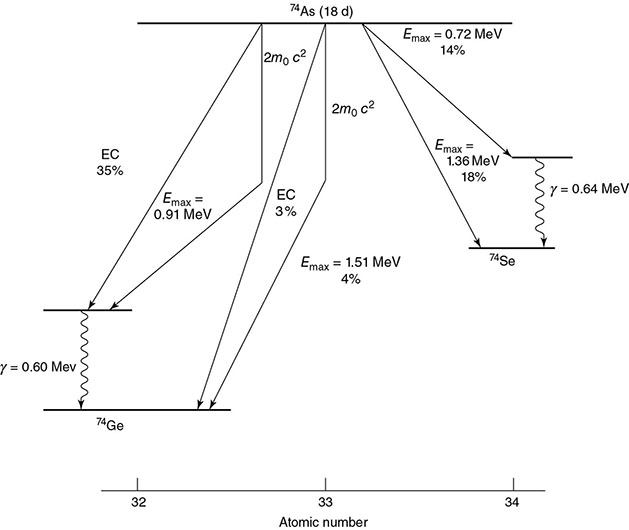
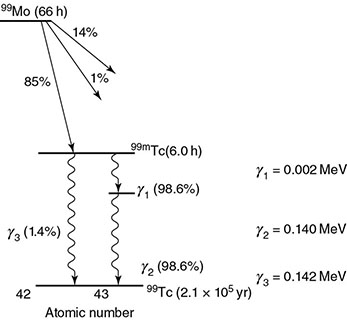
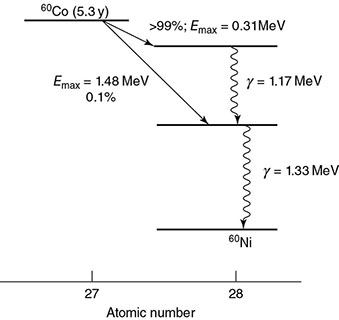
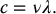1 Medical imaging at its core relies on foundational physics of electromagnetic radiation, atomic structure of stable and radioactive matter, and the interaction of radiation with matter. Associated with these topics are the process of X-ray formation and detection. Most medical imaging technologies exploit the principles associated with these foundational physics to form the medical image. These principles also impose fundamental limitations of what is possible; nature never meets our expectations for the ideal. This necessitates innovation to find technological solutions to mitigate fundamental challenges. Most advances in imaging technology have in fact been founded on innovations that best address these fundamental limitations. Therefore, insights into medical imaging and its progress necessitate an understanding of the physics of radiation and matter. Thus, it is most appropriate that we first address the foundational physics of radiation and matter in this chapter followed in Chapter 2 by a foundation of human anatomy and physiology. Electromagnetic radiation consists of oscillating electric and magnetic fields. An electromagnetic wave requires no medium for propagation; that is, it can travel in a vacuum as well as through matter. In the simplified diagram in Figure 1.1, the wavelength of an electromagnetic wave is depicted as the distance between the adjacent crests of the oscillating fields. The wave is moving from left to right in the diagram. Figure 1.1 Simplified diagram of an electromagnetic wave. The constant speed c of electromagnetic radiation in a vacuum is the product of the frequency ν and the wavelength λ of the electromagnetic wave, Often it is convenient to assign wavelike properties to electromagnetic energy. At other times, it is useful to regard these radiations as discrete bundles of energy-termed photons or quanta. The two interpretations of electromagnetic radiation are united by the equation where E represents the energy of a photon and ν represents the frequency of the electromagnetic wave. The symbol h represents Planck’s constant, 6.62 × 10−34 J s. From Eq. (1.1), and the photon energy may therefore be written as Using this equation, the energy in units of kiloelectron volts (keV) of a photon of wavelength λ in nanometers (nm) may be computed by Electromagnetic waves ranging in energy from a few nanoelectron volts up to the order of a gigaelectron volt make up the electromagnetic spectrum in Table 1.1 (definition of electron volt can be found in Section 1.2.2.3). Table 1.1 Generally accepted ranges for the electromagnetic spectrum Note that the range of values for many designations overlap and are not universally defined. All matter is composed of atoms. A sample of a pure element is composed of a single type of atom, and chemical compounds are composed of more than one type of atom. Atoms themselves are complicated entities with detailed internal structure, but they are the smallest unit of matter that retains the chemical properties of a material. In that sense, an atom is a fundamental building block of matter. In the case of a compound, the fundamental building block is a molecule consisting of one or more atoms bound together by electrostatic attraction and/or the sharing of electrons by more than one nucleus. In its simplest depiction, the Bohr model of the atom is a positively charged nucleus, containing electrically neutral neutrons and positively charged protons, surrounded by one or more negatively charged electrons. The number and distribution of electrons in the atom determine the chemical properties of the atom, and the number and configuration of neutrons and protons (collectively, nucleons) in the nucleus determine the stability of the atom in its nuclear configuration. The positive charge and roughly half the mass of the nucleus are contributed by protons. Each proton possesses a positive charge of +1.6 × 10−19 C, equal in magnitude but opposite in sign to the charge of an electron. The number of protons (or positive charges) in the nucleus is the atomic number of the atom, designated as Z. The number of neutrons in a nucleus is the neutron number N for the nucleus. The mass number A of the nucleus is the number of nucleons in the nucleus, i.e. A = Z + N. The mass of a proton is 1.6734 × 10−27 kg, and neutrons have a mass of 1.6747 × 10−27 kg. Clearly, expressing the mass of atomic particles in kilograms is unwieldy, so the atomic mass unit (amu) is a more convenient unit for atomic particles. An amu is defined as 1/12 the mass of the carbon atom, which has six protons, six neutrons, and six electrons. Therefore, The standard form used to denote the composition of a specific atom or nucleus is Isotopes of a particular element are atoms that possess the same number of protons but a varying number of neutrons. For example, 1H(protium), 2H(deuterium), and 3H(tritium) are isotopes of the element hydrogen and Table 1.2 Nucleus variability based on the numbers of protons and neutrons Atoms in their normal state are neutral because the number of electrons outside the nucleus (i.e. the negative charge in the atom) equals the number of protons (i.e. the positive charge in the atom) of the nucleus. Electrons are positioned in energy levels, termed shells that surround the nucleus. The first or K-shell contains no more than two electrons, the second or L shell contains no more than eight electrons, and the third or M shell contains no more than 18 electrons (see Figure 1.2). The outermost electron shell of an atom, no matter which shell it is, never contains more than eight electrons. Electrons in this shell are called valence electrons and determine, to a large degree, the chemical properties of the atom. For example, atoms with an outer shell entirely filled with electrons seldom react chemically; these atoms constitute inert elements known as the noble gases (i.e. helium, neon, argon, krypton, xenon, and radon). Figure 1.2 Electron configuration showing electron shells in the Bohr model of the atom for potassium, with 19 electrons and 19 protons (Z = 19). The energy levels for electrons are divided into sublevels separated from each other. To describe the position of an electron in the extranuclear structure of an atom, the electron is assigned four quantum numbers designated below as n, l, ml, and ms. The principal quantum number, n, defines the main energy level or shell within which the electron resides (n = 1 for the K-shell, n = 2 for the L shell, etc.). The orbital angular momentum (also called azimuthal) quantum number, l, describes the electron’s angular momentum (l = 0, 1, 2, … n − 1). The orientation of the electron’s magnetic moment in a magnetic field is defined by the magnetic quantum number, ml (ml = −l, −l + 1, … l − 1, l). The direction of the electron’s spin upon its own axis is specified by the spin quantum number, ms (ms = +½ or −½). The Pauli exclusion principle states that no two electrons in the same atomic system may be assigned identical values for all four quantum numbers. Listed in Table 1.3 are quantum numbers for electrons in a few atoms with low atomic numbers. Table 1.3 Quantum numbers for electrons in helium, carbon, and sodium The values of the orbital angular momentum quantum number, l = 0, 1, 2, 3, 4, 5, and 6, are also identified with the symbols, s, p, d, f, g, h, and i, respectively. This notation is known as “spectroscopic” because it is used to describe the separate emission lines observed when light emitted from a heated metallic vapor lamp is passed through a prism. From 1890s onward, observation of these spectra provided major clues about the binding energies of electrons in atoms of the metals under study. By the 1920s, it was known that the spectral lines above “s” (i.e. l = 0) could be split into multiple lines in the presence of a magnetic field. The lines were thought to correspond to “orbitals” or groupings of similar electrons within orbits. The modern view of this phenomenon is that while the “s” orbital is spherically symmetric (see Figure 1.3), the other orbitals are not. In the presence of a magnetic field, the “p” orbital can be in alignment along any one of the three axes of space: x, y, or z. Each of these three orientations has a slightly different energy corresponding to the three possible values of ml (−1, 0, and 1). According to the Pauli exclusion principle, each orbital may contain two electrons, one with ms = +½ and the other with ms = –½. Figure 1.3 Possible location of the electron in the hydrogen atom for three different energy states or combinations of principal and angular momentum quantum numbers (n, l, m). Brighter shading corresponds to higher probability locations. From left to right: (2,0,0), (2,1,0), (2,1,1), (3,2,1). The K-shell of an atom consists of one orbital, 1s, containing two electrons. The L shell consists of the 2s subshell that contains one orbital (two electrons) and the 2p subshell containing a maximum of three orbitals (i.e. six electrons). If the L shell of an atom is filled, its electrons will be noted in spectroscopic notation as 2s22p6. This notation is summarized for three atoms: helium, carbon, and sodium, in Table 1.3. Since the late 1920s, it has been understood that electrons in an atom do not behave exactly like tiny moons orbiting a planet-like nucleus. It is more accurate to define them as entities whose behavior is described by wave functions, rather than as point particles in orbits. Although a wave function itself is not directly observable, calculations performed with this function predict the location of the electron. In contrast to the calculations of classical mechanics, in which properties such as force, mass, and acceleration are used in equations to yield a definite answer for a quantity such as position in space, quantum mechanical calculations yield probabilities. At a particular location in space, for example, the square of the amplitude of a particle’s wave function yields the probability that the particle will appear at that location. In Figure 1.3, this probability is depicted for several possible energy levels of a single electron surrounding a hydrogen nucleus (i.e. a single proton). In this illustration, a brighter shading implies a higher probability of finding the electron at that location. Locations at which the probability is maximized correspond roughly to the electron shell model discussed previously. However, it is important to emphasize that the probability of finding the electron at other locations, even in the middle of the nucleus, is not zero. This particular result explains a certain form of radioactive decay in which a nucleus captures an electron, a phenomenon that is better explainable by quantum mechanics. The extent to which electrons are bound to the nucleus determines several energy absorption and emission phenomena. The binding energy of an electron (Eb) is defined as the energy required to completely remove the electron from the atom. When energy is measured in the macroscopic world of everyday experience, units such as joules (J) and kilowatt hours (kWh) are used. In the microscopic world, the electron volt (eV) is a more convenient unit of energy. One electron volt is the kinetic energy imparted to an electron accelerated across a potential difference (i.e. voltage) of 1 V. One eV is equal to 1.6 × 10−19 J or 4.4 × 10−26 kWh. As a unit of energy, the eV describes potential energy as well as kinetic energy; the binding energy of an electron in an atom, for example, is a form of potential energy. An electron in an inner shell of an atom is attracted to the nucleus by a force greater than that exerted by the nucleus on an electron farther away. An electron may be moved from one shell to a more distant shell only if energy is supplied by an external source. Binding energy is a negative value because it represents an amount of energy that must be supplied to remove an electron from an atom. The energy that must be imparted to an atom to move an electron from an inner shell to an outer shell is equal to the arithmetic difference in binding energy between the two shells. For example, the binding energy for an electron in the K-shell of hydrogen is −13.5 and −3.4 eV for an electron in the L shell. The energy required to move an electron from the K to the L shell in hydrogen is (−3.4 eV) − (−13.5 eV) = 10.1 eV. Electrons in inner shells of high-Z atoms are bound to the nucleus with a force much greater than that exerted upon the solitary electron in hydrogen (Table 1.4). Table 1.4 Average binding energies (in eV) for electrons in hydrogen (Z = 1) and tungsten (Z = 74) The electrons within a particular electron shell do not have exactly the same binding energy. Differences in binding energy among the electrons in a particular shell are determined by the orbital magnetic and spin quantum numbers, l, ml, and ms. The combinations of these quantum numbers provide three subshells for the L shell (LI to LIII) and five subshells for the M shell (MI–MV), where the M subshells occur only if a magnetic field is present. Energy differences between the subshells are small when compared to differences between shells, but these differences are important in medical imaging as they explain certain properties of the emission spectra of X-ray tubes. Table 1.5 gives values for the binding energies of K, L, and M shell electrons for selected elements. Table 1.5 Binding energies of electron shells of selected elements Source: Data from Fine [1]. Various processes can cause an electron to be ejected from an electron shell. When an electron is removed from a shell a vacancy or hole is left in the shell (i.e. a unique quantum “address” is left vacant). An electron may move from another shell to fill the vacancy. This movement, called an electron transition, involves a change in the binding energy of the moving electron. To move an inner shell electron to an outer shell, some external source of energy is required. Alternatively, an outer shell electron may drop spontaneously to fill a vacancy in an inner shell. This spontaneous transition results in the release of energy. Spontaneous transitions of outer shell electrons falling to inner shells are depicted in Figure 1.4. Figure 1.4 (a) Electron transition from an outer shell to an inner shell. (b) Electron transition accompanied by the release of a characteristic photon. (c) Electron transition accompanied by the emission of an Auger electron. The energy released when an outer electron falls to an inner shell equals the difference in binding energy between the two shells involved in the transition, for example, an electron moving from the M to the K-shell of tungsten releases (−69 500 eV) − (−2810 eV) = −66 690 eV or −66.69 keV. The energy is released in one of the two forms. In its first form, the transition energy is released as a photon (see Figure 1.4b). As the binding energy of electron shells is a unique characteristic of each element, the emitted photon is called a characteristic photon or characteristic X-ray. The emitted photon may be described as a K, L, or M characteristic photon denoting the destination of the transition electron. An electron transition creates a vacancy in the outer shell where the electron originated, and this vacancy may be filled by an electron transition from another shell, leaving yet another vacancy and so on. Thus, a vacancy in an inner electron shell produces a cascade of electron transitions that yield a range of characteristic photon energies. Electron shells farther from the nucleus are more closely spaced in terms of binding energy. Therefore, characteristic photons produced by transitions among outer shells have less energy than do those produced by transitions involving inner shells. For transitions to shells beyond the M shell, characteristic photons are no longer energetic enough to be considered X-rays. An alternative to the release of the energy through characteristic X-ray production is for the atom to “eject” another electron to compensate for an electron vacancy (Figure 1.4c). In this process, the energy released during an electron transition is transferred to another electron. This energy is sufficient to eject the electron from its shell. The ejected electron is referred to as an Auger electron. The kinetic energy of the ejected electron will not equal the total energy released during the transition because some of the transition energy is used to free the electron from its shell. The Auger electron is usually ejected from the same shell that held the electron that made the transition to an inner shell, as shown in Figure 1.4c. In this case, the kinetic energy of the Auger electron is calculated by twice subtracting the binding energy of the outer shell electron from the binding energy of the inner shell electron. The first subtraction yields the transition energy and the second subtraction accounts for the liberation of the ejected electron. Either characteristic photon emission or Auger electron emission may occur during an electron transition, and although it is impossible to predict which process will occur for a specific atom, the probability of characteristic emission is termed the fluorescence yield, ω, defined as The fluorescence yield increases with atomic number as depicted in Figure 1.5. For a transition to the K-shell of calcium, for example, the probability is 0.19 that a K characteristic photon will be emitted and 0.81 that an Auger electron will be emitted. The fluorescence yield is a factor to consider in the selection of materials used to produce X-ray imaging system and also for radioactive sources for nuclear imaging where Auger electrons result in increased radiation dose to the patient because they do not travel far in tissue. Figure 1.5 Fluorescence yield for the K-shell vs. atomic number. Electrons in individual atoms have specific binding energies described by quantum mechanics, but when atoms bind together into solids, the energy levels change as the electrons influence each other. Just as each atom has a unique set of quantum energy levels, a solid also has a particular set of energy levels, called energy bands, which are determined by the combination of atoms composing the solid and by bulk properties of the material such as temperature and pressure. Furthermore, similar to quantum vacancies or holes that may exist when an allowable energy state in a single atom is not filled, energy bands in a solid may or may not be fully populated with electrons. This enables the movement of charge between bands. Two electron energy bands of a solid are depicted in Figure 1.6. The lower energy band, called the valence band, consists of electrons that are tightly bound in the chemical structure of the material. The upper energy band, called the conduction band, contains electrons that are relatively loosely bound. Conduction band electrons can move in the material and may constitute an electrical current under the proper conditions. If no electrons populate the conduction band, then the material cannot support an electrical current under normal circumstances. However, if enough energy is imparted to an electron in the valence band to raise it to the conduction band, then the material can support an electrical current. Figure 1.6 Energy level diagram for solids. An electron promoted from the valence band to the conduction band may move freely in the material to constitute an electric current. Solids can be separated into three categories based on the difference in energy between electrons in the valence and conduction bands. In conductors, there is little energy difference between the bands, so the electrons are continuously promoted from the valence to the conduction band by routine collisions between electrons. In insulators, the conduction and valence bands are separated by a wide band gap, also known as the “forbidden zone,” of 3 eV or more. Under this condition, application of voltage to the material usually will not provide enough energy to promote electrons from the valence band to the conduction band. Therefore, insulators do not support an electrical current under normal circumstances. Of course, there is always a “breakdown voltage” above which an insulator will support a current, although probably not without structural damage to the material. If the band gap of the material is between 0 and 3 eV, the material exhibits electrical properties between those of an insulator and a conductor. Such a material, termed a semiconductor, will conduct electricity under certain conditions and act as an insulator under others. The conditions may be altered by the addition to the material of trace amounts of impurities that have allowable energy levels within the band gap of the solid, creating electron traps as depicted in Figure 1.6. Semiconductors have significant applications in radiation detection. As previously stated, very little energy is required to promote electrons to the conduction band in a conductor. However, the electrons in the conduction band do not fully move freely. As electrons attempt to move through the material, they interact with other electrons and with imperfections such as impurities in the solid. At each encounter, some energy is transferred to atoms and molecules and ultimately transferred to heat. This transfer of energy is the basis of electrical resistance and is usually viewed as energy loss, unless explicitly intended for heat production as in an electric heating element. Otherwise, the loss or energy and mitigation for that loss can create a significant technological challenge as in an X-ray tube. There is a class of material, called superconductors, in which there is very little resistance to the flow of electrons under certain conditions. First discovered in mercury [2], it has been described using the formalism of quantum mechanics [3,4] and is applicable to other materials at very low temperature. In a superconductor, the passage of an electron disturbs the structure of the material in such a way as to encourage the passage of another electron arriving after exactly the right interval of time. The passage of the first electron establishes an oscillation in the positive charge of the material that pulls the second electron through. This behavior has been analogized as “electronic waterskiing” where one electron is swept along in another electron’s “wake.” Thus, electrons tend to travel in what are known as Cooper pairs. Cooper pair electrons form a lower energy bound state where they can move as a unit with essentially no resistance to flow. Currents in superconducting loops of wire can continue for several years with no additional input of energy. Many types of materials exhibit superconductive behavior when cooled to temperatures in the range of a few degrees Kelvin (room temperature is approximately 295 K); in fact, 26 elements and thousands of alloys and compounds exhibit this behavior. Lowering the temperature promotes superconductivity by decreasing molecular motion, thereby decreasing the kinetic energy of the material. However, maintenance of materials at very low temperatures often requires liquid helium as a cooling agent. Helium liquefies at 23 K, is relatively expensive, and is usually insulated from ambient conditions with another refrigerant such as liquid nitrogen, which adds to the cost. Superconducting technology is a foundation of modern magnetic resonance imaging (MRI) systems, where a string magnetic field is produced and maintained by superconducting coils. The science of superconducting has been leading to higher temperature possibilities in new material (e.g. barium–lanthanum–copper oxide and magnesium diboride) [5,6], with potential profound impact across electronic technology, which is sure to have a significant influence on medical imaging technology as well. In a model analogous to that for electrons, the shell model of the nucleus considers nucleons to be distributed between shells and was introduced to explain the existence of discrete nuclear energy states. Furthermore, nuclei are extraordinarily stable if they contain 2, 8, 20, 82, or 126 protons or similar numbers of neutrons. These numbers are termed magic numbers and may reflect full occupancy of nuclear shells. Nuclei with odd numbers of neutrons or protons tend to be less stable than nuclei with even numbers of neutrons and protons. The pairing of similar nucleons increases the stability of the nucleus, as illustrated in Table 1.6. Table 1.6 Relationships between protons, neutrons, and stability Protons have “like” charges, i.e. each has the same positive charge and as such repel each other by the electrostatic force of repulsion. One may then wonder how a nucleus stays together. The answer is that when protons are very close together, there is also an attractive force. This force, called the strong nuclear force, is one hundred times stronger than the electrostatic force of repulsion. However, it acts only over distances on the order of magnitude of the diameter of the nucleus. Therefore, protons can stay together in the nucleus once they are there but assembling a nucleus by forcing protons together requires the expenditure of significant energy to overcome the electrostatic repulsion. Neutrons have no electrostatic charge and do not experience the electrostatic force. Therefore, adding neutrons to a nucleus requires much less energy. Neutrons are, however, governed by the weak nuclear force, a force through which a neutron is believed to change spontaneously into a proton, an electron, and an antineutrino (an almost massless, virtually noninteracting particle) and back (i.e. a proton to a neutron, a positron, and a neutrino). These processes, called beta decay, are described in greater detail in the Section 1.3.1. Gravity, as the fourth of the traditional four fundamental forces of nature (i.e. electromagnetism, strong nuclear force, weak nuclear force, and gravity), is so overshadowed in terms of magnitude by the other forces within an atom that it is believed to play no essential role in nuclear stability or instability. The mass of an atom is less than the sum of the masses of its neutrons, protons, and electrons. This seeming paradox reflects Einstein’s famous equation E = mc2 depicting the exchangeability of the binding energy of the nucleus and the masses of its constituent particles. When the nucleons are separate, they have their own individual masses, but when they are combined in a nucleus, some of their mass is converted into binding energy. The mass difference between the sum of the masses of the atomic constituents and the mass of the assembled atom is termed the mass defect. In Einstein’s equation, an energy E is equivalent to mass, m, multiplied by the speed of light in a vacuum, c, (i.e. 2.998 × 108 m/s) squared. Because of the significant magnitude of the proportionality constant, c2, in this equation, 1 kg of mass is equal to 9 × 1016 J, roughly equivalent to the energy released during detonation of 30 megatons of C7H5N3O6 (i.e. TNT). The energy equivalent of even 1 amu is significant: Figure 1.7 depicts the average binding energy per nucleon as a function of the mass number A. Figure 1.7 Average binding energy per nucleon vs. mass number and its exploitation in the processes of fission and fusion. The potential energy bound in the nucleus can be released if a nucleus with a high mass number separates or fissions into two parts, each with an average binding energy per nucleon greater than that of the original nucleus. The energy release occurs because this split produces low-Z products with a higher average binding energy per nucleon than the original high-Z nucleus (see Figure 1.7). A transition from a state of lower binding energy per nucleon to a state of higher binding energy per nucleon results in the release of energy. This is reminiscent of the previous discussion of energy release that accompanies an L to K electron transition. However, the energy available from transition between nuclear energy levels is orders of magnitude greater than the energy released during electron transitions. Certain high-A nuclei (e.g. 233U, 235U, and 239Pu) fission spontaneously after absorbing a slowly moving neutron. For 235U, a typical fission reaction is as follows: The energy released is designated as Q and averages more than 200 MeV per fission. The energy is liberated primarily as 235U radiation, kinetic energy of radioactive fission products (e.g. The counterpart to fission is fusion, which is the combination of certain low-mass nuclei to produce a nucleus with an average binding energy per nucleon greater than that for either of the original nuclei. This process is accompanied by the release of large amounts of energy. A typical reaction is as follows: In this particular reaction, Q = 18 MeV per fusion. To form products with higher average binding energy per nucleon, nuclei must be brought sufficiently near one another, so the strong nuclear force can initiate fusion. In the process, the electrostatic force of repulsion must be overcome as the two nuclei approach each other. Nuclei moving at very high velocities possess enough momentum to overcome this repulsive force. Adequate velocities may be attained by heating a sample containing low-Z nuclei to a temperature greater than 12 × 1060 K, roughly equivalent to the temperature in the inner region of the Sun. Fusion is in fact the primary reaction fueling the energy production in the stars including our sun. Fusion has been used for military purposes where the high temperatures necessary in initiating a fusion (e.g. in a hydrogen bomb) are triggered with a fission igniter explosion. Although still out of reach, however, there is much hope and effort for harnessing fusion for safe energy production. Protons and neutrons behave like small magnets and have associated magnetic moments. The term moment has a strict meaning in physics. When a force is applied on a wrench to turn a bolt (see Figure 1.8a), for example, the mechanical moment is the product of force and length. The mechanical moment can be increased by increasing the length of the wrench, applying more force to the wrench, or a combination of the two. A magnetic moment (see Figure 1.8b) is likewise the product of the current in a circuit (i.e. a path followed by electrical charges) and the area encompassed by the circuit, so the magnetic moment is increased by increasing the current, the area, or a combination of the two. The magnetic moment is a vector, a quantity having both magnitude and direction. Like electrons, protons have a characteristic called spin, which can be explained by treating the proton as a small object spinning on its axis. In this model, the spinning charge of the proton produces a magnetic moment (see Figure 1.8c). Figure 1.8 The concept of a “moment” in physics. (a) A mechanical moment is defined by force F and length l. (b) A magnetic moment is defined by current I and the area A enclosed by the current. (c) The magnetic moment produced by a spinning charged object. The spinning–charge model of the proton has two marked limitations. First, the mathematical prediction for the value of the magnetic moment is not quite what has been measured experimentally. From the model, a proton would have a fundamental magnetic moment known as the nuclear magneton, μn as Here, e is the charge of the proton in coulombs, ℏ is Planck’s constant divided by 2π, and Mp is the mass of the proton. The magnetic moment magneton, μp, of the proton, however, has been measured as μp = 2.79μn. This classical observation has been explained using quantum mechanics and is used to describe the fundamental concepts of MRI (see Chapter 10). The unit of the nuclear magneton, energy (i.e. electron volt) per magnetic field strength (i.e. gauss), expresses the fact that a magnetic moment has a certain potential energy in a magnetic field. The second difficulty of the spinning proton model is that the neutron, an uncharged particle, exhibits a magnetic moment equal to 1.91μn. An explanation for these limitations is that neutrons and protons are composed of other more fundamental particles called quarks [7,8]. Quarks have fractional charges adding up to a unit charge. The nonuniform distribution of spinning charges of the quarks is the basis for the observed magnetic moments. When magnetic moments exist near each other, as in the nucleus, they tend to form pairs with the vectors of the moments pointed in opposite directions. In nuclei with even numbers of neutrons and protons, this pairing cancels out the magnetic properties of the nucleus as a whole. Thus, an atom such as Table 1.7 Nuclides with a net magnetic moment Source: Data from Heath [9]. Assuming a nucleus shell model resembling the electron shell model, a neutron or proton in a high energy level may fall to fill a vacancy at a lower energy level. This transition releases energy and yields a more stable nucleus. The amount of energy released is again related to the difference in binding energy between the higher and lower levels, but the binding energy is much greater for neutrons and protons inside the nucleus than for electrons outside the nucleus. Hence, energy released during nuclear transitions is much greater than that released during electron transitions. This process is known as radioactivity, first discovered in 1896 by Henri Becquerel who observed the emission of radiation, later shown to be beta particles, from uranium salts. Becquerel experienced a skin burn from carrying a radioactive sample in his vest pocket, which is also the first known bioeffect of radiation exposure. In all radioactive transitions, the nucleus loses energy and gains stability, which is the essence of radioactive decay. In any radioactive process, the mass number of the decaying nucleus (i.e. parent) equals the sum of the mass numbers of the product nucleus (i.e. progeny) and the ejected particle. That is, mass number A is conserved in radioactive decay. The energy is released in the form of the kinetic energy of the progeny, radiation, and heat. Radioactive transitions are often depicted by decay schemes. A decay scheme depicts the decay processes specific for a nuclide, a generic term for any nuclear form, with mass–energy on the y-axis plotted against the atomic number of the nuclide on the x-axis. An example is shown in Figure 1.9 where the generic nuclide Figure 1.9 A generic radioactive decay scheme representing alpha decay (1), positron decay and electron capture (2), beta decay (3), and isometric transition (4). Any of the pathways may yield a nuclide that undergoes an isomeric transition, as the nucleus has the same number of neutrons and protons before and after the energy change and is shown as Pathway 3 and Pathway 4 in Figure 1.9. In isometric transition, the nucleus gains stability by transition of a neutron between neutron energy levels or a proton between proton energy levels. In this process, the energy is released by either an electromagnetic particle, i.e. gamma ray (γ-ray), or by an ejected electron, by internal conversion. Note that no radioactive nuclide decays solely by an isomeric transition. Isomeric transitions are always preceded by either electron capture or emission of an alpha (α) or beta (±β) particle. The specific decay scheme for a given nuclide is strongly influenced by the ratio of N to Z. In low-Z stable nuclei, the number of neutrons is about equal to the number of protons. As Z increases, the number of neutrons increases more rapidly than the number of protons in stable nuclei, as depicted in Figure 1.10. The shell model of the nucleus accounts for this finding by suggesting that at higher Z, the energy difference is slightly less between neutron levels than between proton levels. Figure 1.10 Number of neutrons (N) for stable (or least unstable) nuclei plotted as a function of the number of protons (Z). Nuclei with too many or too few neutrons to reside on or close to the line of stability depicted in Figure 1.10 are unstable and therefore undergo radioactive decay. Nuclei above and to the left of the line of stability (i.e. those with N/Z ratio too high for stability) tend to emit electrons by the process of beta (β−) decay, i.e. Pathway 3. Nuclei below and to the right of the line of stability (i.e. those with N/A ratio too low for stability) tend to undergo the competing processes of positron (β+) decay and electron capture, i.e. Pathway 2. In both cases, the total number of nucleons A remains the same in the parent and progeny nuclei. Table 1.8 offers a summary of the key attributes of various radioactive transitions, which we discuss in more detail below. Table 1.8 Summary of radioactive decay in terms of changes in A, Z, and N from parent to progeny Some heavy nuclei gain stability by the emission of an alpha (α) particle, which is two protons and two neutrons tightly bound as a nucleus of helium, A decay scheme for alpha decay is depicted in Figure 1.11. Figure 1.11 Radioactive decay scheme for α decay of 226Ra. Beta decay can be envisioned as a neutron falling to a lower energy level reserved for protons and “becoming” a proton. In this process, the Z and N of the nucleus change, and the nucleus transmutes from one element to another. The product is most stable if it contains even numbers of protons and neutrons and least stable if it contains an odd number for either [11]. Nuclei with an N/Z ratio too high for stability (see Figure 1.10) decay as where A decay scheme for this transition is shown in Figure 1.12. A beta (β−) particle with a maximum energy (Emax) of 1.17 MeV is released in 5% of all decays; in the remaining 95%, a beta (β−) particle with an Emax of 0.51 MeV is accompanied by an isomeric transition of 0.66 MeV, where either a γ-ray is emitted or an electron is ejected by internal conversion. The transition energy is 1.17 MeV for the decay of 137Cs. Figure 1.12 Radioactive decay scheme for electron decay of 137Cs. Beta (β−) decay pathways are characterized by specific maximum energies; however, most beta (β−) particles are ejected with energies lower than these maxima. The average energy of beta (β−) particles is about 1/3 Emax along a specific pathway. The energy distribution of beta (β−) particles emitted during the decay of 32P is shown in Figure 1.13. Spectra of similar shape but with different values of Emax and Emean exist for the decay pathways of every beta particle emitting radioactive nuclide. In each particular decay, the difference in energy between Emax and the specific energy of the beta (β−) particle is carried away by the antineutrino. That is, where Emax is the energy released during the beta (β−) decay process, Ek is the kinetic energy of the beta (β−) particle, and Figure 1.13 Energy spectrum for beta (β−) emission from 32P. Analogous to beta decay, positron decay or electron capture (EC) can be thought as a proton falling to a lower energy level reserved for neutrons, in which case the proton “becomes” a neutron, although conservation of mass dictates that a lower mass proton cannot truly change to a neutron. As in the case of beta rays, the progeny is most stable if it contains even numbers of protons and neutrons and least stable if it contains an odd number for either [10]. Positron decay results from the nuclear transition where In positron decay, the N/Z ratio increases; hence, positron-emitting nuclides tend to be positioned below the N/Z stability curve shown in Figure 1.10. The decay of The N/Z ratio of a nuclide may also be increased by electron capture, in which an electron is captured by the nucleus to yield the following transition: Most electrons are captured from the K electron shell, although occasionally an electron may be captured from the L shell or a shell even farther from the nucleus. During electron capture, a hole is created in an electron shell within the atom. This vacancy is filled by an electron cascading from a higher shell, resulting in the release of characteristic radiation or one or more Auger electrons. Many nuclei decay by both electron capture and positron emission, as illustrated below for 22Na: The electron capture branching ratio is the probability of electron capture per decay for a particular nuclide. For 22Na, the branching ratio is 10% for electron capture and 90% of the nuclei decay by positron emission. Generally, positron decay prevails over electron capture when both decay modes occur. A decay scheme for 22Na is shown in Figure 1.14. The 2moc2 listed alongside the vertical portion of the positron decay pathway represents the energy equivalent (i.e. 1.02 MeV or 2moc2) of the additional mass of the products of positron decay. This additional mass includes the greater mass of the neutron as compared with the proton together with the mass of the ejected positron. This amount of energy must be supplied by the transition energy during positron decay. Nuclei that cannot furnish at least 1.02 MeV for the transition do not decay by positron emission. These nuclei increase their N/Z ratios solely by electron capture. Figure 1.14 Radioactive decay scheme for positron decay and electron capture of 22Na. There are a few nuclides that can decay by beta (β−) emission, positron emission, or electron capture. An example is 74As (see Figure 1.15), which undergoes beta (β−) emission 32% of the time, positron emission 30%, and electron capture 38%. All modes of decay result in the transformation of the highly unstable “odd-odd” 74As nucleus (Z = 33, N = 41) into a progeny nucleus with even Z and even N. Figure 1.15 The decay scheme for 74As illustrates competing processes of beta (β−) emission, positron emission, and electron capture. Radioactive decay often leaves the progeny in an exited state necessitating an isometric transition. A common form of isomeric transition is gamma decay, in which the energy is released as a γ-ray. γ-rays are electromagnetic radiation of similar energy to X-rays and are differentiated only by their origin: X-rays result from electron interactions outside the nucleus, whereas γ-rays result from nuclear transitions. The gamma emission process competes with another isomeric transition, internal conversion, in which an electron from an extra nuclear shell carries the energy out of the atom. Sometimes, one or more of the excited states of a progeny nuclide may exist for a finite lifetime. An excited state is termed a metastable state if its half-life exceeds 10−6 seconds (see Section 1.3.2.1). For example, the decay scheme for 99Mo shown in Figure 1.16 exhibits a metastable energy state, 99mTc, that has a half-life of six hours. Figure 1.16 Simplified radioactive decay scheme for 99Mo. Just as electron transitions emit characteristic X-rays, metastatic nuclides emit γ-rays with characteristic energies. For example, 99mTc emits photons of 142 and 140 keV and beta (β−) decay of 60Co releases photons of 1.17 and 1.33 MeV (see Figure 1.17). In the latter case, the photons are released during a cascade of isomeric transitions of progeny 60Ni nuclei from excited states to the ground energy state. Figure 1.17 Radioactive decay scheme for 60Co. An isomeric transition can also occur by the interaction of the nucleus with an electron in one of the electron shells, i.e. internal conversion. When this happens, the electron is ejected with kinetic energy Ek equal to the energy Eγ released by the nucleus, reduced by the binding energy Eb of the electron:
Physics of Radiation and Matter
1.1 Introduction
1.2 Electromagnetic Radiation
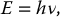

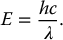

Designation
Frequency (Hz)
Wavelength (m)
Energy (eV)
γ-rays
1018 to 1021
3 × 10−10 to 3 × 10−13
4 × 103 to 4 × 106
X-rays
1015 to 1025
3 × 10−7 to 3 × 10−17
10 to 4 × 1010
Ultraviolet
1015 to 1017
3 × 10−7 to 3 × 10−9
4–400
Visible
4 × 1014 to 7 × 1014
7.5 × 10−7 to 4.3 × 10−7
1.7–2.9
Infrared
1011 to 4 × 1014
0.003 to 7.5 × 10−7
4 × 10−4 to 1.7
Radar and microwave
108 to 1011
3 to 0.003
4 × 10−7 to 4 × 10−4
Television
3 × 107 to 3 × 109
30 to 0.3
4 × 10−8 to 4 × 10−6
FM radio
8.8 × 106 to 1.08 × 108
34 to 2.8
3.6 × 10−8 to 4.4 × 10−7
AM radio
5.35 × 105 to 1.605 × 106
5.61 × 102 to 2.8 × 102
2.2 × 10−9 to 4.4 × 10−9
Electric power
0 to 103
0 to 3 × 105
0 to 4 × 10−12
1.2.1 The Atom
1.2.2 Structure of the Atom
1.2.2.1 Atomic Nomenclature
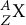 , where X is the chemical symbol (e.g. H, He, and Li) and A and Z are as defined above. There is some redundancy in this symbolism. The atomic number, Z, is uniquely associated with the chemical symbol, X. For example, when Z = 6, the chemical symbol is always C, i.e. the element carbon. As such, the Z subscript is often omitted.
, where X is the chemical symbol (e.g. H, He, and Li) and A and Z are as defined above. There is some redundancy in this symbolism. The atomic number, Z, is uniquely associated with the chemical symbol, X. For example, when Z = 6, the chemical symbol is always C, i.e. the element carbon. As such, the Z subscript is often omitted.
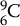 ,
, 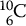 ,
, 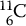 ,
, 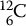 ,
, 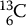 ,
, 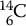 ,
, 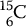 , and
, and 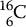 are isotopes of carbon. Isotones are atoms that possess the same number of neutrons but a different number of protons. For example,
are isotopes of carbon. Isotones are atoms that possess the same number of neutrons but a different number of protons. For example, 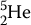 ,
, 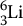 ,
, 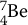 ,
, 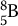 , and
, and 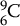 are isotones, each contains three neutrons. Isobars are atoms with the same number of nucleons but a different number of protons and a different number of neutrons. For example,
are isotones, each contains three neutrons. Isobars are atoms with the same number of nucleons but a different number of protons and a different number of neutrons. For example, 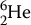 ,
, 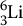 , and
, and 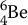 are isobars, with six nucleons each. Finally, isomers represent different energy states for nuclei with the same number of neutrons and protons. Differences between isotopes, isotones, isobars, and isomers are illustrated in Table 1.2.
are isobars, with six nucleons each. Finally, isomers represent different energy states for nuclei with the same number of neutrons and protons. Differences between isotopes, isotones, isobars, and isomers are illustrated in Table 1.2.
Atomic number Z
Neutron number N
Mass number A
Isotopes
Same
Different
Different
Isotones
Different
Same
Different
Isobars
Different
Different
Same
Isomers
Same
Same
Same (different nuclear energy states)
1.2.2.2 Electrons

Element
n
l
ml
ms
Orbital
Shell
Helium (Z = 2)
1
0
0
–½
1s2
K
1
0
0
+½
Carbon (Z = 6)
1
0
0
–½
1s2
K
1
0
0
+½
2
0
0
–½
2s2
L
2
0
0
+½
2
1
−1
–½
2p2
2
1
−1
+½
Sodium (Z = 11)
1
0
0
–½
1s2
K
1
0
0
+½
2
0
0
–½
2s2
L
2
0
0
+½
2
1
−1
–½
2p6
2
1
−1
+½
2
1
0
–½
2
1
0
+½
2
1
1
–½
2
1
1
+½
3
0
0
–½
3s
M

1.2.2.3 Electron Energy Levels and Binding Energies
N
Shell
Hydrogen
Tungsten
1
K
−13.5
−69 500
2
L
−3.4
−11 280
3
M
−1.5
−2810
4
N
−0.90
−588
5
O
−0.54
−74
Binding energy of shell (keV)
Atomic number
Element
K
LI
LII
LIII
1
Hydrogen
0.0136
6
Carbon
0.283
8
Oxygen
0.531
11
Sodium
1.08
0.055
0.034
0.034
13
Aluminum
1.559
0.087
0.073
0.072
14
Silicon
1.838
0.118
0.099
0.098
19
Potassium
3.607
0.341
0.297
0.294
20
Calcium
4.038
0.399
0.352
0.349
26
Iron
7.111
0.849
0.721
0.708
29
Copper
8.980
1.100
0.953
0.933
31
Gallium
10.368
1.30
1.134
1.117
32
Germanium
11.103
1.42
1.248
1.217
39
Yttrium
17.037
2.369
2.154
2.079
42
Molybdenum
20.002
2.884
2.627
2.523
47
Silver
25.517
3.810
3.528
3.352
53
Iodine
33.164
5.190
4.856
4.559
54
Xenon
34.570
5.452
5.104
4.782
56
Barium
37.410
5.995
5.623
5.247
57
Lanthanum
38.931
6.283
5.894
5.489
58
Cerium
40.449
6.561
6.165
5.729
74
Tungsten
69.508
12.090
11.535
10.198
79
Gold
80.713
14.353
13.733
11.919
82
Lead
88.001
15.870
15.207
13.044
92
Uranium
115.591
21.753
20.943
17.163
1.2.2.4 Electron Transitions, Characteristic, and Auger Emission

1.2.2.5 Electronic Conduction
1.2.3 The Nucleus
Number of protons
Number of neutrons
Number of stable nuclei
Even
Even
165
Even
Odd
57
Odd
Even
53
Odd
Odd
6
1.2.3.1 Nuclear Forces and Stability
1.2.3.2 Nuclear Fusion and Fission

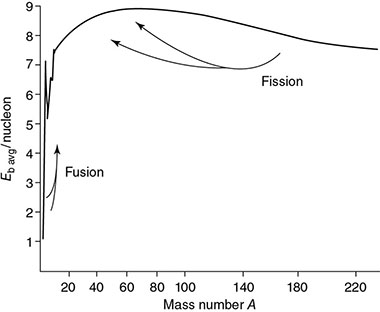
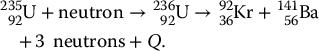
 and
and  ), neutrons, and of course heat and light. Neutrons released during fission may interact with other nuclei and create the possibility of a chain reaction, provided that sufficient mass of fissionable material is contained within a small volume. The rate at which fission can occur in a material may be regulated by controlling the number of neutrons available to interact with fissionable nuclei; this is the method used to control nuclear reactors. Uncontrolled nuclear fission results in an “atomic explosion.”
), neutrons, and of course heat and light. Neutrons released during fission may interact with other nuclei and create the possibility of a chain reaction, provided that sufficient mass of fissionable material is contained within a small volume. The rate at which fission can occur in a material may be regulated by controlling the number of neutrons available to interact with fissionable nuclei; this is the method used to control nuclear reactors. Uncontrolled nuclear fission results in an “atomic explosion.”
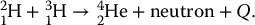
1.2.3.3 Nuclear Spin and Nuclear Magnetic Moments
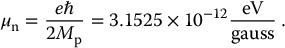
 with six protons and six neutrons has no net magnetic moment because the neutrons and protons are paired up. An atom with an odd number of either neutrons or protons will have a net magnetic moment. For example,
with six protons and six neutrons has no net magnetic moment because the neutrons and protons are paired up. An atom with an odd number of either neutrons or protons will have a net magnetic moment. For example, 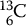 with six protons and seven neutrons has a net magnetic moment because it contains an unpaired neutron. Also,
with six protons and seven neutrons has a net magnetic moment because it contains an unpaired neutron. Also, 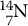 with seven protons and seven neutrons has a net magnetic moment because both proton and neutron numbers are odd. Table 1.7 lists several nuclides with net magnetic moments. The presence of a net magnetic moment for the nucleus is essential to MRI, as only nuclides with net magnetic moments can interact with the magnetic field of an MRI system to provide a signal to form an image.
with seven protons and seven neutrons has a net magnetic moment because both proton and neutron numbers are odd. Table 1.7 lists several nuclides with net magnetic moments. The presence of a net magnetic moment for the nucleus is essential to MRI, as only nuclides with net magnetic moments can interact with the magnetic field of an MRI system to provide a signal to form an image.
Nuclide
Number of protons
Number of neutrons
Magnetic moment (multiple of μn)
1H
1
0
2.79
2H
1
1
0.86
13C
6
7
0.70
14N
7
7
0.40
17O
8
9
−1.89
19F
9
10
2.63
23Na
11
12
2.22
31P
15
16
1.13
39K
19
20
0.39
1.3 Radioactivity
1.3.1 Decay Schemes
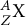 has four possible routes of radioactive decay:
has four possible routes of radioactive decay:
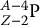 by the emission of a
by the emission of a 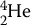 nucleus (Pathway 1)
nucleus (Pathway 1)
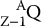 (Pathway 2)
(Pathway 2)
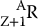 by the emission of an electron from the nucleus (Pathway 3)
by the emission of an electron from the nucleus (Pathway 3)
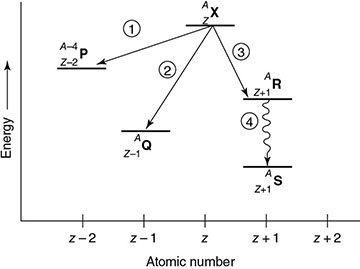
Type of transition
Final A
Final Z
Final N
Comments
Alpha (α) decay
A − 4
Z − 2
N − 2
Fixed amount of released energy per mass–energy conservation
Positron (β+) decay
A
Z − 1
N + 1
Beta particles have an energy distribution with mean energy equal to a third of the maximum energy
Electron capture
A
Z − 1
N + 1
Produces characteristic X-rays and Auger electrons
Beta (β−) decay
A
Z + 1
N − 1
Beta particles have an energy distribution with mean energy equal to a third of the maximum energy
Isomeric transition – gamma (γ) emission
A
Z
N
Metastable if T1/2 > 10−6 s
Isomeric transition (IT) or internal conversion (IC)
A
Z
N
IT and IC are competing processes; IC electrons produce characteristic X-rays and Auger electrons
1.3.1.1 Alpha Decay
 [10]. The alpha particle is a relatively massive, poorly penetrating type of radiation that can be stopped by a sheet of paper or paint. An example of alpha decay is the decay of naturally occurring radium into the inert gas radon by the emission of an alpha particle:
[10]. The alpha particle is a relatively massive, poorly penetrating type of radiation that can be stopped by a sheet of paper or paint. An example of alpha decay is the decay of naturally occurring radium into the inert gas radon by the emission of an alpha particle:
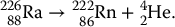

1.3.1.2 Beta Decay

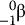 is an electron ejected from the nucleus and
is an electron ejected from the nucleus and 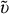 is an antineutrino, a massless neutral particle that is required for the conservation of energy and is the antimatter counterpart of the neutrino. An example of beta negative decay is that of 137Cs:
is an antineutrino, a massless neutral particle that is required for the conservation of energy and is the antimatter counterpart of the neutrino. An example of beta negative decay is that of 137Cs:


 is the energy of the antineutrino.
is the energy of the antineutrino.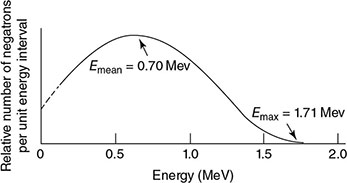
1.3.1.3 Positron Decay and Electron Capture
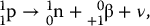
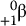 represents a positron ejected from the nucleus during decay and ν is a neutrino that accompanies the positron. The neutrino and antineutrino are similar, except that they have opposite spin and share the same energy balance with their corresponding positron or electron emission.
represents a positron ejected from the nucleus during decay and ν is a neutrino that accompanies the positron. The neutrino and antineutrino are similar, except that they have opposite spin and share the same energy balance with their corresponding positron or electron emission.
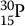 is representative of a positron decay:
is representative of a positron decay:
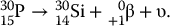
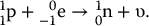

1.3.1.4 Isomeric Transition
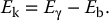
![]()
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree