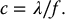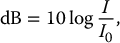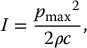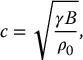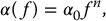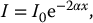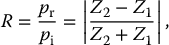9
Ultrasonography
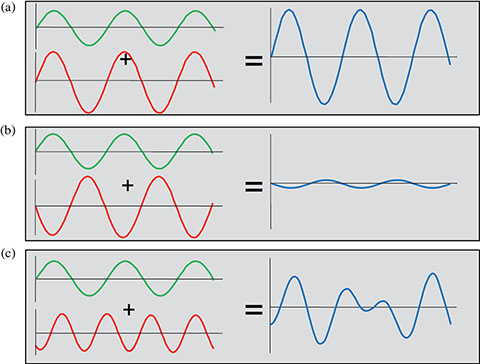
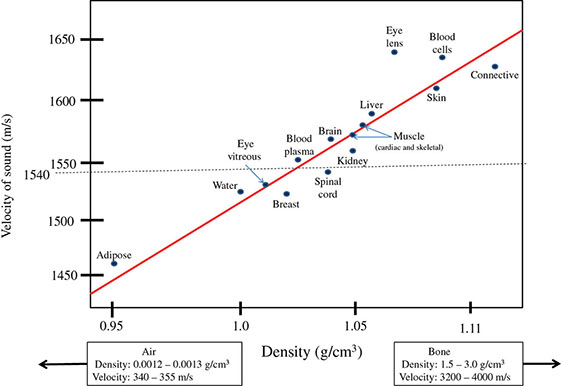
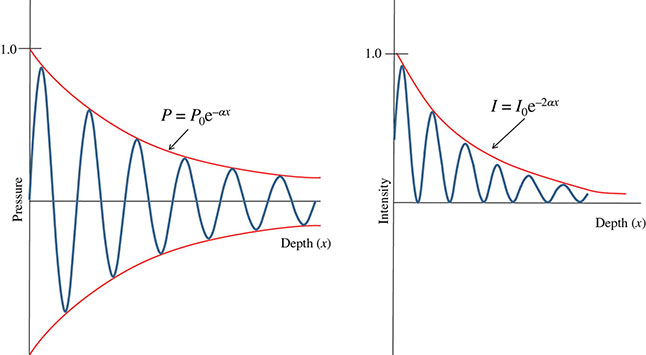
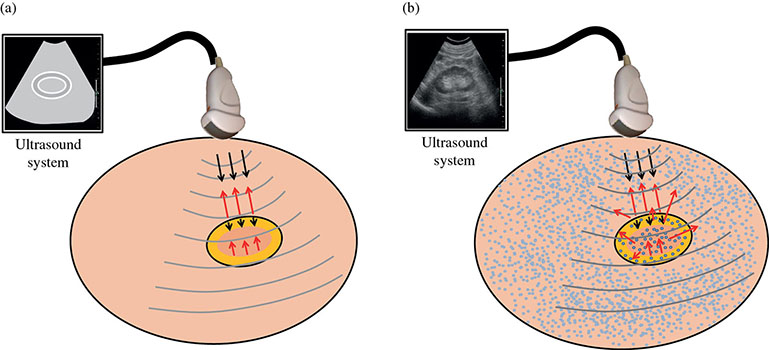
Ultrasonography, or ultrasound in short, is an imaging modality that exploits the acoustical properties of the human tissue in conjunction with an ultrasonic energy source and receptor to characterize the boundaries of tissues and movements within the human body. This modality is one of two main medical imaging modalities, along with magnetic resonance imaging (MRI), that does not use ionizing radiation. However, a source of energy is still employed, and the body’s response to the energy is the foundation of image formation. The most common mode of ultrasonic imaging is through echo location, where the timing of the reflection of ultrasonic waves from the body structures forms the imaging signal. Ultrasound has a depth of penetration in the body in the multicentimeter range (dependent on the wave frequency) and is limited in areas with air or bone, limiting its reach and application. However, given its safety, low cost, and quantitative attribute, its use in obstetrics/gynecology and cardiac care has become ubiquitous, making it an essential component of quality care. Sound is a mechanical disturbance from a state of equilibrium that propagates through an elastic material or medium. An elastic material is a collection of molecules that are in continuous random motion. When no external force is applied to the material, the molecules are distributed uniformly. When a force is applied to the material, each molecule of the material will become closer to the molecules immediately adjacent to it. As the molecules are pressed together, there is an increased pressure at that location; the region of increased pressure is termed a zone of compression. The increased pressure is not at equilibrium, and therefore, the molecules in the zone of compression exert a force on the neighboring molecules to return to equilibrium. This force produces a new zone of compression, and this process propagates through the material. That is, a mechanical disturbance introduced into the material (e.g. compression of spacing between molecules) will travel through the material in a direction away from the source of the disturbance. When a zone of compression migrates through a material, the molecules that surround it will move into this region to restore it to its normal particle density. This creates a second region where the particle density is reduced compared to equilibrium, termed a zone of rarefaction. Thus, the compression zone (high density of molecules) is followed by a rarefaction zone (low density of molecules). Applying force a second time establishes a second compression zone that follows the zone of rarefaction through the material. If the force oscillates on then off continuously, alternating zones of compression and rarefaction are propagated through the material. The propagation of these zones establishes a wavefront of disturbance in the material, known as a longitudinal wave because the motion of the molecules in the material is along the direction of wave propagation. This is different from transverse wave motion, e.g. motion of the surface of water waves, where the molecular motion is perpendicular to the direction of propagation. During the process of sound wave propagation, the molecules are only displaced by a small amount in the compression and rarefaction zones. In fact, it is the change in density that is being propagated through the material, not the molecules themselves. A zone of compression and an adjacent zone of rarefaction constitute one cycle of a sound wave. A wave cycle can be represented as a graph of local pressure (particle density) in the material vs. distance in the direction of the sound wave (Figure 9.1). This wave nature of sound allows the use of classical mechanics of wave motion to be used when analyzing ultrasound imaging. Therefore, the distance covered by one cycle of the wavefront is the wavelength λ of the sound wave. The number of cycles per unit time introduced into the material is the frequency f of the wave, expressed in units of hertz, kilohertz, or megahertz, where 1 Hz equals 1 cycle/s. From this, the speed c of the compression/rarefaction wavefront through a material can be calculated as Figure 9.1 Different representations of sound propagation through a material. The maximum pressure or density of molecules in a wave cycle is the amplitude of the sound wave. The cyclical nature of waves is also important because when two waves meet, they interfere with each other, affecting the wave shape (see Figure 9.2). There are two extremes of interference. In constructive interference, the waves are in phase, i.e. one zone of compression meets another zone of compression. In destructive interference, the waves are out of phase, i.e. the zone of compression meets a zone of rarefaction. Waves undergoing constructive interference add their amplitudes, whereas waves undergoing destructive interference may completely cancel each other. If the waves have different frequencies, the resultant-combined wave may produce a complex compression and rarefaction form. By combining waves of multiple frequencies, the compression and rarefaction zones can be created, so the transition between them is more abrupt. This allows the beam to be “shaped” by using known combinations of waves. Figure 9.2 When multiple waves are combined, the resultant wave will exhibit interference, which in extreme cases of constructive and destructive interference leads to addition (a) or cancellation of the two waves (b). For waves that vary in frequency and/or phase, the resultant wave created can exhibit a complex wave pattern (c). A sound wave with a frequency between about 20 and 20 000 Hz is audible to the human ear. An infrasonic wave is a sound wave below 20 Hz and is not audible to the human ear. An ultrasonic (or ultrasound) wave has a frequency greater than 20 000 Hz and is also inaudible. In clinical diagnostic ultrasound, frequencies between 1 and 20 MHz are used. As an ultrasound wave passes through a medium, it transports energy through the medium; therefore, it is a type of radiation. The rate of energy transport is the wave’s power. Medical ultrasound is produced in beams that are usually focused into a small area, and the beam is described in terms of the power P per unit area a, defined as the beam’s intensity. Power is energy E per time t; therefore, the intensity can be expressed as The relationships among the quantities and units pertaining to intensity are summarized in Table 9.1. Table 9.1 Quantities and units pertaining to ultrasound intensity The intensity of sound is usually described relative to some reference intensity. For example, the intensity of ultrasound waves sent into the body may be compared with that of the ultrasound reflected back to the surface by structures in the body. For many clinical situations, the reflected waves at the surface may be as little as 100th the intensity of the transmitted waves. Waves reflected from structures at the depths of 10 cm or more below the surface may be lower in intensity by a much larger factor. A logarithmic scale is most appropriate for recording data that are spread over a range of values that vary by several orders of magnitude. This along with the foundation that human sensory is logarithmic in response to sonic stimuli is the basis for the use of the decibel (dB) scale in acoustics as where I0 is the reference intensity. Using a logarithm scale provides an easy way to understand changes in the intensity. Several rules can be extracted from the decibel scale: No universal standard reference intensity exists for ultrasound. Thus, the statement “ultrasound at 50 dB was used” is nonsensical. However, a statement such as “the returning echo was 50 dB below the transmitted signal” is informative because the transmitted signal then becomes the reference intensity for this particular application. Conversely, for audible sound, a statement such as “a jet engine produces sound at 100 dB” is appropriate because there is a generally accepted reference intensity of 10−16 W/cm2 for audible sound [1]. As a comparison, the human ear is unable to distinguish a difference in loudness less than about 1 dB, and a 1-kHz note at 120 dB (10−4 W/cm2) is painfully loud. As intensity is defined as power per unit area and power is energy per unit time (see Table 9.1), Eq. (9.2) may be used to compare the power or the energy contained within two ultrasound waves as However, as sound propagation produces a change in the molecular density, a more practical definition would be related to the pressure change. Ultrasound wave intensity is related to maximum pressure (pmax) in the medium as where ρ is the density of the medium (g/cm3) and c is the speed of the wave in the medium [2]. Substituting Eq. (9.5) for I and I0 in Eq. (9.3) yields When comparing the pressure of two waves, Eq. (9.6) may be used directly. That is, the pressure does not have to be converted to intensity to determine the decibel value. The velocity of an ultrasound wave through a medium varies with the physical density and stiffness of the material. The stiffness is given by the material’s bulk modulus, a measure of the resistance to compression. The bulk modulus B is related to the velocity of sound propagation by the following relationship defined at the material’s ratio of specific heat γ and equilibrium density ρ0. In low-density media, such as air and other gases, molecules may move over relatively large distances before they influence neighboring molecules, and the velocity of an ultrasound wave is relatively low. In solids, the motion of molecules is constrained by the molecular architecture and the velocity of ultrasound is relatively high. Liquids exhibit ultrasound velocity intermediates between those in gases and solids. The velocity of sound relative to the density for various tissues is shown in Figure 9.3. Figure 9.3 Velocity of sound propagation vs. density for tissue [3]. Reproduced with permission of Acoustical Society of America. With the notable exceptions of lung (i.e. air) and bone, biological tissues yield roughly equal velocities, with an average velocity of 1540 m/s. For most clinical applications, an ultrasound system assumes that the velocity of sound propagation does not change and is equal to this average. However, as there are small changes in velocity between tissues, the wavelength of ultrasound changes as it moves across tissue boundaries (see Eq. (9.1)), with the frequency remaining relatively constant. The velocity of an ultrasound wave should be distinguished from the velocity of the molecules whose displacement into zones of compression and rarefaction constitutes the wave. The molecular velocity describes the small change in location and therefore the velocity of the individual molecules in the medium. It is the molecular velocity that will determine the attenuation of the sound energy as it propagates through a material. As an ultrasound beam propagates through a material, its energy decreases with distance traveled. As with all radiation, the term “attenuation” refers to any mechanism (i.e. absorption, reflection, and scattering) that removes energy from the ultrasound beam. Ultrasound is absorbed by the material if part of the wave’s energy is converted into other forms of energy, such as an increase in the random motion of molecules (i.e. heat). Ultrasound is reflected if there is an orderly deflection of all or part of the wave energy. If part of an ultrasound wave changes direction in a less orderly manner, the event is usually described as scatter. Absorption of sound energy is described by the theory of fluid viscosity. When sound moves through a material, some of the force of the pressure wave must overcome the viscous drag of the particles in the medium as they slide past each other. This produces a resistance to the motion in the direction of the wave propagation (i.e. longitudinal direction). The resistance to the sound wave’s kinetic energy results in work being done on the particles by the sudden compression and the kinetic energy of this translational motion of the molecules causes the pressure, density, and temperature to rise. Some of this energy is subsequently fed into rotational and vibrational energy of the molecules and the pressure falls, this is termed “relaxation.” If the compression is reversed sufficiently quickly, negligible energy is lost, and the work of compression can be recovered during rarefaction. However, if the compression–rarefaction cycle is sufficiently slow, thermodynamic equilibrium between the different energy modes has time to be established and again the process is reversible. Finally, if the oscillation period lies somewhere in between these extremes, some sound energy will be irreversibly lost to the internal energy of the material. Consequently, the material will not behave perfectly elastically and will have a complex bulk modulus. Mathematically, this introduces a dynamic resistance coefficient of bulk viscosity to the wave equation of sound motion, meaning Eq. (9.7) is not accurate. In addition to the energy loss in the direction of the wave propagation, there is also a resistance transverse to the wave motion. The normal stress (pressure) in a material is explained by molecular collisions. In a material of uniform temperature at rest, molecules move randomly with equal probability in all directions and molecular collisions produce zero average net flux of momentum across the material, so there is static equilibrium. However, if there are two areas flowing at different speeds, velocity is discontinuous at the interface between the areas and the molecules now carry the momentum associated with random motion plus the mean flow. Molecules passing through the interface from the higher velocity region ultimately collide with molecules of the slower moving material (or stationary area), transferring to them greater momentum than is passed in the opposite direction. This effect produces a stress component tangential to the interface, i.e. a shear stress that acts to reduce the mean velocity difference (see Figure 9.4). At very low relative speeds, the tangential velocity exhibits a continuous, smooth variation with distance from the interface, which is known as the rate of shear. However, the shear area formed is inherently unstable and develops transverse waves away from the interface. At a sufficiently high relative speed, this breaks up into turbulence (this is the origin of the noise from aircraft jet engines). Kinetic theory and experiment show that the viscous stress is directly proportional to the rate of shear, and this introduces a dynamic resistance coefficient of shear viscosity to the wave equation of sound motion. These viscous stresses are essentially nonconservative and dissipate kinetic energy into heat. Figure 9.4 Different representations of shear wave propagation through a material. Therefore, the attenuation of sound has two components: bulk viscosity and shear viscosity. Both have a complicated nature to their development and propagation. Although understanding this relationship is not required for interpreting images in most clinical uses, the components are important in more advanced imaging applications and in safety concerns [1,4–6]. One clinical use of shear waves is elastography [7]. Elastography is the measurement of the elastic properties of a material, which are determined through an analysis of its compressibility. Most methods for quantifying this compressibility utilize the theories of Young’s modulus and apply them to the tissue. Elastography can be performed by palpating (i.e. using external pressure) to compress an object, but it can also be accomplished with the shear wave produced from the longitudinal ultrasound wave, this is often termed acoustic radiation force impulse (ARFI) elastography. As shown in Eq. (9.7), the speed of the wavefront through a material is determined by its density and the bulk modulus. If a force is applied to a material, it will be compressed, and the speed of the wavefront will change. Measuring the change in position before and after a shear wave allows calculation of the elasticity of the material. The compression and rarefaction regions from shear wave propagate perpendicular from the longitudinal wave and are attenuated rapidly. Therefore, the frequency of the shear waves does not interfere with standard image acquisition (see Section 9.6.2). The attenuation of ultrasound in a material is described by the attenuation coefficient α (dB/cm). The attenuation coefficient is known only approximately and varies significantly with tissue type and environment because of the complexity of the bulk and shear viscosity components. Also, attenuation is dependent on the frequency of the sound. The relationship of the attenuation coefficient and frequency has been derived as where α0 is the attenuation measured at a reference frequency (e.g. 1 MHz), f is the frequency of the ultrasound in MHz, and n is between values of 0.0–2.0 and has units of dB/(cm MHz) [5,6]. For pure fluids, attenuation results entirely from absorption because there is not a large variation in the structure of the molecules to cause scatter and for frequencies up to 3 GHz n = 2.0. For most soft tissue, n varies from 0.5 to 1.5 and a first-order approximation for determining the attenuation at different frequencies used in medical ultrasound can be assumed to be between 0.3 and 0.6 dB/(cm MHz). Table 9.2 shows the attenuation coefficient for several tissues. Note that the attenuation of ultrasound is very high in both bone and air. This property and the large reflection coefficient of tissue–bone and tissue–air interfaces (see Section 9.2.3.3) make it difficult to visualize structures lying behind bone and within the lungs. However, little attenuation occurs in water, so this medium is a very good transmitter of ultrasound energy. Table 9.2 Attenuation coefficients, α, for 1-MHz ultrasound Equation (9.8) states that the attenuation of ultrasound energy increases with frequency. That is, higher frequency ultrasound is attenuated more readily and is therefore less penetrating than the ultrasound of lower frequency. The energy loss in a medium composed of layers of different materials is the sum of the energy loss in each layer. Using the attenuation coefficient, the amount of energy lost as the sound propagates through a material can be determined. Similar to other forms of radiation, the attenuation of sound energy is an exponential process and is given by where x is the distance traveled. This can also be solved as a change in the pressure with distance traveled (see Figure 9.5). The factor of 2 difference between the equations shown in Figure 9.5 is due to the relationship of pressure to intensity (see Eq. (9.5)). Figure 9.5 Pressure and intensity remaining in a sound beam as a function of the depth of penetration into a medium. The diagram is an exaggeration of the decrease because of attenuation. In soft tissue, the decrease is only a very small amount per wavelength. The behavior of a sound wave when it encounters an obstacle depends on the size of the obstacle compared with the wavelength of the sound. If the obstacle’s size is large compared with the wavelength of sound, and if the obstacle is relatively smooth, then the wave retains its form even if it changes the direction. Part of the sound wave may be reflected, and the remainder transmitted through the interface as a wave of lower intensity. Reflection in which the ultrasound beam retains its integrity is said to be “specular,” from the Latin for mirror. Reflection of visible light from a plane mirror is an optical example of specular reflection. Conversely, if the size of the obstacle is comparable to or smaller than the wavelength of the ultrasound, the obstacle will scatter energy in various directions. Some of the ultrasound energy may return to its original source after this nonspecular scatter but probably not until many scatter events have occurred. An optical example of nonspecular reflection occurs when a mirror is coated with steam and the water droplets are nonspecular reflectors that scatter the light beam. In ultrasound imaging, visual identification of the boundaries between organs is possible because of specular reflection, and nonspecular reflection creates the appearance of tissue parenchyma (see Figure 9.6). This is due to structures in tissue, such as collagen fibers, that are smaller than the wavelength of ultrasound and cause scatter that returns to the ultrasound source through multiple pathways. The sound that returns to the source from such nonspecular reflectors is no longer a coherent beam. Instead, it is the sum of many constituent waves and produces a complex pattern of constructive and destructive interference back at the source. This interference pattern, known as speckle, provides the characteristic ultrasonic appearance of the interior of organs and tissue. Figure 9.6 Graphic example of specular reflections (a) and nonspecular (b). Nonspecular reflections produce scatter of the ultrasound beam from small structures within tissue and provide the internal appearance of organs in an image. The fraction of the impinging energy reflected from an interface depends on the difference in the acoustic impedance of the material on opposite sides of the interface. For this discussion, reflection is assumed to occur at interfaces that have dimensions greater than the ultrasound wavelength, i.e. specular reflection. The mechanism by which sound waves are altered at the interfaces between materials is determined based on the impedance to the sound waves within each material. Impedance is a property of a sound medium that is analogous to resistance or impedance in electrical circuit theory. When sound energy is traveling through one medium and encounters a discontinuity in the impedance to its motion, part of the energy will be transferred across the boundary and part will be reflected back into the original medium. The greater the difference between the impedances of the materials, the greater the percentage of energy reflected. Acoustic impedance Z of a medium is the product of the density ρ of the medium and the velocity c of the ultrasound wave in the medium as Acoustic impedance is expressed in units of Rayleighs (rayls), and 1 rayl = 1 kg/(m2 s). The acoustic impedances of several materials are listed in Table 9.3. Table 9.3 Approximate acoustic impedances of selected materials Using the impedance values, the amount of energy reflected and transmitted at an interface between two materials can be estimated. For an ultrasound wave incident perpendicular to an interface, the fraction of the incident energy that is reflected (expressed as the reflection coefficient R) is where pr and pi are the pressure of the reflected and incident sound waves and Z1 and Z2 are the acoustic impedances of the two media. Similarly, the fraction of the incident energy that is transmitted across an interface is described by the transmission coefficient T as When there is a large impedance mismatch at an interface, most of the energy of an ultrasound wave is reflected and only a small amount is transmitted across the interface. For example, ultrasound beams are reflected strongly at air–tissue and air–water interfaces because the impedance of air is much less than that of the tissue or water. Because of the high value of the coefficient of ultrasound reflection at an air–tissue interface, water baths and various creams and gels are used during ultrasound examinations to remove air pockets (thereby obtaining good acoustic coupling) between the ultrasound transducer and the patient’s skin. With adequate acoustic coupling, the ultrasound waves will enter the patient with little reflection at the skin surface. Similarly, strong reflections of ultrasound occur at the boundary between the chest wall and the lungs and at the millions of air–tissue interfaces within the lungs. Because of the large impedance mismatch at these interfaces, the use of ultrasound as a diagnostic tool for the lungs is limited. The impedance mismatch is also high between soft tissues and bone, and the use of ultrasound to identify tissue characteristics in regions behind bone is limited. Because of the large differences in the attenuation coefficient and acoustic impedance, microencapsulated gas-filled containers are used as contrast media in ultrasound. Note that the discussion of ultrasound reflection above assumes that the ultrasound beam strikes the reflecting interface at an angle of 0°, meaning perpendicular to the surface. In reality, ultrasound impinges upon interfaces in the body at all angles. For any ultrasound beam angle of incidence θi, the angle of the reflected ultrasound energy θr equals the angle of incidence, and the angle of transmittance/refraction θt is given by Snell’s law (see Figure 9.7). Figure 9.7 Ultrasound reflection and transmission at an interface. The angle of incidence θi equals the angle of reflection θr and the angle of transmittance or refraction θt is given by Snell’s law. These nonzero angle interactions complicate the determination of the reflection coefficient. In these cases, the reflection coefficient is given by In a typical medical ultrasound examination, the same apparatus (i.e. transducer – see Section 9.3) both transmits and detects ultrasound. Therefore, in clinical use, very little reflected energy will be detected if the ultrasound strikes the interface at an angle more than about 3° from perpendicular. The cosine of 3° is 0.9986, so Eq. (9.13) is essentially equal to Eq. (9.11) except for special acquisition methods that may utilize separate transmit and receive systems. As an ultrasound beam crosses an interface obliquely between two media, its direction is changed (i.e. the beam is bent). If the velocity of ultrasound is higher in the second medium, the beam enters this medium at a more oblique angle. This behavior of an ultrasound wave being transmitted obliquely across an interface is termed refraction. The relationship between incident and refraction angles is described by Snell’s law (see Figure 9.7) expressed as Two conditions are required for refraction to occur: (i) the sound wave must strike an interface at an angle other than 0°; and (ii) the speed of sound must differ on opposite sides of the interface. If an ultrasound beam impinges very obliquely upon a medium and the ultrasound velocity is higher in the second medium, the beam may be refracted such that no ultrasound energy enters the medium. The incidence angle at which refraction causes no ultrasound to enter a medium is termed the critical angle θc (see Figure 9.8). Figure 9.8 For an incidence angle θi equal to the critical angle θc, refraction causes the sound to be transmitted along the surface of the material. For incidence angles greater than θc, sound transmission across the interface is prevented by refraction. A transducer is any device that converts one form of energy into another; an ultrasound transducer converts electrical energy into mechanical energy and vice versa. Transducers for ultrasound imaging consist of one or more piezoelectric crystals or elements. The basic properties of ultrasound transducers (including resonance, frequency response, focusing, etc.) can be illustrated in terms of single-element transducers. However, imaging is almost always performed with multiple-element arrays of piezoelectric crystals. In 1880, Pierre and Jacques Curie discovered the piezoelectric effect and thereafter Paul Langevin attempted to develop piezoelectric substances as senders and receivers of high-frequency mechanical disturbances (i.e. sound waves) through materials [8]. The specific application was the use of sound to detect submarines during World War I. This technique, sound navigation and ranging (SONAR) finally became practical during World War II. Industrial uses of ultrasound began in 1928 with the suggestion of Soviet physicist Sergei Sokolov that it could be used to detect hidden flaws in materials [9]. The piezoelectric effect is only exhibited by certain crystals. These materials develop a voltage across opposite surfaces in response to applied pressure, or conversely, when a voltage is applied across opposite surfaces, the crystal will change its shape [10–12]. Therefore, this effect can be used to both produce the pressure wave from the crystal and to record an electrical signal in response to a returning sound wave. The magnitude of the electrical signal produced in the crystal varies directly with the wave pressure of the incident ultrasound. Similarly, application of a voltage across the crystal causes deformation of the crystal (either compression or extension, depending on the polarity of the voltage) that can be controlled to produce the ultrasound wave. The movement of the surface of a piezoelectric crystal used in diagnostic imaging is on the order of a few micrometers at a rate of several million times per second. Essentially, all diagnostic ultrasound units use piezoelectric crystals for the generation and detection of ultrasound. Many crystals exhibit the piezoelectric effect at low temperatures but are unsuitable as ultrasound transducers because their piezoelectric properties do not exist at room temperature. A number of piezoelectric crystals (including quartz and Rochelle salts) that can produce the effect near room temperature occur in nature. However, crystals for clinical use are almost all manmade ceramic ferroelectrics; the most common are barium titanate, lead metaniobate, and lead zirconate titanate (PZT). In some transducers of newer design, the piezoelectric ceramic is mixed with epoxy to form a composite ceramic [13]. The piezoelectric crystal is the functional component of an ultrasound transducer, and a piezoelectric crystal exhibits its greatest response at a resonance frequency. The resonance frequency is determined by the thickness of the crystal (i.e. the dimension of the crystal in the direction of propagation of the ultrasound beam). As the crystal goes through a complete cycle from contraction to expansion, compression waves move toward the center of the crystal from opposite crystal faces. If the crystal thickness equals one wavelength of the sound waves, the compressions arrive at the opposite faces just as the next crystal contraction begins. Thus, the compression waves oppose the contraction and diminish or “dampen” the crystal’s response. Therefore, it is inefficient to use a crystal with a thickness of one wavelength because energy would be wasted. However, if the crystal thickness equals half of the wavelength, a compression wave reaches the opposite crystal face just as expansion is beginning to occur. Each compression wave produced in the contraction phase aids in the expansion phase of the cycle. A similar result is obtained for any odd multiple of half wavelengths (e.g. 3λ/2 and 5λ/2) with the crystal progressing through more than one cycle before a given compression wave arrives at the opposite face. However, additional crystal thickness produces more attenuation of the energy within the crystal, so the most efficient operation is achieved for a crystal with a thickness equal to half the wavelength of the desired ultrasound wavelength. A crystal of half-wavelength thickness l resonates at frequency
9.1 Introduction
9.2 Sound Properties

9.2.1 Intensity and Pressure
Quantity
Definition
Unit
Energy (E)
Ability to do work
J
Power (P)
Rate at which energy is transported
W (J/s)
Intensity (I)
Power per unit area
W/cm2
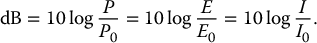
9.2.2 Velocity
9.2.3 Attenuation
9.2.3.1 Absorption

9.2.3.2 Attenuation Coefficient
Material
α (dB/cm)
Material
α (dB/cm)
Water
0.0002
Brain
0.60
Blood plasma
0.07
Muscle (skeletal)
0.74
Vitreous of eye
0.10
Breast
0.75
Blood cells
0.28
Lens of eye
0.80
Adipose
0.30
Kidney
1.00
Skin
0.35
Connective
1.57
Liver
0.50
Bone
20.0
Muscle (cardiac)
0.52
Lung (air)
40.0
9.2.3.3 Reflection
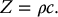
Material
Acoustic impedance (kg/(m2 s1)) × 10−4
Air (at standard temperature and pressure)
0.0004
Fat
1.38
Vitreous of eye
1.50
Water
1.50
Brain
1.55
Blood
1.61
Kidney
1.62
Soft tissue (mean value)
1.63
Spleen
1.64
Liver
1.65
Muscle
1.70
Lens of eye
1.85
Bone
6.10
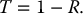
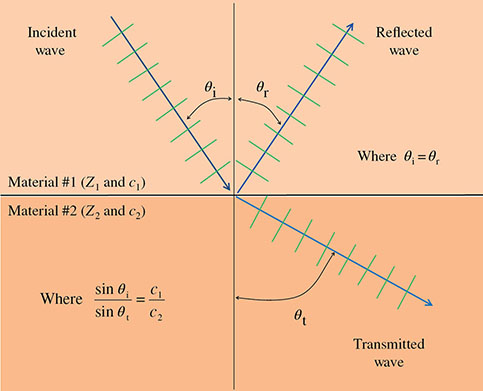
9.2.3.4 Refraction
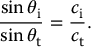
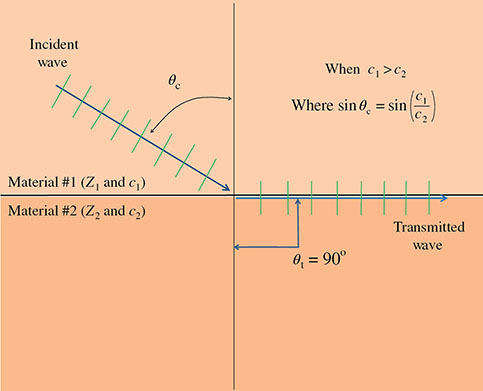
9.3 Transducers
9.3.1 Piezoelectric Effect
9.3.2 Transducer Design
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree