Contrast Agents and Relaxation Effects
John C. Gore
Matthew R. Hight
James M. Joers
Richard P. Kennan
H. Charles Manning
In many clinical applications of MRI the differences of intrinsic magnetic resonance properties (e.g., relaxation times or proton density) between adjacent tissues are great enough so that, by appropriate choice of pulse sequence, neighboring structures can be distinguished and the image contrast is sufficient for clinical purposes. Contrast in MR images arises mainly from the heterogeneous distribution of tissue relaxation times and water contents, and the lack of need for contrast materials in many important applications is one of the major advantages of MRI. There, nonetheless, remain other important areas in which administration of an external contrast agent is essential; to differentiate structures that are very similar in appearance, to provide additional specificity in describing regions of abnormal signal, to opacify or highlight spaces, or to depict specific properties such as tissue vascularity and perfusion. Moreover, external agents also have the potential to report vital physiological characteristics and cellular properties such as pH or metabolite content. Consequently, there has been considerable activity for many years to develop agents that, when introduced into the body, alter the MRI signals from tissues for a variety of clinical purposes. Although the range and type of products approved for use in human subjects are still small, there continue to evolve many creative ideas for the design and use of novel agents that probe new tissue properties or functions which are especially important in preclinical studies of experimental animal models and which may ultimately find applications in human subjects.
In common with other modalities, MRI contrast agents must satisfy a number of practical constraints, not simply that of producing a large effect in an imaging study. There are requirements that are determined by any particular application. The materials must be of low or tolerable toxicity, be biocompatible, have the desirable form (e.g., for gastrointestinal use, as opposed to intravenous use), have the desired excretion pathway, and where possible be targeted with an appropriate organ or even cellular or molecular specificity. These design features are all important, but several of them will not be discussed much further in this chapter. Instead, we will at first concentrate mainly on the underlying mechanisms of action that determine the efficacy of an agent as a contrast material in MRI. We will emphasize the physical principles that affect the mode of action of MR contrast agents, and consider the factors that affect the fundamental efficacy of these basic interactions. The manner in which various molecular or biological factors modify the efficacy of MR contrast agents in vivo is itself a unique feature of MR agents that distinguishes them from x-ray agents or nuclear probes. We will then illustrate how physiological and structural properties of tissue can affect the relaxation efficacy of MR contrast agents in ways that are unique to this modality, which can not only complicate the interpretation of MRI signals following contrast administrations but also provide a potential source of additional information. In addition, in recent years radiological imaging has entered the era of so-called molecular imaging, and within MRI there are ambitions to create agents that report on specific molecular or cellular events, such as gene expression, and their downstream physiological consequences, such as changes in tissue metabolism. Building upon our discussion of environmental factors contributing to relaxation efficiency, we will describe some recent developments aimed at the rational design of “smart” contrast agents, whereby the agent’s relaxation efficiency is dependant upon the tissue microenvironment, and how this characteristic may be employed to report on local conditions in tissues and fluids of interest. We will also comment on the difficulties of achieving true molecular imaging with MRI because of the relatively large amounts of contrast agent needed for detection. Rather than discuss specific applications or potential new agents, we will concentrate in detail on the design and reasons for the selection of the materials that are in use today, with special emphasis on explaining and rationalizing the choices of gadolinium chelates, macromolecular complexes containing gadolinium, paramagnetic agents designed to shift proton resonance frequencies, and superparamagnetic iron oxide particles as practical agents.
In any NMR image the signal intensity at a given location depends on a combination of different factors: there may be several different proton populations present (such as fat and water) each with its own proton density Mo, relaxation times T1 and T2, and there may be intrinsic variations in the magnetic susceptibility and associated field gradients, causing differences in T2*. Good contrast is obtained between different regions when these intrinsic properties vary, e.g., when T1 is longer in gray matter than white matter. In clinical imaging, primary determinants of contrast are the relaxation times, so it is natural to design contrast agents that attempt to modify T1, T2, or T2*. Another important factor is that it is usually difficult and dangerous to significantly alter proton density, though simple liquids can be used to fill spaces in the GI tract. Thus a useful prelude to a consideration of the design of contrast agents is the first to review the factors that determine relaxation times in tissues.
Relaxation Theory in Solutions
First, consider some underlying basic concepts of nuclear magnetic resonance (NMR). Each hydrogen nucleus (a single proton) possesses spin (or angular momentum) which in turn provides it with a magnetic moment, so that it can be considered like a small bar magnet (a dipole) with separate poles. When a
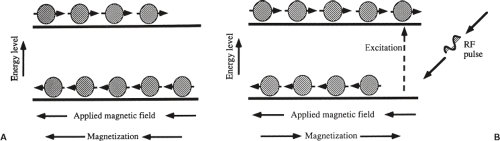
large number of such magnetic nuclei are placed in an external magnetic field they tend to align along the direction of the field. However, the nuclear magnetic energy states obey the rules of quantum theory, so only specific energy states are permitted, analogous to the behavior of electrons orbiting a nucleus being constrained to specific energy levels and shells. For protons in a magnetic field, this means only two orientations of the nuclear magnet relative to the field direction are allowed, which we can consider as parallel (or UP) and antiparallel (or DOWN) to the magnetic field. These two states correspond to the two allowed energy levels (Fig. 3.1A). The UP position corresponds to a lower energy level, so a larger number of protons populate this energy level, and the summed effect is to produce a net magnetization in the direction of the field.
large number of such magnetic nuclei are placed in an external magnetic field they tend to align along the direction of the field. However, the nuclear magnetic energy states obey the rules of quantum theory, so only specific energy states are permitted, analogous to the behavior of electrons orbiting a nucleus being constrained to specific energy levels and shells. For protons in a magnetic field, this means only two orientations of the nuclear magnet relative to the field direction are allowed, which we can consider as parallel (or UP) and antiparallel (or DOWN) to the magnetic field. These two states correspond to the two allowed energy levels (Fig. 3.1A). The UP position corresponds to a lower energy level, so a larger number of protons populate this energy level, and the summed effect is to produce a net magnetization in the direction of the field.
 FIGURE 3.1 A: Protons are spin 1/2 nuclei so that they may occupy one of only two allowed energy levels that correspond to two different orientations in the applied field. In equilibrium, the lower energy level is preferred, so more nuclei align with the field than in the opposite sense. B: The energy difference between the two levels corresponds to a discrete transition that can be excited by absorption of a quantum of energy of precisely the right size. This corresponds in NMR to an RF excitation. |
The populations of the two energy levels in equilibrium depend on the energy difference between them, ΔE, which is proportional to Bo, the static applied field strength. ΔE is, in practice, very small. For example, for protons in a magnetic field of 1 Tesla (T), ΔE is only 1.76 × 10−7 eV, which is an insignificant energy difference on the chemical or molecular scale. Because the energy difference is so small, the preference for taking one alignment rather than the other is also very weak, so that the difference in numbers of spins pointing up rather than down is very small (about 10 in a million).
MRI acquisitions involve inducing transitions between the two energy levels by absorption or emission of quanta with energy ΔE, which is analogous to the transitions of electrons between orbits in an atom. In the presence of not only a static field but also of an additional alternating field of frequency ω0, where ω0 satisfies the relation
where h is the Planck’s constant, and the spin system absorbs energy so that the population of the upper energy level increases while that of the lower level decreases (Fig. 3.1B). Because radiation of only a precisely defined frequency (the Larmor frequency) is effective, the process is called a resonant process. For hydrogen nuclei in a field of 1 T, ω0 is 42.6 MHz, which is called a radiofrequency (RF). If the energy absorbed is sufficient to equalize the populations of the two levels, saturation is said to have occurred. A completely or a partially saturated system will return to equilibrium because of two simultaneous processes. First, the absorbed energy will be redistributed within the spin system by processes in which every transition of a nucleus from a higher to a lower level is accompanied by a transition of a nucleus from the lower to the higher state, the process called spin–spin relaxation. Second, there will be a gradual loss of energy to the other nuclei and electrons in the material, collectively called the lattice, resulting from transitions of nuclei from the upper to the lower state. This second process is spin–lattice relaxation. The time constants characterizing these two processes are T2 and T1, respectively, the spin–spin (or transverse) and spin–lattice (or longitudinal) relaxation times.
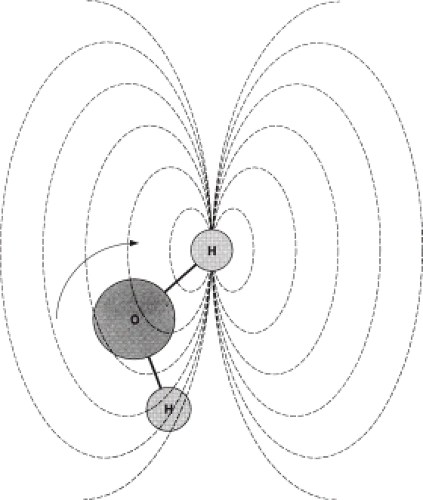
The time constants T1 and T2 yield valuable information about the local interactions experienced by nuclei. T1 describes the rate at which a nonequilibrium spin distribution exponentially approaches equilibrium following absorption of RF energy, or conversely, the time to establish magnetization along the field direction when the object is first introduced. However, an excited nuclear spin does not spontaneously lose its energy but relies almost entirely on interaction with the surrounding material. Spin–lattice relaxation, where the lattice is the environment surrounding the nucleus, and includes the remainder of the host molecule as well as other solute and solvent molecules, occurs because of interactions of the excited nuclear spin dipole with the random fluctuating magnetic fields that exist on an atomic scale inside tissues. These originate from neighboring nuclei or electrons and are modulated by the motion of other surrounding dipoles in the lattice which have components fluctuating with the same frequency as the resonance frequency, ω0. Spin–lattice relaxation is a type of stimulated recovery, in which the spins that have been excited to the upper energy level by the transmitted RF pulse are encouraged to return to the lower level by the action of an alternating magnetic field of appropriate frequency. This stimulated recovery is very efficient when there is a local fluctuating field that can provide a magnetic interaction at the Larmor frequency, so that there is available a quantum of energy exactly equal to the difference in levels of the nuclear spin states. A suitable source of stimulating interaction can be discovered by close inspection of the atomic environment of the protons in tissue. For example, in water, each proton in a water molecule has a neighboring proton that is also a magnetic dipole which generates a magnetic field at the proton of about 5 G (0.5 mT) (Fig. 3.2). This field, however, is constantly changing in amplitude and direction as the water molecule rotates rapidly and moves about in the liquid. It also changes as a result of intermolecular collision, translation, or chemical dissociation and exchange. The magnetic field experienced by any nucleus will fluctuate with a frequency spectrum that is dependent on the rate of molecular tumbling due to the random thermal motion of the host and surrounding molecules (Fig. 3.3). The mean strength of the local field is determined by the strength of the magnetic dipoles in the medium and how close they approach to the hydrogen nuclei. Only the component of the frequency spectrum which is equal to the resonant frequency, ω0 (or, for reasons beyond our discussion, 2ω0), is effective in stimulating an energy exchange to induce transitions between nuclear spin states and lead to
thermal equilibrium, i.e., T1 relaxation. In liquids the characteristic frequencies of thermal motion are of the order of 1011 Hz or higher, much greater than NMR frequencies of 107 to 108 Hz. Consequently, the component of the frequency spectrum from molecular motion that can induce T1 relaxation is small and the process is slow. If the molecular motion becomes slower, for example, because of lower temperature, or increased molecular size, the intensity of the fluctuations of the magnetic field at the resonance frequency increases. As the motion becomes even slower, this intensity will at some point reach a maximum, but will then decrease again as the energy of the motion becomes increasingly concentrated in frequencies lower than the NMR range (when it is again not effective). Thus, T1 passes through a minimum value as the molecular motion becomes slower. The effect of the molecular motion is usually expressed by a correlation time, τc (see below, and Fig. 3.4), characteristic of the time of rotation of a molecule or of the time of its translation into a neighboring position. Relaxation rates in simple liquids are affected, for example, by viscosity, temperature, and the presence of dissolved ions and molecules, which alter the correlation times of molecular motion or the amplitudes of the dipolar interactions. In tissue, water relaxation times are shorter than in pure water because of interactions of the water molecules with macromolecules. Proteins and other macromolecular structures exert, via protons on their surfaces, dipolar fields that fluctuate relatively slowly and which are efficient sites for relaxation. The effect of these interactions with solute molecules is communicated to the rest of the water present in tissue through space or by processes of exchange and diffusion of water molecules and hydrogen atoms.
thermal equilibrium, i.e., T1 relaxation. In liquids the characteristic frequencies of thermal motion are of the order of 1011 Hz or higher, much greater than NMR frequencies of 107 to 108 Hz. Consequently, the component of the frequency spectrum from molecular motion that can induce T1 relaxation is small and the process is slow. If the molecular motion becomes slower, for example, because of lower temperature, or increased molecular size, the intensity of the fluctuations of the magnetic field at the resonance frequency increases. As the motion becomes even slower, this intensity will at some point reach a maximum, but will then decrease again as the energy of the motion becomes increasingly concentrated in frequencies lower than the NMR range (when it is again not effective). Thus, T1 passes through a minimum value as the molecular motion becomes slower. The effect of the molecular motion is usually expressed by a correlation time, τc (see below, and Fig. 3.4), characteristic of the time of rotation of a molecule or of the time of its translation into a neighboring position. Relaxation rates in simple liquids are affected, for example, by viscosity, temperature, and the presence of dissolved ions and molecules, which alter the correlation times of molecular motion or the amplitudes of the dipolar interactions. In tissue, water relaxation times are shorter than in pure water because of interactions of the water molecules with macromolecules. Proteins and other macromolecular structures exert, via protons on their surfaces, dipolar fields that fluctuate relatively slowly and which are efficient sites for relaxation. The effect of these interactions with solute molecules is communicated to the rest of the water present in tissue through space or by processes of exchange and diffusion of water molecules and hydrogen atoms.
 FIGURE 3.2 A dipole can be considered as two spherical foci of magnetic flux, separated in space. The resultant field pattern is similar to that from a thin bar magnet and falls off as the inverse third power of the distance. The magnetic field around a hydrogen nucleus varies in space, and the effect on a neighboring nucleus in the same molecule changes with time if the molecule moves. |
 FIGURE 3.3 The local magnetic field experienced by any proton varies as other nuclei or electrons that generate magnetic fields move past. |
Paramagnetic Relaxation
To design external agents that reduce T1 or T2 in tissues, we need a means of affecting the amplitude and time scale of variation of the local magnetic fields experienced by water molecules. Paramagnetic agents are effective contrast materials because they are able to do this. They are materials that on the atomic scale generate extremely strong local magnetic fields. Each agent possesses a large magnetic dipole moment compared to the proton. Transition and rare-earth metal ions are examples of these. The origin of their strong local fields lies in the fact that they contain unpaired electrons, viz., particles with spin and electric charge (and therefore a magnetic moment) that have not been “matched” (paired off) in a chemical bond with spins of opposite character, so that there is a net residual magnetic dipole moment. The electron spin magnetic dipole is 658 times greater than the proton (essentially because it has a smaller radius but the same charge), so any water molecules that approach close to an unpaired electron will experience an intense interaction that can promote relaxation. The observation that paramagnetics have a dramatic effect on the relaxation time of protons in solution has been well understood from the earliest work in NMR. For example, Bloch (1), in his 1946 article, stated that “it is recommendable to add to the substance a certain percentage of paramagnetic atoms or molecules to reduce T1,” in his case in order to reduce saturation effects and speed up his observations. With the advent of NMR imaging, it was quickly realized that paramagnetic agents could be used to provide enhanced image contrast. Lauterbur et al. (2) demonstrated the powerful effects of manganese ions in a canine model of myocardial infarction, and were able to show regional T1 effects in areas of the excised heart ex vivo. The first in vivo studies demonstrating contrast enhancement in MRI were performed by Gore et al. (3) who measured the time course of enhancement by manganese and other paramagnetic ions in rabbit tissues and demonstrated the feasibility of assessing organ uptake and excretion. They also performed the first human contrast studies by demonstrating the effects of oral ferrous ions on the signal from the GI tract (4).
A relatively complete theoretical description of nuclear relaxation, including a description of the effect of the dipole–dipole interaction between water molecules as well as the effects of paramagnetic ions, was given by Bloembergen, Purcell, and Pound in 1948 (5). They derived a simple approximate relation, since widely quoted in the radiological literature:
where ΔR1 is the increase in relaxation rate over pure solvent, N is the number of ions per cm3, μ is the magnetic moment of the ion, T is the temperature, γ is the gyromagnetic ratio of the proton, η the solution viscosity, and k is a physical constant.
This equation predicts that relaxation rate (the rate R1 = 1/T1) should increase in direct proportion to ion concentration. The predicted linearity between rate and concentration has been observed widely in both simple solutions and whole tissues for a variety of paramagnetic agents. However, the slope of the line, namely the rate increase per unit concentration of material or relaxivity, is found to depend not only on the choice of paramagnetic species, but also on the solvent and the nature
of other solutes, as well as on factors within the tissue. This relationship is further discussed below, but it emphasizes a fundamental difference between contrast agents for NMR and other modalities, namely in NMR the agent itself is not directly visualized, but rather the effect of the agent on the behavior of protons, which is not an intrinsic property of the agent alone. This is different to, for example, x-ray agents, whose effects on x-ray absorption are independent of their chemical form or changes in the environment. Equation 1 also predicts that the relaxation rate is proportional to the square of the magnetic moment. The magnetic moment is largely determined by the number of unpaired electron spins, and it is a prime factor that affects the materials’ magnetic susceptibility. The electron structures for several ions of potential interest are shown in Table 3.1. Table 3.2 shows the measured relaxation rates per unit concentration for various ions, normalized to the value for copper, along with the magnetic moment squared determined from susceptibility measurements. There is obviously a good correlation for several species, notably manganese and gadolinium, but there are severe discrepancies for many others. For example, most of the lanthanide rare-earth ions, typified by erbium, are at least an order of magnitude less effective as relaxation agents than would be predicted by considerations of magnetic moment alone. For example, dysprosium and holmium were considered in the early days of MRI as potential relaxation agents, but were shown to be quite ineffective at reducing T1 (6,7). This
discrepancy highlights the fact that Equation 1 is an inadequate description of paramagnetic effects even in simple aqueous solution. It ignores a fundamental requirement that was introduced earlier, viz., the time scale of the fluctuating magnetic field produced by the ion and experienced by the nuclei must match the frequency of the NMR transition to be affected. A more complete theoretical description was developed by Solomon (8) and by Bloembergen and Morgan (9,10). Recall that relaxation results from the action of fluctuating local magnetic fields experienced by protons, which stimulate the return to equilibrium of an excited population of spins. In water alone the dominant source of such effects is the dipole–dipole interaction between neighboring protons, mainly between hydrogen nuclei in the same water molecule. Molecular tumbling in Brownian motion causes the weak magnetic field produced by each proton to fluctuate randomly, and at the site of a neighboring proton these random alterations in the net field produce relaxation. T1 is sensitive mainly to the spectral component of such fluctuations at the Larmor frequency; T2 is sensitive also to low-frequency components, as described below. The time-scale characteristic of the dipolar interaction reflects molecular motion and clearly is expected to influence the efficacy of relaxation. Qualitatively, when there is a concentration of kinetic motion in the appropriate frequency range, relaxation will be efficient. We can envisage other types of motion which will be too rapid or too slow to be effective. An important descriptor is the correlation time, τc, which measures the time over which the local fluctuating field appears continuous and deterministic. It represents the time it takes on average for the field to change significantly. A more precise interpretation using simple statistical concepts is given in Figure 3.4.
of other solutes, as well as on factors within the tissue. This relationship is further discussed below, but it emphasizes a fundamental difference between contrast agents for NMR and other modalities, namely in NMR the agent itself is not directly visualized, but rather the effect of the agent on the behavior of protons, which is not an intrinsic property of the agent alone. This is different to, for example, x-ray agents, whose effects on x-ray absorption are independent of their chemical form or changes in the environment. Equation 1 also predicts that the relaxation rate is proportional to the square of the magnetic moment. The magnetic moment is largely determined by the number of unpaired electron spins, and it is a prime factor that affects the materials’ magnetic susceptibility. The electron structures for several ions of potential interest are shown in Table 3.1. Table 3.2 shows the measured relaxation rates per unit concentration for various ions, normalized to the value for copper, along with the magnetic moment squared determined from susceptibility measurements. There is obviously a good correlation for several species, notably manganese and gadolinium, but there are severe discrepancies for many others. For example, most of the lanthanide rare-earth ions, typified by erbium, are at least an order of magnitude less effective as relaxation agents than would be predicted by considerations of magnetic moment alone. For example, dysprosium and holmium were considered in the early days of MRI as potential relaxation agents, but were shown to be quite ineffective at reducing T1 (6,7). This
discrepancy highlights the fact that Equation 1 is an inadequate description of paramagnetic effects even in simple aqueous solution. It ignores a fundamental requirement that was introduced earlier, viz., the time scale of the fluctuating magnetic field produced by the ion and experienced by the nuclei must match the frequency of the NMR transition to be affected. A more complete theoretical description was developed by Solomon (8) and by Bloembergen and Morgan (9,10). Recall that relaxation results from the action of fluctuating local magnetic fields experienced by protons, which stimulate the return to equilibrium of an excited population of spins. In water alone the dominant source of such effects is the dipole–dipole interaction between neighboring protons, mainly between hydrogen nuclei in the same water molecule. Molecular tumbling in Brownian motion causes the weak magnetic field produced by each proton to fluctuate randomly, and at the site of a neighboring proton these random alterations in the net field produce relaxation. T1 is sensitive mainly to the spectral component of such fluctuations at the Larmor frequency; T2 is sensitive also to low-frequency components, as described below. The time-scale characteristic of the dipolar interaction reflects molecular motion and clearly is expected to influence the efficacy of relaxation. Qualitatively, when there is a concentration of kinetic motion in the appropriate frequency range, relaxation will be efficient. We can envisage other types of motion which will be too rapid or too slow to be effective. An important descriptor is the correlation time, τc, which measures the time over which the local fluctuating field appears continuous and deterministic. It represents the time it takes on average for the field to change significantly. A more precise interpretation using simple statistical concepts is given in Figure 3.4.
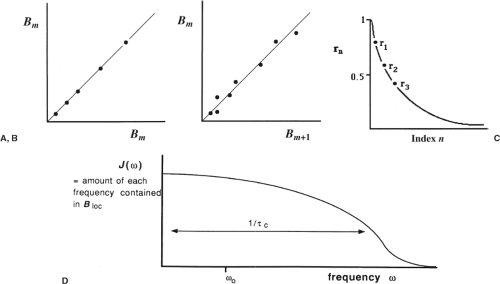 FIGURE 3.4 The meaning of correlation times. Many processes are neither purely random nor deterministic, but show traits both of ordered and stochastic natures. For example, if a fast movie were to record the positions of all the water molecules in a sample of liquid, starting with them each individually labeled, it would show them all moving randomly with time so that after an interval the molecular arrangement (the pattern of the labels) would bear no relation to the starting picture. However, it would take a finite time for one random arrangement to convert to another without there being an apparent evolution, so that successive “still” frames from the movie would show an apparent causal relationship that grows weaker with the length of the intervening time. The period or interval for this apparent causality to disappear is the correlation time. A: For the local fluctuating magnetic field experienced by a proton the correlation time can be given a precise mathematical interpretation using familiar (hopefully) statistical concepts. Consider recording the local field, B(t), over some time interval 0 < t < T, by taking discrete measurements at regular sampling times mΔt, where m varies from 1 to a large number. These samples are called Bm. If we plot the numbers Bm against themselves, we obviously obtain a perfect straight line relationship. The x and y variables are the same set of numbers, so they are perfectly correlated. Mathematically, we can compute the correlation coefficient for the linear regression, r0, which in this case is exactly 1. B: We can then plot a second graph, of Bm against Bm+1, viz., the x axis variables are also Bm samples, but shifted 1 place in order of measurement, as though column 2 of a table had slipped one row relative to column 1. If B(t) does not vary too rapidly in the sample interval Δt the plot of Bm vs. Bm+1 will be scattered about the line of regression to a degree that depends on how much change occurs. We can then recompute the correlation coefficient, r1, which will be less than 1. Repeating this procedure we can compute r2 for the line Bm vs. Bm+2, and so on. For a field modulated by random molecular motions, r0 > r1 > r2. We can further plot the value of rn obtained for each shift of the data, i.e., rn vs. n. C: The rate that rn decays with n measures the interval over which a sample of waveform of the field appears different, or unconnected, with a corresponding sample taken later. The width of the plot of rn vs. n (which is termed the autocorrelation function) is the correlation time in units of Δt. For completely random rapidly fluctuating fields, it is short, but for systems in which it takes a relatively long time for a molecule to tumble it may be much longer (see text). D: An alternate yet equivalent description of the fluctuating local field is the spectral density. The local field can be considered to comprise a mixture of component frequencies, a frequency spectrum. The amount at any frequency ω is denoted by J(ω0), the spectral density. If the autocorrelation function (Fig. 3.4C) is of the form  |
TABLE 3.1 Electron Structures of Elements | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
Paramagnetic ions accelerate relaxation by mechanisms similar to those that account for normal water relaxation. Since the relaxation rate depends on the magnetic moment squared, and the electron moment is 658 that of the proton, we expect that roughly 2.5 mmol of unpaired electrons will double the relaxation rate of water (110-molar protons), all other factors being equal. Although, in practice, many agents are more effective than this lower limit, it is instructive to note that such concentrations are several orders of magnitude greater than the levels typically detected in radionuclide counting experiments. This is particularly important to realize in contemplating the possibility of labeling target-specific carrier molecules or designing other molecular probes. Paramagnetic ions in simple solutions most effectively relax the protons in water molecules in close proximity, in the first monolayer of molecules around the ion, as discussed further below. In addition, there is a weaker effect on molecules in outer spheres, which may, nonetheless, be effectively relaxed. If R1 and R2 denote the relaxation rates of protons in the coordination sphere of an aquoion, and these are rapidly exchanging position and continuously diffusing through the solvent water (intrinsic relaxation rate Rw), the overall rates will be a weighted average, R′1,2:
where u is the fraction of time each proton spends in the coordination shell. Note that this assumes all the water has access to the inner shell of an ion. This relation will not hold when water
compartments are restricted, which can be important in vivo as discussed below.
compartments are restricted, which can be important in vivo as discussed below.
TABLE 3.2 Comparison of Measured Relaxation Rate Enhancement at 20 MHz with Magnetic Moments, μ, Both Normalized to the Value for Copper | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
If the coordination number of the ion is q, and M and N denote the molar concentrations of the metal ion and water molecules, respectively, then:
where ΔR1,2 are the relaxivities, or increases in relaxation rates over the solvent per unit concentrations of metal. The relaxation rates R1,2 of protons in the coordination sphere are predicted by the Solomon–Bloembergen–Morgan (SBM) equations (10):
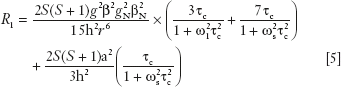
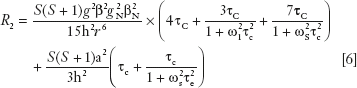
where ω1, ωs are the Larmor frequencies for proton and electron spins, respectively (which depend on the magnetic field strength), βN is the nuclear magneton, β is the Bohr magneton, g is the electronic g factor, r is the ion–nucleus distance, a is the hyperfine coupling constant, S is the electron spin quantum number, and gN is the proton g factor.
At first sight these equations may appear daunting but they are actually quite simple to interpret. They state that the relaxation rates for each paramagnetic species depend on the values of several physical constants and four variable quantities: first, the magnetic moment of the paramagnetic species (via S); second, the distance between the paramagnetic center and the nucleus being relaxed (r); third, the frequency of the NMR measurement; and fourth, the correlation time that describes the rate of variation of the local field.
The first terms in brackets on the right-hand side describe the dipolar electron–nucleon interaction. This arises for all paramagnetics because there is a magnetic moment associated with the electron spin that has a spatial dipole dependence similar to that of the proton in Figure 3.2. This is sometimes referred to as a “through-space” coupling. The second terms describe a so-called scalar or contact hyperfine interaction, which is important only for some paramagnetic agents, and which involves spin exchange between the electron and nucleus, and is mediated by the formation of a transient chemical bond (a so-called through-bond interaction). The correlation times characteristics of these two interactions are different and denoted as τc and τe, respectively. τc itself is determined by the combined effects of three processes: rotation, electron spin relaxation, and proton exchange. Rotation alters the orientation of the proton relative to the magnetic field of the electrons. The electron spin relaxation time refers to the time which elapses on average before the electron spin itself alters its orientation. Proton exchange denotes the process whereby water molecules enter and depart the hydration sphere of the paramagnetic center. Since these processes are independent, their rates are additive so that
where τR is the rotational correlation time for the ion and its hydration complex, τM is the exchange correlation time, the average time a proton stays in the hydration sphere of an ion; and τS is the electron spin relaxation time. Similarly,
Equation 7 states that the effective correlation time is dominated by the fastest molecular process which affects it. Qualitatively, if the electron spins maintain their orientation during the course of Brownian motion and diffusion, relaxation will be primarily dependent on the rate at which water molecules randomly alter their position and orientation in the vicinity of the ions (τR ≪ τS). Alternatively, if the electron spin changes its direction frequently, perhaps because of variations in its magnetic environment due to variations in the detailed atomic configuration (τS ≪ τR), the magnetic field at the neighboring proton will fluctuate more rapidly and be less effective at increasing the relaxation rate. In aquo, τM is usually relatively long and can often be neglected, though it may be advantageous to modify the water bonding to compounds to ensure exchange is not too rapid.
The SBM description verifies that relaxation rate is proportional to the second power of magnetic moment, since
However, the SBM equations show a dependence on three factors in addition to magnetic moment. These are (a) correlation time, (b) frequency, and (c) distance.
Correlation Time
The dependence on correlation time underlines the fact that the local magnetic field must fluctuate neither too slowly nor too rapidly for effective relaxation. The local field, as seen in Figure 3.3, does not simply oscillate at a single frequency, but instead produces a magnetic perturbation that can be decomposed into a range of component frequencies (by the process called Fourier analysis). The constituent range of frequencies is called the power spectrum of the field, or the spectral density. NMR imaging frequencies are relatively low compared to other atomic rates such as water molecule rotations. Materials for which the correlation time is very short spread the spectral energy over a very wide range of frequencies and, consequently, the amplitude in a narrow frequency range, particularly at a low NMR frequency, is actually quite low. On the other hand, agents with a longer correlation time, where the interaction is varying more slowly, tend to have more energy concentrated in the low frequency range which is relevant to the NMR frequency. Thus we can predict that materials with too short a correlation time will in fact be quite ineffective as relaxation agents whereas agents with a longer correlation time will be more effective. There is an optimal range of τc. Figure 3.5A shows this graphically and it may be seen that there is a dramatic increase in the relaxation efficiency as we increase the correlation time. To produce strong relaxation effects, the paramagnetic species should have a reasonably long τc, and thus long τR and τS. The major discrepancies of the expected values of T1 in Table 3.1 are explicable because most of the lanthanides, cobalt, and nickel have short electron spin relaxation times (τR = 10−13 seconds). Thus, the local fields they generate fluctuate too rapidly to be effective. Manganese and gadolinium have relatively long τR (10−10 seconds) and thus are more effective. Furthermore, the dominant correlation time is the rotational correlation time, τR. This dominance imparts the characteristic that if τR can be lengthened, the agent will be even more effective. In particular, for the regime ωIτc ≪ 1, if the scalar contribution can be ignored (see below), Equation 5 reduces to:
and increasing τR (=τc) will improve relaxivity. Such an effect may be dramatically realized when the paramagnetic species binds to a larger molecule, so that it tumbles at a slower rate or has its motion restricted; and thereby is characterized by a larger reorientation time constant. The result is a so-called proton relaxation enhancement as first described by Eisinger et al. (11). Figure 3.6 demonstrates the effect of a paramagnetic ion (manganese) binding to metal-binding sites on bovine serum albumin and γ-globulin. The amount of metal required to shorten the solution relaxation times is much less in the presence of the macromolecules than in free solution. The behavior in the presence of the solute can be described in terms of an enhancement ratio, ε:
 FIGURE 3.5 A. The dependence of the inner sphere longitudinal relaxation rate, 1/T1, as a function of correlation time τc, as predicted by the SBM equations, assuming the hyperfine contribution is negligible. This curve varies with the NMR frequency. B: The variation of the inner sphere longitudinal relaxation rate 1/T1 as a function of frequency (in MHz) for different values of the rotational correlation time for gadolinium, assuming S = 7/2, q = 1, r = 3.1 A°, τM = 3 × 10−9 s, τs = 10−10 s. |
 FIGURE 3.6 Relaxation rate of Mn-doped albumin (solid triangles) and γ-globulin (open triangles) solutions at 4.5% concentration and pH 7.7 vs. Mn concentration. The rate for Mn solutions (circles) is also shown for comparison. (From Kang YS, Gore JC, Armitage IM. Studies of factors affecting the design of NMR contrast agents: manganese in blood as a model system. Magn Reson Med 1984;1:396–409, with permission.) |
For the Mn–albumin system ε is around 10 at 20 MHz, pH = 7.0 (12). For manganese introduced intravenously in whole tissues, ε is typically 5 at 20 MHz (13), showing that significant enhancements are achievable in vivo by binding effects of ions to proteins, membranes, and other high–molecular-weight structures. Similar properties are displayed by gadolinium. For example, Reuben (14) demonstrated enhancements of around 5 for Gd bound to BSA at 24.3 MHz, while Burton et al. (15) recorded enhancements of around 8 at 40 MHz for gadolinium bound to immunoglobulin-G, with τR = 10−8 seconds. Lauffer et al. have more recently estimated enhancements of up to 10 for Gd bound via DTPA or EDTA to IgG and BSA (16).
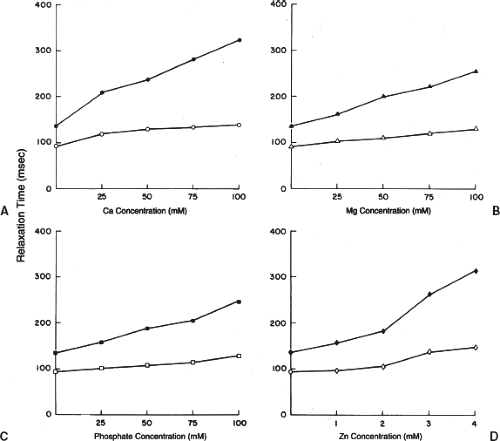
Binding effects producing enhancements are important factors in determining the relaxation behavior of some paramagnetic species, so variations in the chemical equilibrium that may displace bound ions can have a subtle influence on proton relaxation measurements. Thus, alterations in hydrogen ion concentration (pH) or the presence of other competing ions (e.g., calcium, phosphate, or other metals) have been shown to affect the enhancement ratio and relaxation times of solutions of free metal and protein. When these competing ions are abundant, the association of the metal with the protein decreases, and bound metal ions are released to free solution, thereby becoming less effective by tumbling more rapidly (Fig. 3.7).
Enhancement effects are of major interest in the design of paramagnetic delivery methods. For example, proteins and polyamino acids, liposomes, or monoclonal antibodies of high molecular weight, can provide a suitable tumbling vehicle to lengthen the correlation time of bound gadolinium to 10−8 seconds, and thereby provide optimal relaxivity. Some examples of this behavior are described below. Furthermore, as suggested by Lauffer (17), in vivo enhancements may be obtainable by distributing agents in a tissue compartment with high microviscosity, or by forming noncovalent bonds with macromolecules in tissue. Gd-DTPA does not localize intracellularly, and so may not benefit from interacting with the
more viscous cytosol. However, there have been few quantitative measurements of the in vivo relaxivity of clinical agents (since this requires knowing the tissue concentration of the metal) and the issue of whether Gd chelates are more or less effective in reducing tissue water rates as when studied ex vivo requires further clarification.
more viscous cytosol. However, there have been few quantitative measurements of the in vivo relaxivity of clinical agents (since this requires knowing the tissue concentration of the metal) and the issue of whether Gd chelates are more or less effective in reducing tissue water rates as when studied ex vivo requires further clarification.
 FIGURE 3.7 Relaxation times T1 (solid symbols) and T2 (open symbols) of 4.5 g% albumin–70 μM Mn solutions at pH 7.7 titrated with Ca (A), Mg (B), phosphate (C), and zinc (D). Ionic strength was maintained at 0.3 with NaCl. (From Kang YS, Gore JC, Armitage IM. Studies of factors affecting the design of NMR contrast agents: manganese in blood as a model system. Magn Reson Med 1984;1:396–409, with permission.) |
A secondary consequence of lengthening τR is that such a change does not affect τe (Equation 8) and so does not influence the contact contribution to relaxation. It can be inferred from Table 3.2 and the SBM equations that the contact term accounts for much of the spin–spin relaxation of free manganese solutions, for which T1/T2 is typically 5 (in most other solutions of free ions, T1 and T2 are approximately equal). Increasing τR, therefore, has a less significant effect on T2 than on T1. Thus, as τR increases, T1/T2 decreases toward 1. The ratio T1/T2 is a sensitive index of the binding kinetics of a metal with a significant contact contribution (12).
Related posts:
Stay updated, free articles. Join our Telegram channel
Full access? Get Clinical Tree
