([1])
where N m is the number of spins in the state m, N 0 is the total number of spins, E m is the energy of the state m, T is the absolute temperature, and k B is Boltzmann’s constant. E m is given by
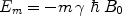
([2])
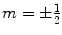 , γ is the gyromagnetic ratio for the nucleus in question, and ħ is the reduced Planck’s constant. Nuclei with spin quantum number
, γ is the gyromagnetic ratio for the nucleus in question, and ħ is the reduced Planck’s constant. Nuclei with spin quantum number 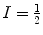 (e.g., 1H, 3He, 13C, 129Xe) have two eigenstates in a magnetic field. The difference in population between the two possible energy states is described by the polarization, P, and it is defined accordingly:
(e.g., 1H, 3He, 13C, 129Xe) have two eigenstates in a magnetic field. The difference in population between the two possible energy states is described by the polarization, P, and it is defined accordingly: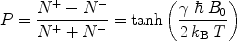
([3])
For experiments performed at room temperature and with the highest magnetic field available, E m is small compared to k B T and Eq. [3] can be reduced to:


([4])
From these expressions, the polarization for protons in a 1.5 T MR scanner becomes ∼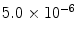 and the polarization for 13C is only ∼
and the polarization for 13C is only ∼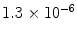 . The polarization may therefore be enhanced 200,000 and 800,000 times respectively if polarization of unity can be achieved.
. The polarization may therefore be enhanced 200,000 and 800,000 times respectively if polarization of unity can be achieved.
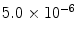 and the polarization for 13C is only ∼
and the polarization for 13C is only ∼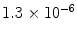 . The polarization may therefore be enhanced 200,000 and 800,000 times respectively if polarization of unity can be achieved.
. The polarization may therefore be enhanced 200,000 and 800,000 times respectively if polarization of unity can be achieved.In an MR scanner operating at field strength of a few megahertz or higher, the dominating source of noise is the imaged object (e.g., the patient) and the signal-to-noise ratio, SNR, may be expressed as (1)
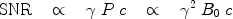
where c is the concentration of the nucleus in question. The polarization, and thereby the strength of the NMR signal, increases proportionally with the magnetic field (1), which has been the motivation for developing higher field MR systems. The concentration of a hyperpolarized imaging agent may be 0.5 M in the injection syringe, but will decrease to 1–20 mM in vivo due to dilution in the vascular system. In hyperpolarized metabolic mapping applications, where the signals from intracellular metabolites are being visualized, the available concentration may be one to two orders of magnitude lower. This should be compared to the proton concentration of ∼80 M in the body.
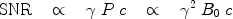
([5])
The term hyperpolarization is used to indicate that the available polarization is no longer determined by the main magnetic field of the MR scanner. Instead it is higher than the thermal equilibrium polarization given by Eq. [4]. The polarization for a hyperpolarized agent is created outside the imaging system by means of a polarizer. The hyperpolarization method can be based on several principles (2–6) of which two have successfully been applied to molecules in solution: para-hydrogen induced polarization (PHIP) and dynamic nuclear polarization (DNP) followed by dissolution.
The right-hand side of Eq. [5] is not valid for hyperpolarized molecules; instead the middle part of the expression should be used: the image quality is no longer a function of B 0. However, the choice of nucleus will still influence the SNR through the gyromagnetic ratio.
2 Dynamic Nuclear Polarization (DNP)
2.1 Introduction
In recent years, a novel method for polarizing nuclear spins in molecules in solution has been developed. The method takes advantage of dynamic nuclear polarization (DNP) in the solid state followed by rapid dissolution in a suitable solvent (6–8). The polarization is retained almost completely in the dissolution step creating a solution with a non-thermal nuclear polarization approaching unity.
DNP was first described theoretically by Overhauser in 1953 (9) and a few months later demonstrated by Carver and Slichter (10) in metallic lithium. Overhauser predicted that saturating the conduction electrons of a metal would lead to a dynamic polarization of the nuclear spins. This was a fundamental discovery causing disbelief at the time: that heating of one spin system could lead to the cooling of another. The prediction by Overhauser for metals was soon extended to electron spins in solution by Abragam (11) and most NMR spectroscopists are today familiar with the nuclear and electronic Overhauser effect. However, this effect is limited to solutions where relaxation processes couple the spin systems via the lattice (thermal motions). Soon after, the solid effect was described for spins in the solid state coupled by dipolar interactions (12). Later, DNP in the solid state was extended mechanistically to processes involving several electron spins and thermal mixing (13). The theory of DNP in the solid state, however, has failed to provide a quantitative description in general. In the solid state, the high electron spin polarization is in part transferred to the nuclear spins by microwave irradiation close to the resonance frequency of the electron spin. The efficiency of this process depends on several parameters characterizing the various spin systems, but also on technical factors such as microwave frequency and power.
DNP has mainly been applied to the generation of polarized targets for neutron scattering experiments and it has been demonstrated that the nuclear polarizations of 1H and 13C could be increased to almost 100% and to ∼50%, respectively in the solid state by means of DNP at low temperature (14, 15). The mechanism requires the presence of unpaired electrons, which are added to the sample as, for example, an organic radical. The magnetic moment of the electron is 658 times higher than that of the proton. This means that the electron spin will reach polarization of unity at a moderate magnetic field strength and liquid helium temperature. Equation [3] gives an electron spin polarization of 98% at 3.35 T and 1 K. The efficiency of the transfer of polarization from the electron to the nuclear spins depends on several factors.
2.2 DNP Sample Preparation
The formulation of a new compound as a hyperpolarized imaging agent with the DNP method imposes several challenges. In order for the DNP process to be effective, the electron paramagnetic agent must be homogeneously distributed within the sample. Many compounds will be crystalline and have a tendency to crystallize as saturated aqueous solutions. This typically leads to poor polarization. To prevent crystallization and to produce an amorphous solid upon cooling of the sample, glass formers such as glycerol or DMSO can be added to the mixture of the compound and the electron paramagnetic agent. Since it is often a requirement for in vivo studies to achieve a high concentration of the compound after dissolution, it is necessary to be able to formulate the compound in a concentrated form. A solvent mixture with high solubility for the compound and good glassing properties is therefore chosen. Secondly, the solvent mixture has to be either biologically compatible or removed from the solution after polarization.
2.3 Electron Paramagnetic Agent
The source of the unpaired electron is typically an organic radical, but a few metal ions have been employed successfully for DNP, Cr(V) in particular (14–16). The choice of electron paramagnetic agent will depend on a number of factors. First, the electron paramagnetic agent needs to be chemically stable and dissolve readily in the matrix of interest. Second, the electron paramagnetic resonance (EPR) spectrum of the radical should have a width that allows DNP to be effective for the nucleus of interest, i.e., a linewidth that exceeds the Larmor frequency of the nuclear spin. In practice, the above criteria means that two classes of electron paramagnetic agents are available, namely, nitroxides (17, 18) and trityls (19–21). The nitroxides belong to a class of molecules that have been studied extensively by EPR, and which have been used for DNP in many systems. Nitroxides are characterized by having a broad EPR spectrum  (16), covering the Larmor frequency of all nuclear spins. Some of them have reasonable chemical stability and come with different degrees of hydrophilicity. Another class of electron paramagnetic agents with superior properties for direct polarization of low-γ nuclei such as 13C, 15N, and 2H is the trityls
(16), covering the Larmor frequency of all nuclear spins. Some of them have reasonable chemical stability and come with different degrees of hydrophilicity. Another class of electron paramagnetic agents with superior properties for direct polarization of low-γ nuclei such as 13C, 15N, and 2H is the trityls  (16, 22, 23). The trityls also exist with a range of hydrophilicities and some of them are chemically very stable. It was recently discovered that gadolinium can positively affect the solid-state DNP enhancement (24). Other paramagnetic ions and molecules (Mn2+ and O2) can in part have the same effect. The physics is not understood, but Hu et. al. (23) have shown that the longitudinal relaxation time of the electron paramagnetic agent is shortened by the presence of the Gd ions. The effect of adding 1–2 mmol/L Gd3+ is a 50–100% improvement of the DNP. The effect seems to be general to most samples, but has to be optimized for each sample similarly to the concentration of the electron paramagnetic agent. There is no direct DNP effect of the Gd3+ by itself. Finally, Gd3+ may enhance the solid-state polarization by DNP, but care should be taken in eliminating relaxation in the liquid state. Free Gd-ions would cause detrimental liquid-state relaxation and pose an in vivo safety risk.
(16, 22, 23). The trityls also exist with a range of hydrophilicities and some of them are chemically very stable. It was recently discovered that gadolinium can positively affect the solid-state DNP enhancement (24). Other paramagnetic ions and molecules (Mn2+ and O2) can in part have the same effect. The physics is not understood, but Hu et. al. (23) have shown that the longitudinal relaxation time of the electron paramagnetic agent is shortened by the presence of the Gd ions. The effect of adding 1–2 mmol/L Gd3+ is a 50–100% improvement of the DNP. The effect seems to be general to most samples, but has to be optimized for each sample similarly to the concentration of the electron paramagnetic agent. There is no direct DNP effect of the Gd3+ by itself. Finally, Gd3+ may enhance the solid-state polarization by DNP, but care should be taken in eliminating relaxation in the liquid state. Free Gd-ions would cause detrimental liquid-state relaxation and pose an in vivo safety risk.
 (16), covering the Larmor frequency of all nuclear spins. Some of them have reasonable chemical stability and come with different degrees of hydrophilicity. Another class of electron paramagnetic agents with superior properties for direct polarization of low-γ nuclei such as 13C, 15N, and 2H is the trityls
(16), covering the Larmor frequency of all nuclear spins. Some of them have reasonable chemical stability and come with different degrees of hydrophilicity. Another class of electron paramagnetic agents with superior properties for direct polarization of low-γ nuclei such as 13C, 15N, and 2H is the trityls  (16, 22, 23). The trityls also exist with a range of hydrophilicities and some of them are chemically very stable. It was recently discovered that gadolinium can positively affect the solid-state DNP enhancement (24). Other paramagnetic ions and molecules (Mn2+ and O2) can in part have the same effect. The physics is not understood, but Hu et. al. (23) have shown that the longitudinal relaxation time of the electron paramagnetic agent is shortened by the presence of the Gd ions. The effect of adding 1–2 mmol/L Gd3+ is a 50–100% improvement of the DNP. The effect seems to be general to most samples, but has to be optimized for each sample similarly to the concentration of the electron paramagnetic agent. There is no direct DNP effect of the Gd3+ by itself. Finally, Gd3+ may enhance the solid-state polarization by DNP, but care should be taken in eliminating relaxation in the liquid state. Free Gd-ions would cause detrimental liquid-state relaxation and pose an in vivo safety risk.
(16, 22, 23). The trityls also exist with a range of hydrophilicities and some of them are chemically very stable. It was recently discovered that gadolinium can positively affect the solid-state DNP enhancement (24). Other paramagnetic ions and molecules (Mn2+ and O2) can in part have the same effect. The physics is not understood, but Hu et. al. (23) have shown that the longitudinal relaxation time of the electron paramagnetic agent is shortened by the presence of the Gd ions. The effect of adding 1–2 mmol/L Gd3+ is a 50–100% improvement of the DNP. The effect seems to be general to most samples, but has to be optimized for each sample similarly to the concentration of the electron paramagnetic agent. There is no direct DNP effect of the Gd3+ by itself. Finally, Gd3+ may enhance the solid-state polarization by DNP, but care should be taken in eliminating relaxation in the liquid state. Free Gd-ions would cause detrimental liquid-state relaxation and pose an in vivo safety risk.2.4 DNP Instrumentation
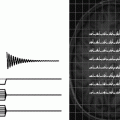
The method and instrumentation for hyperpolarization by DNP was first described by Ardenkjaer-Larsen et al. (6). The polarizer, which is depicted in Fig. 1, is built to a large extent on principles established in the polarized target community. Most solid-state DNP has been performed at magnetic fields between 0.35 T (25) and 16.5 T (26, 27), and at temperatures from below 1 K to room temperature. At temperatures below a few kelvin and magnetic field strengths above a few tesla, electron spins are almost fully polarized, and large nuclear polarizations can be obtained. Unlike in NMR spectroscopy applications where non-equilibrium polarizations can be regenerated by repeating the microwave irradiation and NMR acquisition (26), the polarization generated for in vivo applications will decay irreversibly after dissolution. Hence, the goal is to generate polarizations close to unity. It is therefore important to choose initial operating conditions that have been proven to provide high nuclear polarization, but, at the same time, that are easily achievable using standard instrumentation. Temperatures of ∼1 K can be achieved by pumping in liquid helium. In the original work, the liquid helium was supplied to the sample space through a needle valve from the magnet cryostat, but in a recent publication, an alternative arrangement that used a separate helium dewar was described (28). A magnetic field strength of 3.35 T was chosen since microwave sources are readily available at 94 GHz for irradiation of the electron spin. However, recently it has been demonstrated that for both nitroxides and trityls a significant improvement in polarization can be obtained by increasing the magnetic field strength (29, 30) or lowering the temperature (31). For the compound [1-13C]pyruvic acid the 13C polarization improved from 27% at 3.35 T to 60% at 4.64 T in the solid state. Typically, the literature has been limited and disagreeing on the magnetic field dependence of DNP (32). On the other hand, it has been well established that lowering the temperature is beneficial for DNP. Another recent development in DNP instrumentation for in vivo applications is the closed-cycle sorption pump cryostat (31). This system achieves a base temperature of less than 0.8 K for a pumped 4He bath.
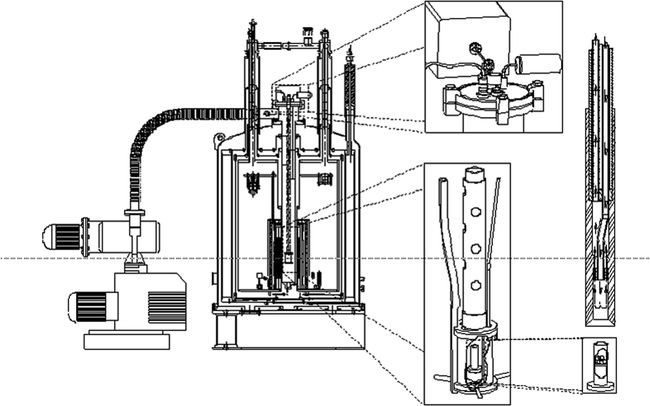

Fig. 1.
DNP prototype polarizer design and principle as used in most hyperpolarization experiments for in vivo studies. A superconducting magnet charged to 3.35 T, mechanical pumps (left-hand side) to reduce the temperature of liquid helium to ∼1.2 K, a microwave source to irradiate the sample at ∼94 GHz, and to the right the dissolution stick, which is introduced at the end of the experiment to dissolve the frozen material in the sample container in lower right corner. Reprinted with permission of John Wiley & Sons, Inc. (6).
2.5 Dissolution
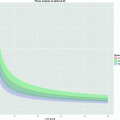
Once adequate solid-state polarization has been obtained, the sample needs to be dissolved in a suitable buffer. The dissolution may involve neutralization of the agent with acid or base, depending on the solid sample preparation. Buffering of the solution may be required to maintain control of pH and achieve a physiologically acceptable formulation. Physiological buffers such as tris(hydroxymethyl)aminomethane(TRIS) or 4-(2-hydroxyethyl)piperazine-1-ethanesulfonate (HEPES) are commonly used. The dissolution has to be efficient and fast compared to nuclear T 1 in order to preserve the nuclear polarization in this process. Formulating the solid sample as beads or powder may improve the dissolution (in terms of polarization and recovery of the solid sample), but understanding and optimizing the fluid dynamics (33) as well as providing the necessary heat is essential for optimal performance of more difficult agents. Relaxation during the dissolution process can depend on several factors. To minimize relaxation, dissolution is performed in the high field of the polarizer (e.g., 3 T in the case of a 3.35 T polarizer), but above the liquid helium surface. Any paramagnetic ions that could increase the relaxation rate are chelated by adding, for example, EDTA to the dissolution medium. To illustrate the severity of relaxation during dissolution [1-13C]pyruvic acid is chosen as an example. This molecule has been well studied with DNP and has high biological relevance. We assume a best case, i.e., relaxation by paramagnetic impurities is eliminated by having pure samples or chelating any remaining impurities. Chemical shift anisotropy is negligible due to the moderate field of the polarizer. Dipolar relaxation to the protons is unavoidable unless the sample is partially deuterated. In Fig. 2 the T 1 of the C-1 of neat [1-13C]pyruvic acid at 9.4 T is given as a function of temperature (unpublished data). It can be seen that the minimum T 1 is ∼1.6 s. With the trityl radical present (20 mmol/L), there will be an additional (dipolar) relaxation contribution. It can be seen that the contribution from the trityl is marginal and shifts the minimum to a different temperature (correlation time). The values in Fig. 2 agree well with theory based on the known distances between the spins. According to dipolar relaxation theory the minimum T 1 scales approximately as B 0, which means that a minimum T 1 of 0.7 s should be expected during the dissolution (in the 3 T polarizer field). The data illustrates that the nuclear spin during the dissolution should pass through this T 1 minimum on a much faster timescale to avoid a loss of polarization. The example illustrates that it is not unreasonable to expect that the loss of relaxation during dissolution can be overcome. The severity of the problem will depend on the target spin and sample properties, but several parameters can be controlled, e.g., the distance to other spins (labeling position), the abundance of other spins (full or partial deuteration), and the concentration of the electron paramagnetic agent (sacrificing DNP for longer T 1).
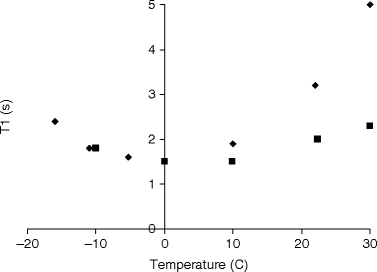

Fig. 2.
Longitudinal relaxation time, T 1, as a function of temperature for (solid diamond) neat [1-13C]pyruvic acid and (solid square) [1-13C]pyruvic acid with 20 mmol/L trityl.
Finally, the solution may undergo a filtration or chromatography step to remove the electron paramagnetic agent involved in the DNP process. In case a Gd chelate has been added, this agent may be removed as well. In most cases, the electron paramagnetic agent or Gd chelate do not cause significant relaxation after dissolution and may also be safe to inject into animals, but can often simply be removed by ion exchange or reverse phase chromatography. The filtration can either be in-line with the dissolution process or a subsequent step. In either case, the filtration is completed in a matter of a few seconds with insignificant loss of polarization or target molecule (unpublished work). However, significant research into optimal filtration materials will be required for new combinations of agents.
2.6 Nuclei and Compounds
Many nuclei have been hyperpolarized by the DNP method: 1H (34), 6Li (35), 19F (36), 13C, 15N (37), 31P (38), 89Y (39), and 129Xe (40). Nuclei with spin greater than ½ other than 6Li will have too short relaxation times and are unattractive for hyperpolarization studies in vivo. High gyromagnetic ratio nuclei such as 1H, 19F, and 31P will also typically have relatively short T 1. The obvious nucleus of choice is 13C, since many cellular metabolites can be labeled at positions in which the nucleus has a long relaxation time. The signal (polarization) that is created in the polarization process decays to thermal equilibrium with a rate (relaxation time) that depends on a number of factors. Some factors can be controlled in the dissolution process e.g., temperature, magnetic field, pH, and paramagnetic impurities. Others, such as intramolecular dipolar, chemical shift and spin rotation contributions depend on the 13C position in a specific molecule and cannot be controlled as easily (with the possible exception of deuteration in some cases to avoid 13C–1H dipolar relaxation).
The range of compounds that have been polarized by DNP is extensive and demonstrates the generality of the method: [13C]urea (6), [1-13C]pyruvate (41), [2-13C]pyruvate (42), [1-13C]lactate (43), [13C]bicarbonate (44), [1,4-13C2]fumarate (45), [1-13C]acetate (46), [5-13C]glutamine (47), [1-13C]glutamate (48), amino acids in general (49), [1,1-13C]acetic anhydride (50), and [2-13C]fructose (51). The choice of compound is motivated not only by formulation and relaxation considerations but also by the imaging application; examples of which will be reviewed in Chapter 33. The list above is not exhaustive, but illustrates the versatility of the DNP dissolution method. The listed compounds have been imaged in vivo and are examples of potentially useful biomarkers for the hyperpolarized MR.
2.7 Summary
The hyperpolarization of small organic molecules by DNP and dissolution for use as MR agents have been successfully demonstrated for a range of compounds of biological interest. Whereas pre-clinical research applications are now pursued at a number of academic institutions, the development of the technology to clinical routine application is a challenge. Partly, this is due to the need for a relatively complex instrumentation in a hospital environment, but also due to the fact that pharmaceutical requirements of sterility and quality control need to be assured.
3 PHIP
Hydrogenation of an organic molecule with para-hydrogen creates a highly ordered spin state which results in the observation of large anti-phase NMR signals. This was discovered, both theoretically and experimentally, by Bower and Weitekamp in 1986 (52, 53). This phenomenon was initially given the names of PASADENA (53) and ALTADENA (54) for two different implementations, using respectively either a high or low magnetic field during hydrogenation. A more widespread acronym, relating to all different implementations of this phenomenon, PHIP is derived from “para-hydrogen induced polarization.” PHIP can be used for magnetic resonance imaging (5, 55–60), where different methods have been developed for transforming the initial spin order into a net polarization. No net polarization is created from the hydrogenation step itself, although large NMR peaks are observed. A further treatment of the system is necessary for conversion into polarization suitable for imaging.
3.1 Properties of Para-hydrogen
The nuclear spin states of the hydrogen molecule exist in two varieties; a triplet state of total spin S = 1 called ortho-hydrogen, and a singlet state of total spin S = 0 called para-hydrogen. The spin states of ortho- and para-hydrogen are symmetric and anti-symmetric respectively, and are given by
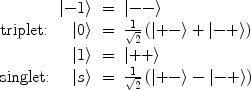 where + and – refer to the sign of the two protons spin values along a given axis, i.e., “spin up” and “spin down.”
where + and – refer to the sign of the two protons spin values along a given axis, i.e., “spin up” and “spin down.”
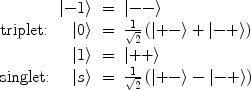
According to the Pauli principle, the total wave function of two identical fermions must be anti-symmetric. The implication of this for the hydrogen molecule is that symmetric rotational quantum states with even rotational quantum numbers are only allowed for the nuclear singlet state and that the anti-symmetric rotational quantum states with odd quantum numbers are only allowed for the triplet states. In the high-temperature limit all four nuclear states are equally populated resulting in ¼ of the states being para-hydrogen, whereas in the low temperature limit only the ground state, the singlet state with J = 0, is populated and we have 100% para-hydrogen. For intermediate temperatures the fraction of para-hydrogen at thermal equilibrium is given by
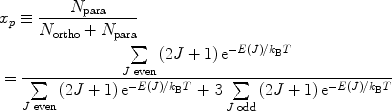
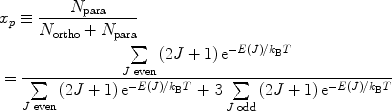
([6])
where  is the energy of the rotational state with quantum number J, k B is the Boltzmann constant, and
is the energy of the rotational state with quantum number J, k B is the Boltzmann constant, and 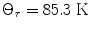 . At liquid hydrogen temperatures (≈14–20 K), we have essentially pure para-hydrogen, and at room temperature, we are in the high-temperature regime (Fig. 3). The conversion between the ortho and para states is however very slow, so for practical purposes an efficient catalyst must be used.
. At liquid hydrogen temperatures (≈14–20 K), we have essentially pure para-hydrogen, and at room temperature, we are in the high-temperature regime (Fig. 3). The conversion between the ortho and para states is however very slow, so for practical purposes an efficient catalyst must be used.

 is the energy of the rotational state with quantum number J, k B is the Boltzmann constant, and
is the energy of the rotational state with quantum number J, k B is the Boltzmann constant, and 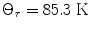 . At liquid hydrogen temperatures (≈14–20 K), we have essentially pure para-hydrogen, and at room temperature, we are in the high-temperature regime (Fig. 3). The conversion between the ortho and para states is however very slow, so for practical purposes an efficient catalyst must be used.
. At liquid hydrogen temperatures (≈14–20 K), we have essentially pure para-hydrogen, and at room temperature, we are in the high-temperature regime (Fig. 3). The conversion between the ortho and para states is however very slow, so for practical purposes an efficient catalyst must be used.
Fig. 3.
Fraction of para-hydrogen vs. temperature at thermal equilibrium.
The spin density operators for ortho- and para-hydrogen are respectively equal to
 and
and 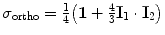 , where I 1 and I 2 are the spin operators
, where I 1 and I 2 are the spin operators 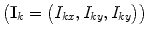 of the two protons. For a mixture of ortho- and para-hydrogen, with a molar fraction x p of para-hydrogen, the total spin density operator is
of the two protons. For a mixture of ortho- and para-hydrogen, with a molar fraction x p of para-hydrogen, the total spin density operator is  , with
, with  .
.3.2 Hydrogenation with Para-hydrogen
The basic principle behind the PHIP phenomenon is the transfer of order from the para-hydrogen proton spin state to nuclear spins of other molecules. This requires a coupling between the para-hydrogen protons and the other nuclear spins. The most common method to obtain this coupling is to hydrogenate the molecule of interest with para-hydrogen, but also the possibility of using a transient coupling, without hydrogenation, has been demonstrated (61). The hydrogenation requires a catalyst that preserves the spin correlation between the protons, i.e., the hydrogen molecules are transferred to the substrate without scrambling of protons between different para-hydrogen molecules. It has recently been demonstrated that not only homogeneous catalysts can be used but also heterogeneous ones (62, 63).
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree