Nuclear Magnetic Resonance Spectroscopy
Objectives
At the completion of this chapter, the student should be able to do the following:
Key Terms
This chapter has several goals: to explain basic nuclear magnetic resonance (NMR) spectroscopy, to get the reader excited about the use of NMR in medicine, and to show that expert treatment of NMR spectroscopy is a specialty topic. The treatment is thus illustrative, not comprehensive. The scientific literature on NMR and its medical companion magnetic resonance spectroscopy (MRS) is extensive.
Magnetic resonance imaging (MRI), the principal subject of this book, evolved from the scientific application of NMR high-resolution spectroscopy. Knowledge of NMR spectroscopy is not essential for an understanding of MRI. Rather, it is an enhancement that should be in the tool chest of any serious MRI radiologist or technologist.
This chapter deals with NMR spectroscopy in simple terms. It will give the imaging physician and technologist a brief look into the history of NMR and a similar glance at the future. It will also give the chemical NMR spectroscopist an equally interesting view of the future. One principal aim of MRS use in MRI is the performance of in vivo analysis of pathologic conditions, which increases the diagnostic value of MRI.
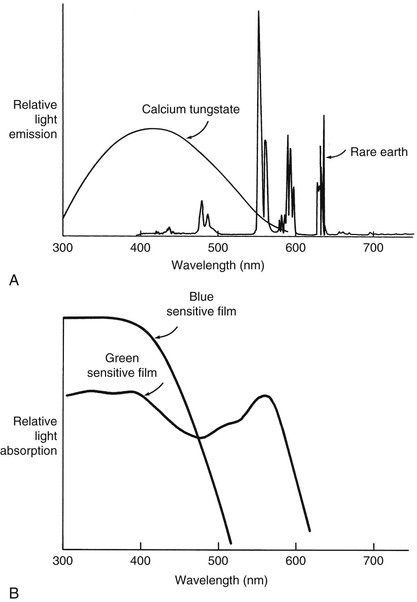
The first concept to remember is the spectrum. A spectrum, sometimes referred to as a frequency distribution, is a convenient graphic means of presenting specific frequency or wavelength-related material. Two spectra that should be familiar are those associated with the emission of light from rare-earth radiographic intensifying screens and the absorption of that light in the emulsion of radiographic film (Figure 21-1).
Absorptiometry is an analysis of the absorption of ultraviolet (UV), visual, and infrared (IR) light transmission through a sample. If the measurement is made at many different wavelengths or frequencies, the resulting graph is reported as a spectrum. For NMR spectroscopy the X-axis defines the radiofrequencies (RFs) emitted by the patient, and the Y-axis is the intensity of the signal produced by the patient at each frequency. Representative UV absorption, IR transmission, and NMR emission spectra are shown in Figure 21-2.
The line widths seen in Figure 21-2 are different in these three spectra. Those of the first two methods are “broad,” whereas the NMR signals appear “sharp.” Indeed, NMR is widely regarded as high-resolution spectroscopy.
MRS line widths can be exceedingly sharp.
An NMR spectrum can be obtained from any free induction decay (FID). The FID is the NMR signal emitted at the Larmor frequency from an excited sample. The FID may be viewed as a plot of signal intensity versus time (Figure 21-3). If the FID results from the excitation of an NMR sample by a single RF pulse, a mathematical process called the Fourier transform (FT) can be used to convert the abstract FID to a readily interpreted spectrum. The NMR spectrum is presented as signal intensity versus frequency.
Fourier transformation is used to analyze periodic (sine or cosine) wave functions mathematically. Knowledge of the operation or underlying mathematics of the FT is not required for the production of MR images or spectra. The reader can simply accept the FT as a black box that produces acceptable results from FID data sets.
Consider an analogy to poker strategies (Figure 21-4). One strategy is to make all moves on the basis of experience, the ability to keep a poker face, and a good feel for the game. Another, more technical, strategy is to factor in the role of probability in the construction of the hand and to discard and draw more cards to improve the chances of making a better hand. At the end of play, a player using the first strategy will probably admit that poker is fun but a little expensive. A more technical player may or may not enjoy the game as much but will probably have extra money.
Nuclear Species
Shortly after the discovery of NMR, one principle became well known: each nuclide in the periodic table has a unique resonance frequency at a given magnetic field strength. In the Larmor relationship the resonant frequency of any NMR signal depends only on the gyromagnetic ratio and the magnetic field strength. The value of gyromagnetic ratio varies by a factor of more than 100 among nuclides.
Each nuclide has its own gyromagnetic ratio.
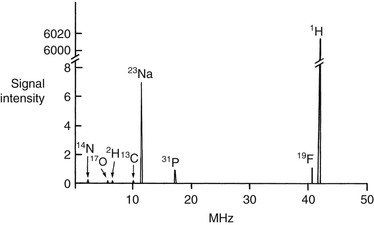
The gyromagnetic ratios of several nuclides of interest to MR in medicine are given in Table 3-1. If a total NMR spectrum of the body could be produced, it would include many nuclear species (Figure 21-5). This is a thought experiment only, so the scale is in reverse of that encountered in NMR spectroscopy.
The spacing between the signals can be understood in the following manner. The gyromagnetic ratio for hydrogen is the largest of any nuclide normally observed. The gyromagnetic ratio for 13C is about four times smaller, so the carbon resonance frequency is reduced four times. Other nuclides scale accordingly.
In any event the extremely large frequency separations between nuclides and the small range of chemical shifts for any given nuclide make it impractical to observe more than one nuclide at a time. Therefore hydrogen signals can be observed completely separately from those signals resulting from any other nuclides.
It may appear from the hypothetical spectrum in Figure 21-5 that 19F and 1H are close enough in resonance frequency to cause accidental overlap. The separation is more than 4% (megahertz apart), whereas the chemical shift ranges of each nuclide are measured in parts per million (hertz apart). There is no overlap.
Chemical Shift
Within a single nuclear species (for example, 1H), more than one peak may be present in an NMR spectrum. Pragmatically, it became obvious early in the development of NMR that the number of these peaks observed at low resolution was a measure of the number of chemically distinct hydrogen atoms in the molecule. For instance, ethanol has the chemical formula CH3CH\OH and exhibits three groups of signals in the NMR spectrum (Figure 21-6).
The pattern of differing Larmor precession frequencies is called chemical shift.
Related posts:
Stay updated, free articles. Join our Telegram channel
Full access? Get Clinical Tree
